If the point (a,a) is placed in between the lines |x+y|=4, then find the values of a.Solution in Bengali
We have lines x+y-4=0 and x+y+4=0
Point (a,a) lies on the line y=x.
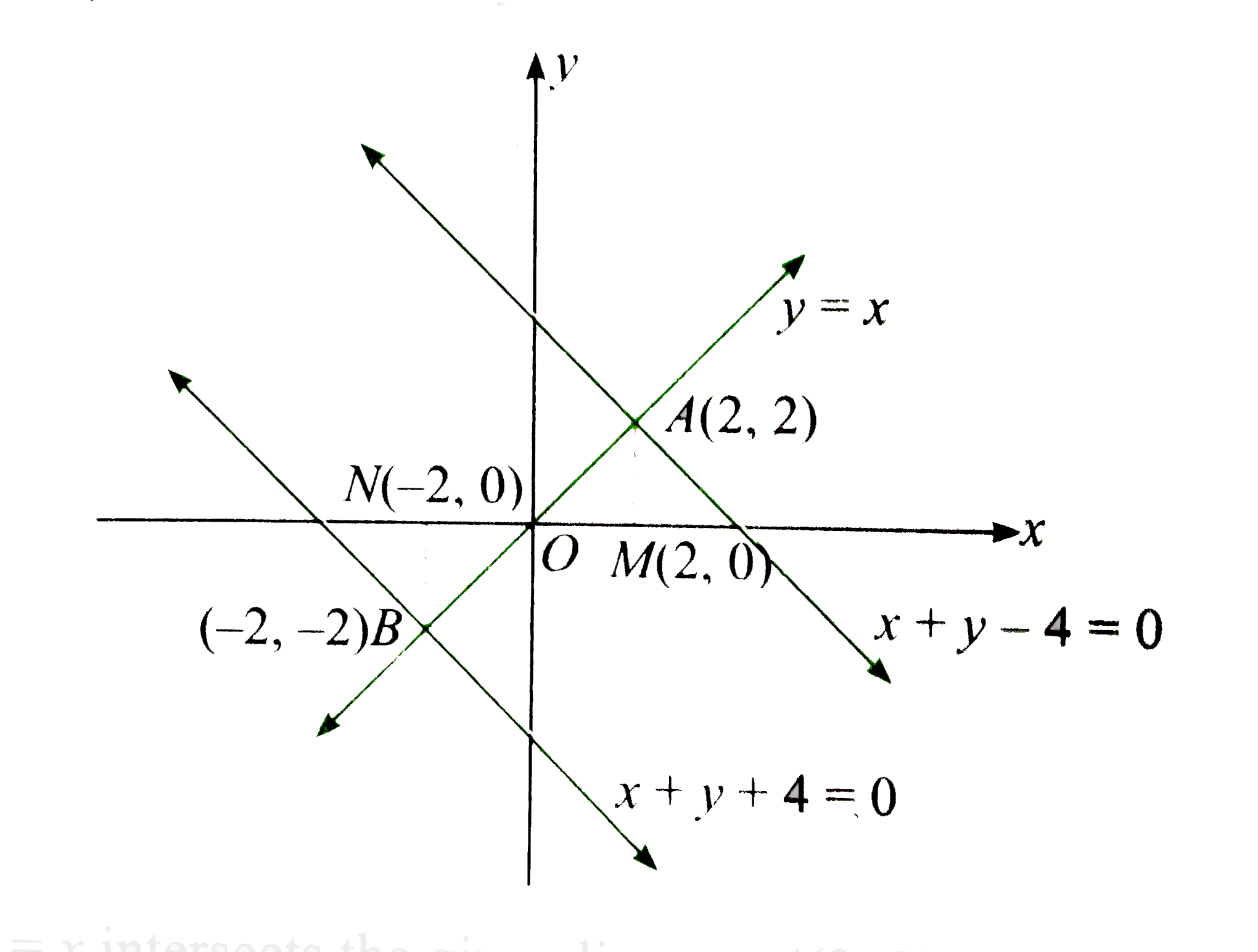
Line y=x intersects the given lines at A(2,2) and B(-2,-2).
So, if (a,a) lies on the line y=x between point A and B, we must have −2<a<2.
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXAMPLE12videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - CONCEPT APPLICATION EXERCISE 2.123videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )1videos
THEORY OF EQUATIONS
Similar Questions
Show that the lines 4x+y−9=0,x−2y+3=0,5x−y−6=0 make equal intercepts on any line of slope 2.
01:41View SolutionFind the equation of the right bisector of the line segment joining the points (3,4) and (-1,2).
04:46View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
03:24View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
09:10View SolutionThe line joining the points A(2,1),andB(3,2) is perpendicular to the line (a2)x+(a+2)y+2=0. Find the values of a.
05:30View SolutionIf the point (a,a) is placed in between the lines |x+y|=4, then find the values of a.
04:28View SolutionIf line 3x−ay−1=0 is parallel to the line (a+2)x−y+3=0 then find the value of a.
03:57View SolutionIf the point (a,a) is placed in between the lines |x+y|=4, then find the values of a.
03:29View SolutionIf the point P(a2,a) lies in the region corresponding to the acute angle between the lines 2y=x and 4y=x , then find the values of a.
06:25View SolutionIf the point (a2,a+1) lies in the angle between the lines 3x−y+1=0 and x+2y−5=0 containing the origin, then find the value of a.
01:27View SolutionIf the point (a2,a+1) lies in the angle between the lines 3x−y+1=0 and x+2y−5=0 containing the origin, then find the value of a.
05:20View SolutionIf the point (a,a) is placed in between the lines |x+y|=4, then find the values of a.
04:00View SolutionIf the point P(a2,a) lies in the region corresponding to the acute angle between the lines 2y=x and 4y=x , then find the values of a.
02:52View SolutionIf the line x+y=a touches the parabola y=x−x2, then find the value of a.
03:06View SolutionIf the point (a,a) is placed in between the lines |x+y|=4, then sum of integral values of a is
02:51View SolutionThe line joining the points A(2,1),andB(3,2) is perpendicular to the line (a2)x+(a+2)y+2=0. Find the values of a.
02:45View Solution

