The centroid of an equilateral triangle is (0,0). If two vertices of the triangle lie on x+y $= 2 \sqrt{2}$ , then one of them will have its coordinates Solution in Bengali

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $(- 2 \sqrt{2}, - 2 \sqrt{2}), (\sqrt{2} - \sqrt{6}, \sqrt{2} + \sqrt{6}), (\sqrt{2} + \sqrt{6}, \sqrt{2} - \sqrt{6})$

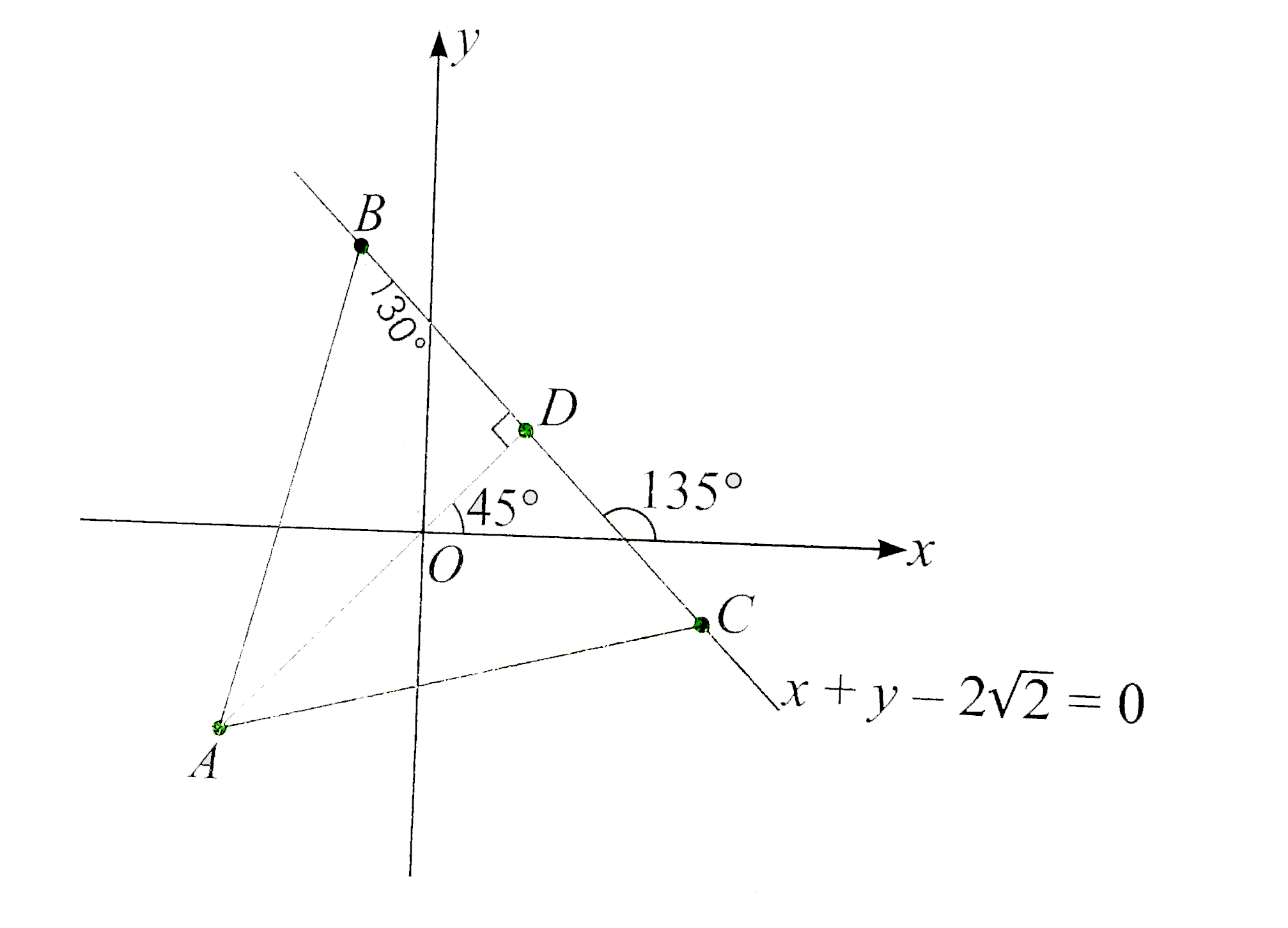
As shown in the figure, equilateral triangle ABC is such that B and C lie on the given line $x + y - 2 \sqrt{2} = 0$ .
AD is perpendicular to BC, which passes through centroid.
$O D = \frac{∣ ∣ 0 + 0 - 2 \sqrt{2} ∣ ∣}{\sqrt{2}} = 2$
$∴ OA=4 (As centroid divides median in the ratio 2 : 1)$
Slope of AD is 1, i.e., tan $45^{\circ}, where 45^{\circ}$ is the inclination of AD with x-axis.
$∴ A \equiv (0 - 4 cos 45^{\circ}, 0 - sin 45^{\circ}) \equiv (- 2 \sqrt{2}, - 2 \sqrt{2})$
$and D \equiv (0 + 2 cos 45^{\circ}, 0 + 2 sin 45^{\circ}) \equiv (\sqrt{2}, \sqrt{2})$
$Now, D C = D B = 2 cot 30^{\circ} = 2 \sqrt{3}$
Therefore, B $\equiv (\sqrt{2} + 2 \sqrt{3} cos 135^{\circ}, \sqrt{2} + 2 \sqrt{3} cos 135^{\circ})$
$\equiv (\sqrt{2} - \sqrt{6}, \sqrt{2} + \sqrt{6})$
$And C \equiv (\sqrt{2} - 2 \sqrt{3} cos 135^{\circ}, \sqrt{2} - 2 \sqrt{3} cos 135^{\circ})$
$\equiv (\sqrt{2} + \sqrt{6}, \sqrt{2} - \sqrt{6})$

Updated on:21/07/2023

|

Class 12 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

CENGAGE PUBLICATION-STRAIGHT LINES-CONCEPT APPLICATION EXERCISE 2.2

Home
Ask Doubt
Profile