A triangle is formed by the lines whose equations are AB: x+y-5=0, BC: x+7y-7=0 and CA: 7x+y+14=0.
Then

A

angle at A is acute

B

angle at C is acute

C

internal angle bisector at angle B is 3x+6y-16=0

D

external angle bisector at angle C is 8x+8y+7 = 0

Text Solution
Verified by Experts

The correct Answer is:A,C,D

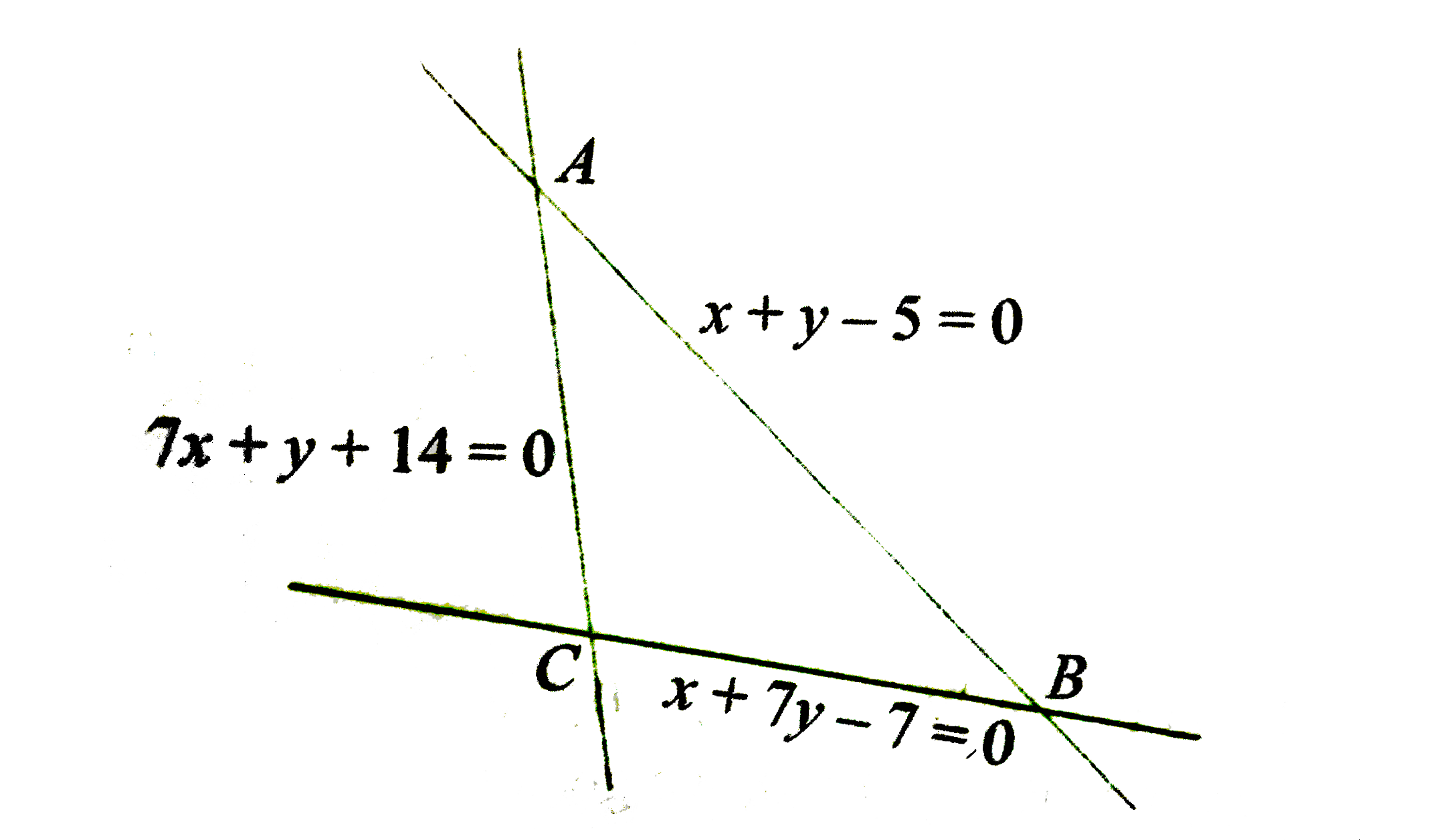
ocr_image
The slopes of the line AB, BC and CA are -1, −17 and -7, respectively,
Let m1=−17,m2=−1,m3=−7.
∴m1>m2>m3
So, tangent fo internal angles of the triangle are
tanA=34,tanB=34 and tanC=−247
So, interior angles A and B are acute and interior angle C is abtuse.
∴ Internal bisector of B ≡ Acute angle bisector at B

≡ Acute angle bisector of lines AB and BC
≡3x+6y−16=0
External bisector of C ≡ Acute bisector of C
≡ Acute angle bisector of lines AC and BC
≡8x+8y+7=0

Updated on:21/07/2023
Doubtnut Promotions Banner
|
Class 12MATHSSTRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Find the area of the triangle formed by the straight lines whose equations are 2y+x−5=0,y+2x−7=0, and x−y+1=0.

    A310
    B10
    C6
    D25

  • Orthocentre of the triangle formed by the lines whose combined equation is (y2−6xy−x2)(4x−y+7)=0 is

    A(1,2)
    B(2,3)
    C(0,1)
    Dnone of these