Question

Given points $P(2,3),Q(4,-2),\text{and}R(\alpha ,0)$. Find the value of $\alpha$ if $PR+RQ$ is minimum.

Explanation

(i) Point `R(alpha,0)` lies on x-axis.

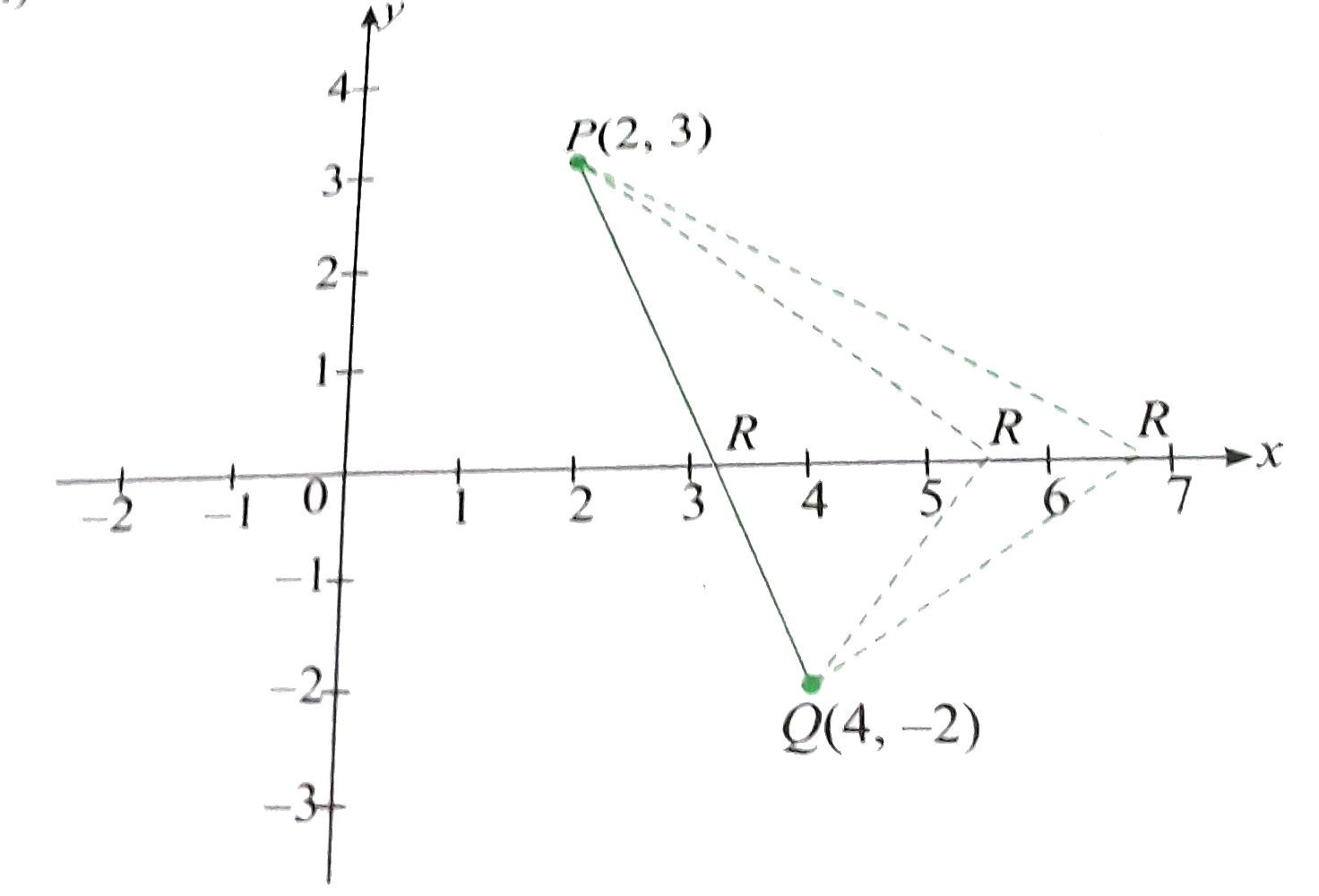
Now, from triangular inequality, `PR+RQgePQ`
Thus minimum value of `PR+RQ` is PQ, which occurs when points `P,Q` and R are collinear.
`rArr|{:(2,,3,,),(4,,-2,,),(alpha,,0,,),(2,,3,,):}|=0`
`rArr-4-12+2alpha+3alpha=0`
`rArralpha=16//5`
(ii)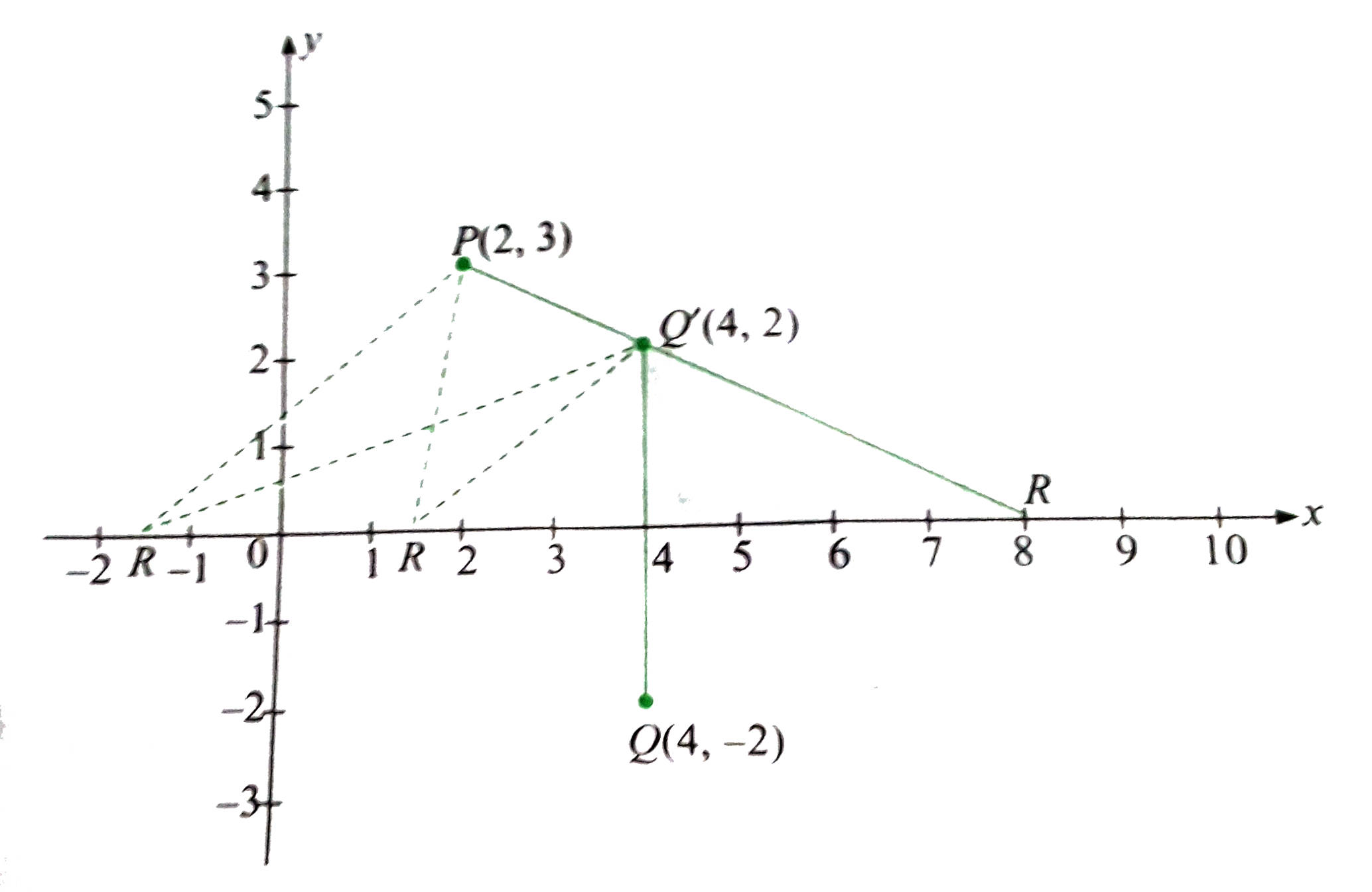
Image of Q in x-axis is `Q'(4,2)`.
Now, `RQ=RQ`'
`therefore |PR-RQ|=|PR-RQ'|`
From triangle inequality,
` |PR-PQ'|le PQ`'.
`therefore |PR-PQ'|_(max)=PQ'`, when `P,Q`' and R are collinear
`therefore|{:(2,,3,,),(4,,2,,),(alpha,,0,,),(2,,3,,):}|=0`
`rArr4-12-2alpha+3alpha=0`
`rArralpha=8`