Let ABCD be a rectangle and P be any point in its plane. Show that AP2+PC2=PB2+PD2.Solution in Bengali
The correct Answer is:NA
Let A≡(0,0),B≡(a,0),C≡(a,b)andD≡(0,b)
Let point P be (h,k).
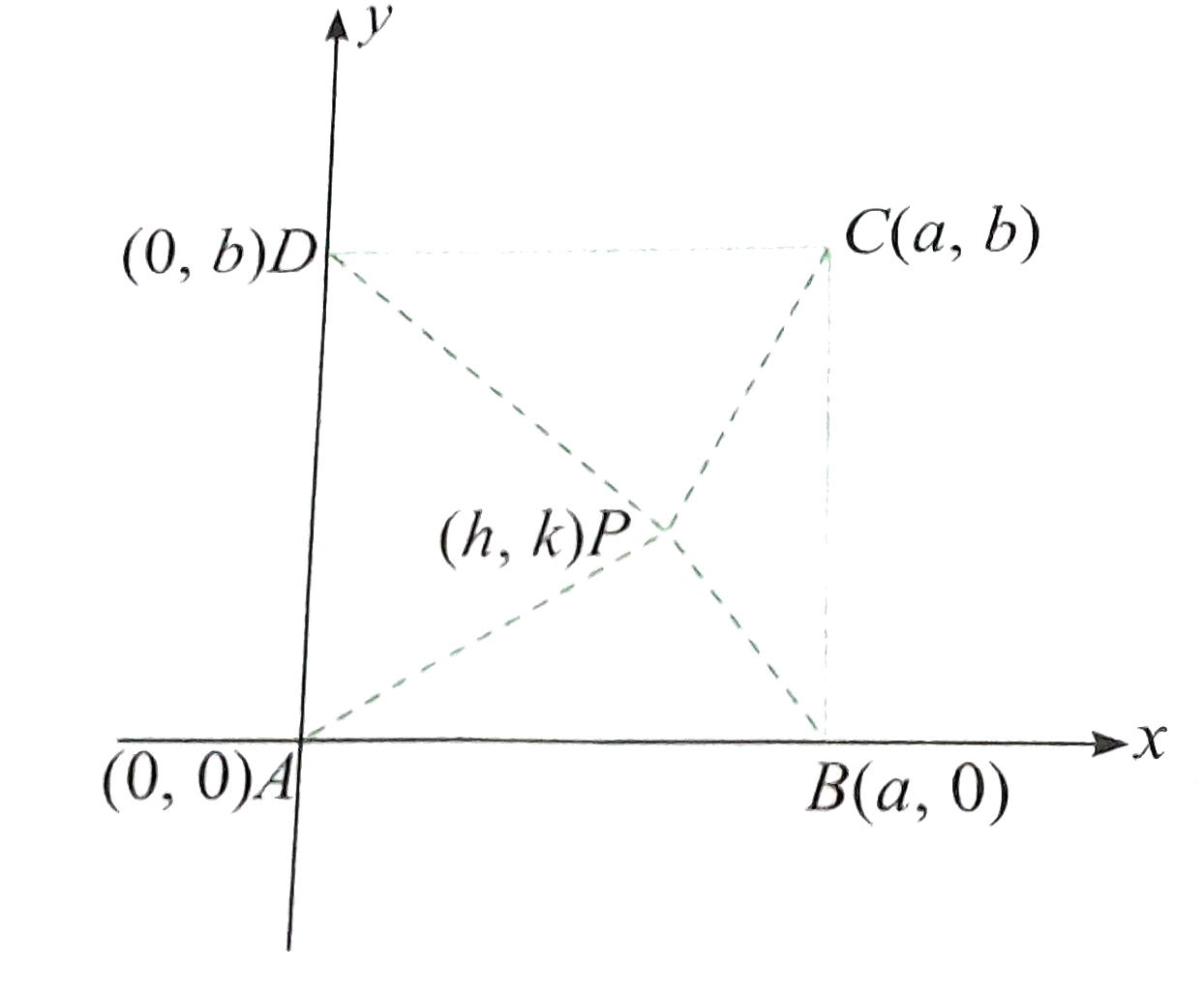
∴PA2+PC2=(h−0)2+(k−0)2+(h−a)2+(k−b)2=2h2+2k2−2k2−2ah−2bk+a2+b2
Also, PB2+PD2=(h−a)2+(k−0)2+(h−0)2+(k−b)2=2h2+2k2−2ah−2bk+a2+b2
Thus, PA2+PC2=PB2+PD2
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.310videos
COORDINATE SYSYEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.48videos
COORDINATE SYSYEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.16videos
COORDINATE SYSYEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type2videos
COORDINATE SYSTEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.213videos
CROSS PRODUCTS
Similar Questions
If point P(3,2) divides the line segment AB internally in the ratio of 3:2 and point Q(−2,3) divides AB externally in the ratio 4:3 then find the coordinates of points A and B.
05:16View SolutionThe line joining the points (x,2x)and(3,5) makes an obtuse angle with the positive direction of the x-axis. Then find the values of x.
08:53View SolutionWhat is the minimum area of a triangle with integral vertices ?
07:37View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
04:01View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
04:36View SolutionIn Figure, P is a point in the interior of a parallelogram ABCD . Show that ar(APB)+ar(PCD)=12ar(^(gm)ABCD) aR(APD)+ar(PBC)=ar(APB)+ar(PCD)
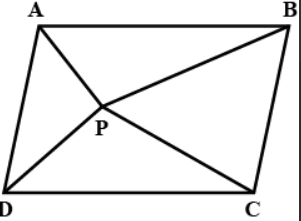 03:44View Solution
03:44View SolutionIn Figure, ABCD and PQRC are rectangles and Q is the mid-point of AC . Prove that DP=PC (ii) PR=12 AC
04:52View SolutionIn Figure, P is a point in the interior of a parallelogram ABCD. Show that ar(APB)+ar(PCD)=12ar (||gmABCD) ar (APD)+ar (PBC)=ar (APB)+ar(PCD)
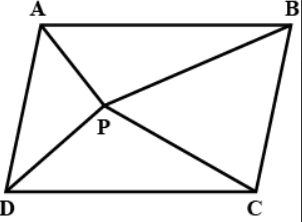 11:49View Solution
11:49View SolutionIf a, b, c, d and p are different real numbers such that (a2+b2+c2)p2−2(ab+bc+cd)p+(b2+c2+d2)≤0, then show that a, b, c and d are in G.P.
09:21View SolutionLet ABCD be a rectangle and P be any point in its plane. Show that AP2+PC2=PB2+PD2.
06:02View SolutionLet ABCD be a rectangle and P be any point in its plane. Show that AP2+PC2=PB2+PD2.
03:41View SolutionIf P is a point and ABCD is a quadrilateral and →AP+→PB+→PD=→PC , show that ABCD is a parallelogram.
04:19View SolutionABCD are four points in a plane and Q is the point of intersection of the lines joining the mid-points of AB and CD;BC and AD. Show that →PA+→PB+→PC+→PD=4→PQ, where P is any point.
09:19View SolutionIn Figure, P is a point in the interior of a parallelogram ABCD . Show that ar(APB)+ar(PCD)=12ar(^(gm)ABCD) aR(APD)+ar(PBC)=ar(APB)+ar(PCD)
05:15View SolutionIn a right triangle ABC right-angled at B, if PandQ are points on the sides ABandAC respectively, then (a)AQ2+CP2=2(AC2+PQ2) (b)2(AQ2+CP2)=AC2+PQ2 (c)AQ2+CP2=AC2+PQ2 (d)AQ+CP=12(AC+PQ).
03:45View SolutionA(4, 2), B(6,5) and C(1, 4) are the vertices of ABC . Find the coordinates of point P on AD such that AP:PD=2:1 .
05:32View Solution

