Find the length of altitude through A of the triangle ABC, where A≡(−3,0)B≡(4,−1),C≡(5,2)Solution in Bengali
The correct Answer is:22√10
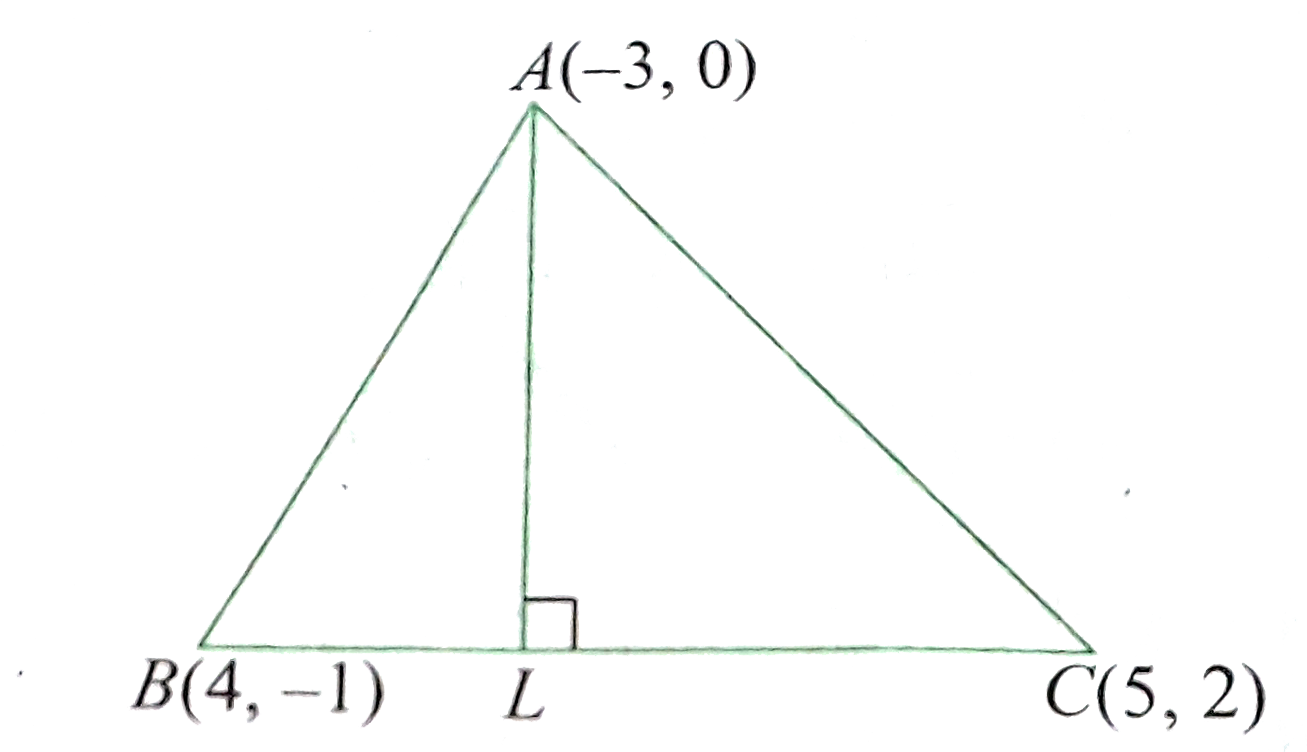
In the figure , AL is altitude .
Area of triangle =12[−3(−1−2)+4(2−0)+5(0+1)]=11
Also, BC=√(5−4)2+(2+1)2=√1+9=√10
Now, area of triangle =12×AL×BC
∴AL=2×11√10=22√10 .
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.310videos
COORDINATE SYSYEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.48videos
COORDINATE SYSYEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.16videos
COORDINATE SYSYEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type2videos
COORDINATE SYSTEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.213videos
CROSS PRODUCTS
Similar Questions
If point P(3,2) divides the line segment AB internally in the ratio of 3:2 and point Q(−2,3) divides AB externally in the ratio 4:3 then find the coordinates of points A and B.
02:12View SolutionThe line joining the points (x,2x)and(3,5) makes an obtuse angle with the positive direction of the x-axis. Then find the values of x.
02:58View SolutionWhat is the minimum area of a triangle with integral vertices ?
04:23View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
08:23View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
03:03View SolutionFind the length of altitude through A of the triangle ABC, where A≡(−3,0)B≡(4,−1),C≡(5,2)
04:09View SolutionIn a triangle ABC with vertices A(2,3),B(4,−1)andC(1,2) . Find the length of the altitude from the vertex A .
View Solutionत्रिभुज ABC के शीर्ष A से डाले जाने वाले शीर्ष-लंब की लम्बाई जहाॅं A=(−3,0),B(4,−1) तथा C = (5 , 2) है, हैं
05:19View SolutionIf A(-3,0),B(4,-1),C(5,2) are vertices of ΔABC then the length of altitude through A is
03:06View SolutionIf A(-3,0),B(4,-1),C(5,2) are vertices of △ ABC, then the length of altitude through A is
03:42View SolutionThe length of altitude through A of the triangle ABC where A≡(−3,0),B≡(4,−1)C≡(5,2) is
03:52View SolutionThe length of the length to be subtended from the vertex A of the triangle ABC, where A = (-3,0), B = (4, -1) and C = (5,2).
03:48View SolutionFind the length of altitude through A of the triangle ABC, where A≡(−3,0)B≡(4,−1),C≡(5,2)
06:28View SolutionConsider a triangle with vertices A(1,2),B(3,1) and C(−3,0). Find the equation of altitude through vertex A.
04:44View SolutionFind the length of altitude through A of the triangle ABC, where A≡(−3,0)B≡(4,−1),C≡(5,2)
07:56View SolutionThe vertices of ABC are (−2, 1), (5, 4) and (2, −3) respectively. Find the area of the triangle and the length of the altitude through A .
04:38View Solution

