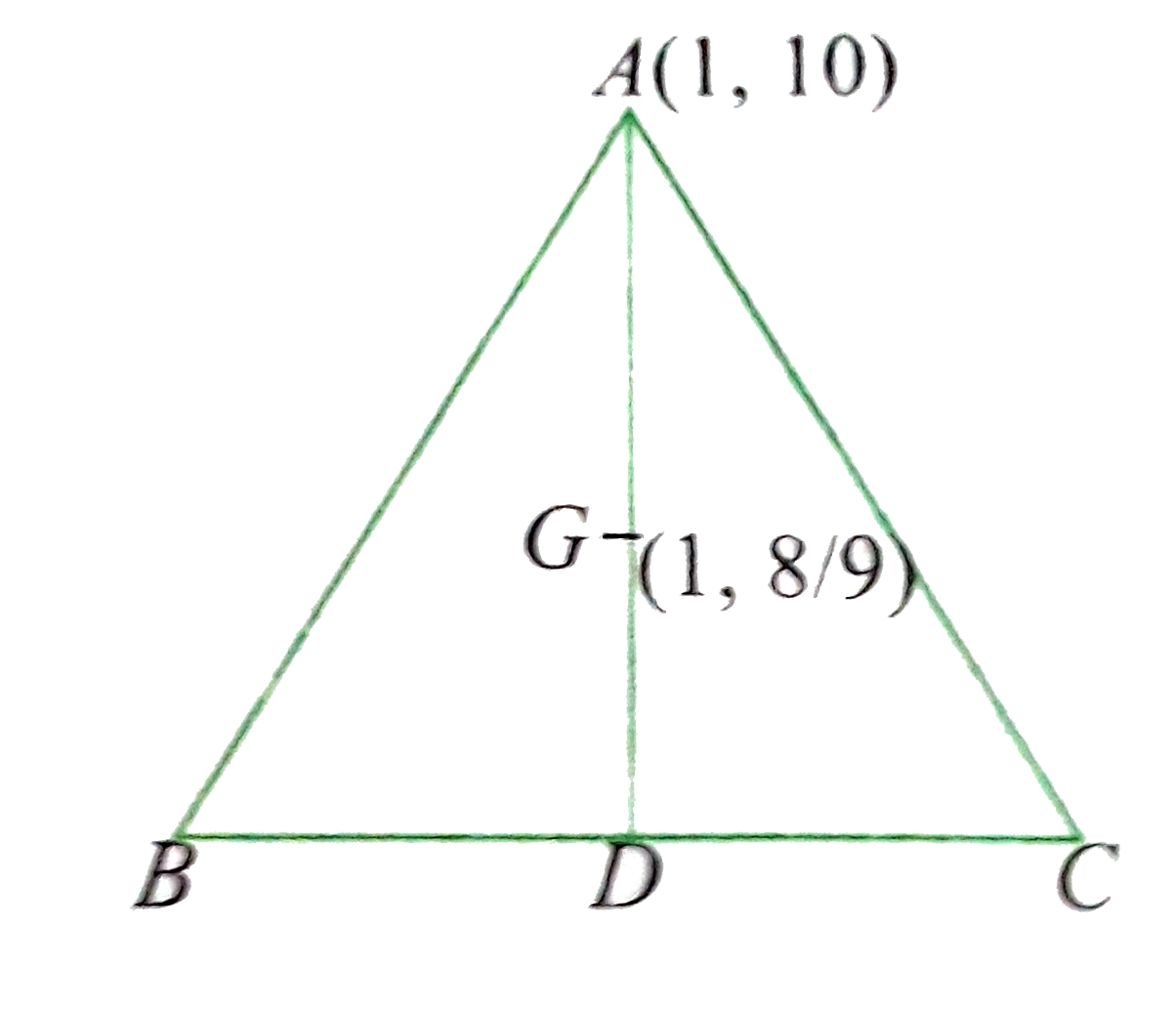
If in triangle ABC, A=(1, 10), circumcentre=(−13,23) and orthocentre=(113,43) then the co-ordinates of mid-point of side opposite to A is:Solution in Bengali
(1,−11/3)
(1/5)
(1,−3)
(1,6)
The correct Answer is:A
Circumcenter O≡(−1/3,2/3) and orthocenter H≡(11/3,4/3)
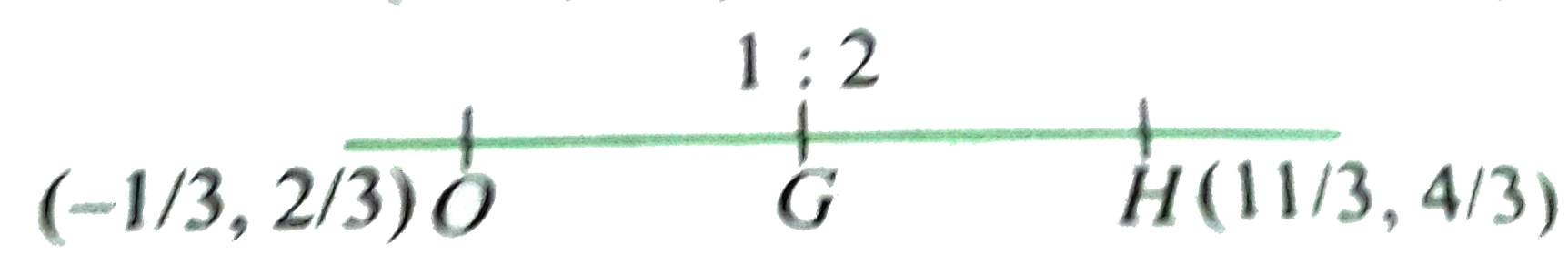
Therefore the coordinates of G are (1,8/9). Now,the point A is (1,10) as G is (1,8/9).
Also, AD:DG=3:1,
∴D≡(3−13−1,8/3−103−1)≡(1,−11/3)
∴D=3−12=1,Dy=8/3−102=−113
Hence, the coordinates of the midpoint of BC are (1,−11/3) .
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Multiple correct13videos
COORDINATE SYSYEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Linked10videos
COORDINATE SYSYEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.69videos
COORDINATE SYSYEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type2videos
COORDINATE SYSTEM
- BOOK - CENGAGE PUBLICATIONCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.213videos
CROSS PRODUCTS
Similar Questions
If (-6,-4), (3,5),(-2,1) are the vertices of a parallelogram, then remaining vertex cannot be
08:27View SolutionFor points P=(x1,y1)and Q=(x2,y2) of the co-ordinate plane a new distance d(P,Q)=|x1−x2|+|y1−y2| is defined .Let O(0,0)and A(3,2). The set of points in first quadrant which are equidistant from O and A is
02:10View SolutionFind the locus of a point whose distance from (a, 0) is equal to its distance from the y-axis.
03:04View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
07:34View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
View Solution(1)If in triangle ABC,A=(1,10) circumcentre (-(1)/(3),(2)/(3)) and orthocentre ((11)/(3),(4)/(3)) then the co-ordinates of mid-point of side opposite to A is (2)A line BC,CA and AB of the triangle ABC in P(x_(1),y_(1)),Q(x_(2)y_(2)),R(x_(3),y_(3)) respectively, then:
07:55View SolutionIf a triangle ABC,A≡(1,10), circumcenter ≡(−13,23), and orthocentre ≡(114,43) , then the coordinates of the midpoint of the side opposite to A are (1,−113) (b) (1,5) (1,−3) (d) (1,6)
02:51View SolutionIf in triangle ABC,A≡(1,10), circumcentre ≡(−13,23) and orthocentre ≡(113,43) then the co-ordinates of mid-point of side opposite to A is (1,−113) (b) (1, 5 ) (c) (1,−3) (d) (1, 6)
03:24View SolutionIf (5.5, 6), (1.5, 3) and (1, 5) are the co-ordinates of the mid points of 3 sides of a triangle, then find the co-ordinates of vertices of △ABC.
02:14View SolutionConsider a triangle ABC in xy-plane with D, E and F as the middle points of the sides, BC, CA and AB respectively. If the co-ordinates of the point D,E,F are (32,32),(72,0)and(0,−12) then:
STATEMENT-1: Circumcentre of triangle ABC lies inside the triangle.
STATEMENT-2: Orthocentre, centroid, circumcentre and incentre of triangle DEF are collinear but of triangle ABC are not-collinear.
STATEMENT-3: Distance between centrioid and ortocente of ΔABCis5√23.09:17View SolutionFind the co ordinates of circumcentre and radius of a circumcircle of ΔABC, if A(7, 1) , B (3, 5) and C(2,0) are given.
08:11View SolutionIf (0, 1) is the orthocentre and (2, 3) is the centroid of a triangle. Then its circumcentre is
04:02View SolutionIf in a ΔABC,B is orthocentre and if circumcentre of ΔABC is (2,4) and vertex A is (0,0) then co-ordinates of vertex 'C' is
04:13View SolutionIf in a ΔABC,B is orthocentre and if circumcentre of ΔABC is (2,4) and vertex A is (0,0) then co-ordinates of vertex C is
04:20View SolutionThe distance between the circumcentre and the orthocentre of the triangle formed by (1,2,3),(3,-1,5),(4,0,-3) is
03:12View SolutionThe vertices of a triangle are (4,−3), (−2,1) and (2,3) . Find the co-ordinates of the circumcentre of the triangle.
[Circumcentre is the point of concurrence of the right-bisectors of the sides of a triangle]05:23View Solution

