Find the area enclosed by $y = g (x),$ x-axis, x=1 and x=37, where g(x) is inverse of $f (x) = x^{3} + 3 x + 1$ .Solution in Bengali

Video Solution

Text Solution

Verified by Experts

$Required area, A = \overset{37}{\int_{1}} g (x) d x = \overset{37}{\int_{1}} f^{- 1} (x) d x .$
$Let f^{- 1} (x) = t or x = f (t)$
Using intelligent gusessing, $f (3) = 37 and f (0) = 1$
$∴ A = \overset{3}{\int_{0}} t f' (t) d t = {[t f (t)]}_{0}^{3} - \overset{3}{\int_{0}} f (t) d t$
$= 3 f (3) - \overset{3}{\int_{0}} (t^{3} + 3 t + 1) d t$
$= 111 - \frac{147}{4} = \frac{297}{4}$
Alternative method :
$f (x) = x^{3} + 3 x + 1 .$
$∴ f' (x) = 3 x^{2} + 3 > 0, \forall \in R .$
$∴ f (x)$ is an increasing function.
Also, $x^{3} + 3 x + 1 = x or x^{3} + 2 x + 1 = 0$ has no positive root.
So, line y=x never meet curve $y = f (x) for x > 0$ .
Graph of $y = f (x) and y = f^{- 1} (x)$ are as shown in the following figure.
$When y = 1, x^{3} + 3 x + 1 = 1, x = 0 .$
$When y = 37, x^{3} + 3 x = 36, x = 3$

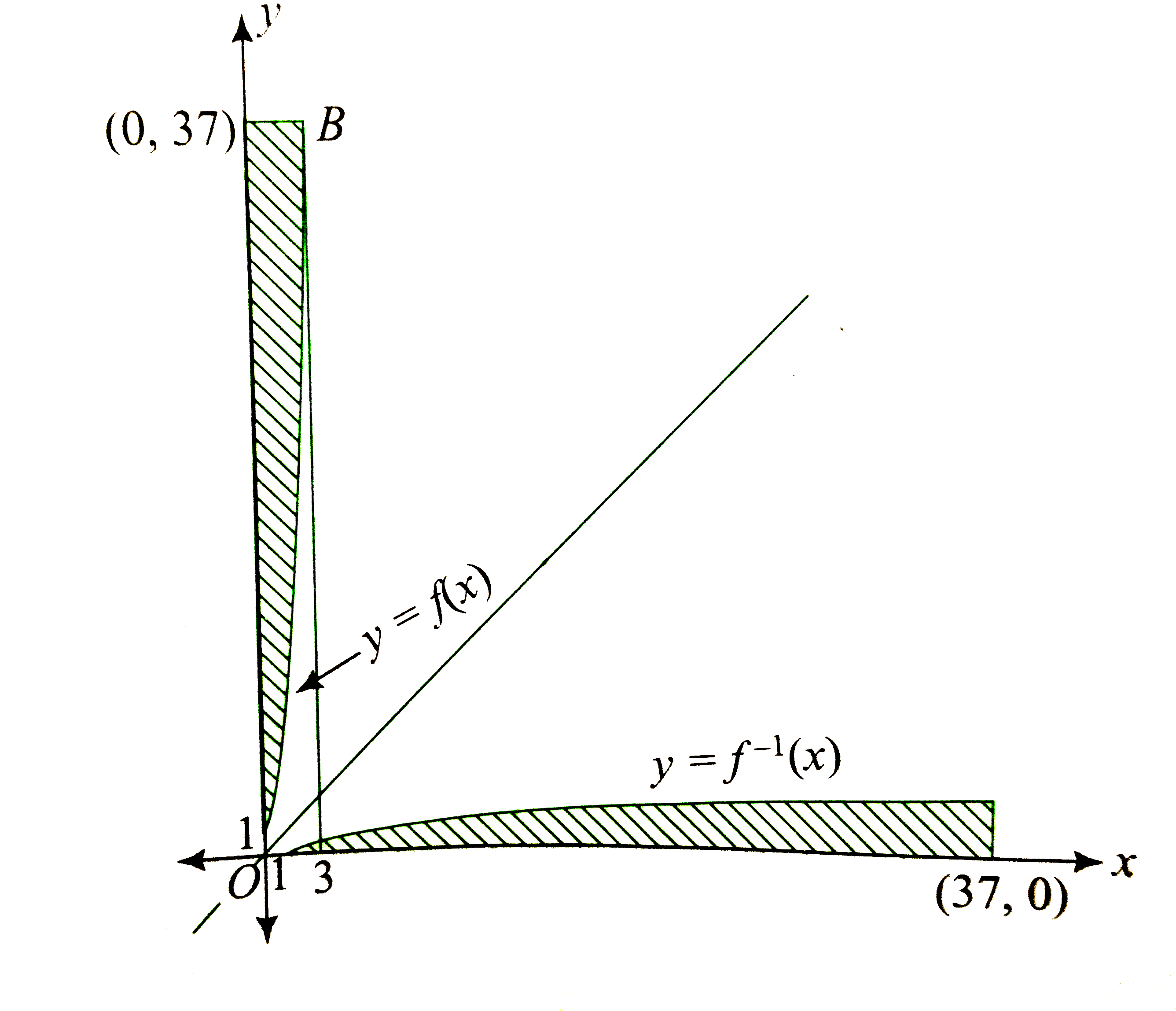
Area bounded by curves $y = f^{- 1} (x),$ x-axis, x=1 and x=37 is same as area bounded by curves $y = f (x), y$ -axis, $y = 1 and y = 37$ .
$∴ Required area = \overset{3}{\int_{0}} (37 - x^{3} - 3 x - 1) d x$
$= {[36 x - \frac{x^{4}}{4} - \frac{3 x^{2}}{2}]}_{0}^{3} = \frac{297}{4}$ sq. units.

|

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

CENGAGE PUBLICATION-AREA-Comprehension Type

Home
Ask Doubt
Profile