Find the area bounded by y=x2andy=x1/3 for x∈[−1,1].Solution in Bengali
The correct Answer is:32 sq. units
Solving given curves we get x2=x1/3
∴ x=0orx=1
Required area, A=0∫−1[x2−x1/3]dx+1∫0[x1/3−x2]dx
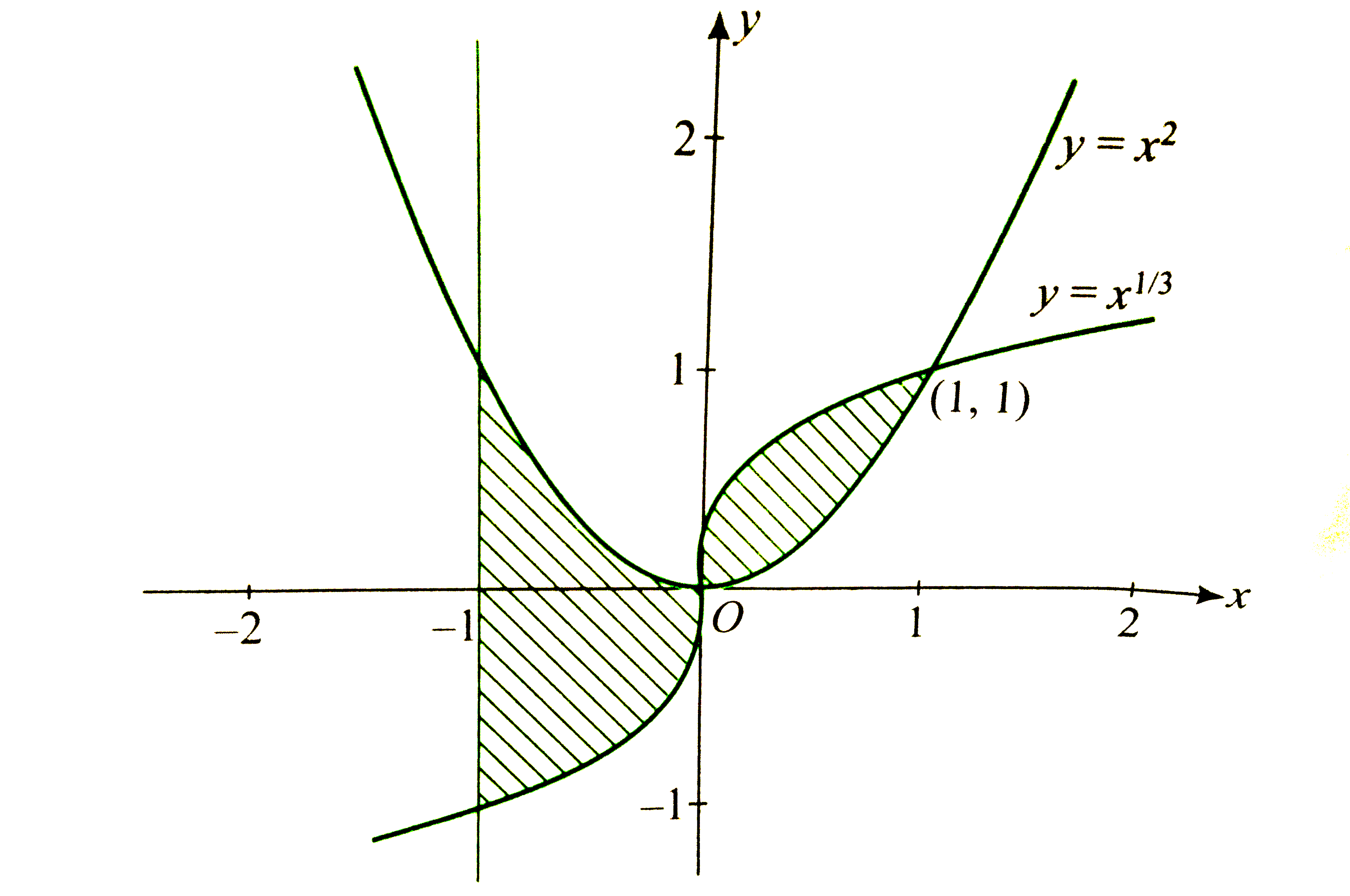
=[x33−3x4/34]0−1+[3x4/34−x33]10
=−[−13−34]+[34−13]=32 sq. units.
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Concept Application Exercise 9.37videos
AREA
- BOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Exercises - Single Correct Answer Type40videos
AREA
- BOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Concept Application Exercise 9.19videos
AREA
- BOOK - CENGAGE PUBLICATIONCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Subjective Type2videos
APPLICATIONS OF DERIVATIVES
- BOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension11videos
BINOMIAL THEOREM
Similar Questions
Find the continuous function f where (x4−4x2)≤f(x)≤(2x2−x3) such that the area bounded by y=f(x),y=x4−4x2. then y-axis, and the line x=t, where (0≤t≤2) is k times the area bounded by y=f(x),y=2x2−x3,y-axis , and line x=t(where0≤t≤2).
10:13View SolutionArea enclosed by the curve y=f(x) defined parametrically as x=1−t21+t2,y=2t1+t2isequa<o πsq.units (b) π2sq.units 3π4sq.units (d) 3π2sq.units
03:25View SolutionFind the area of the smaller part of the circle x2+y2=a2 cut off by the line x=a√2.
07:14View SolutionProve that for λ>1, the equation xlogx+x=λ has least one solution in [1,λ].
02:36View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is04:41View SolutionArea bounded by the curves y=x^(2)-1,x+y=3 is
07:46View SolutionFind the area of the region bounded by y=−1,y=2,x=y3andx=0.
05:01View SolutionArea bounded by y=x^(3)-x and y=x^(2)+x is
05:01View SolutionFind the area bounded by the curves y=√1−x2 and y=x3−x without using integration.
03:12View SolutionFind the area bounded by y=x2andy=x1/3 for x∈[−1,1].
02:26View Solutionसमाकलन का प्रयोग कर y−1=x, x-अक्ष तथा कोटियों x=−2 और x=3 से घिरे क्षेत्र का क्षेत्रफल निकालें ।
01:56View Solutionवक्रों y=−1,y=2,x=y3 और x = 0 से घिरे क्षेत्र का क्षेत्रफल ज्ञात कीजिए।
07:14View SolutionThe area of the region bounded by y = x - 1 and x=3−y2 is
03:03View SolutionFind the area bounded by the curves y=√1−x2 and y=x3−x without using integration.
06:05View SolutionFind the area bounded by y=x2andy=x1/3 for x∈[−1,1].
10:05View SolutionFind the area of the region bounded by
y=x2andy=x306:29View Solution
