For which of the following values of m is the area of the regions bounded by the curve y=x−x2 and the line y=mx equal 92? −4 (b) −2 (c) 2 (d) 4Solution in Bengali
2e∫1√logeydy
2e−1∫−1ex2dx
1∫−1(e−ex2)dx
21∫0√xexdx
The correct Answer is:A, B, C, D
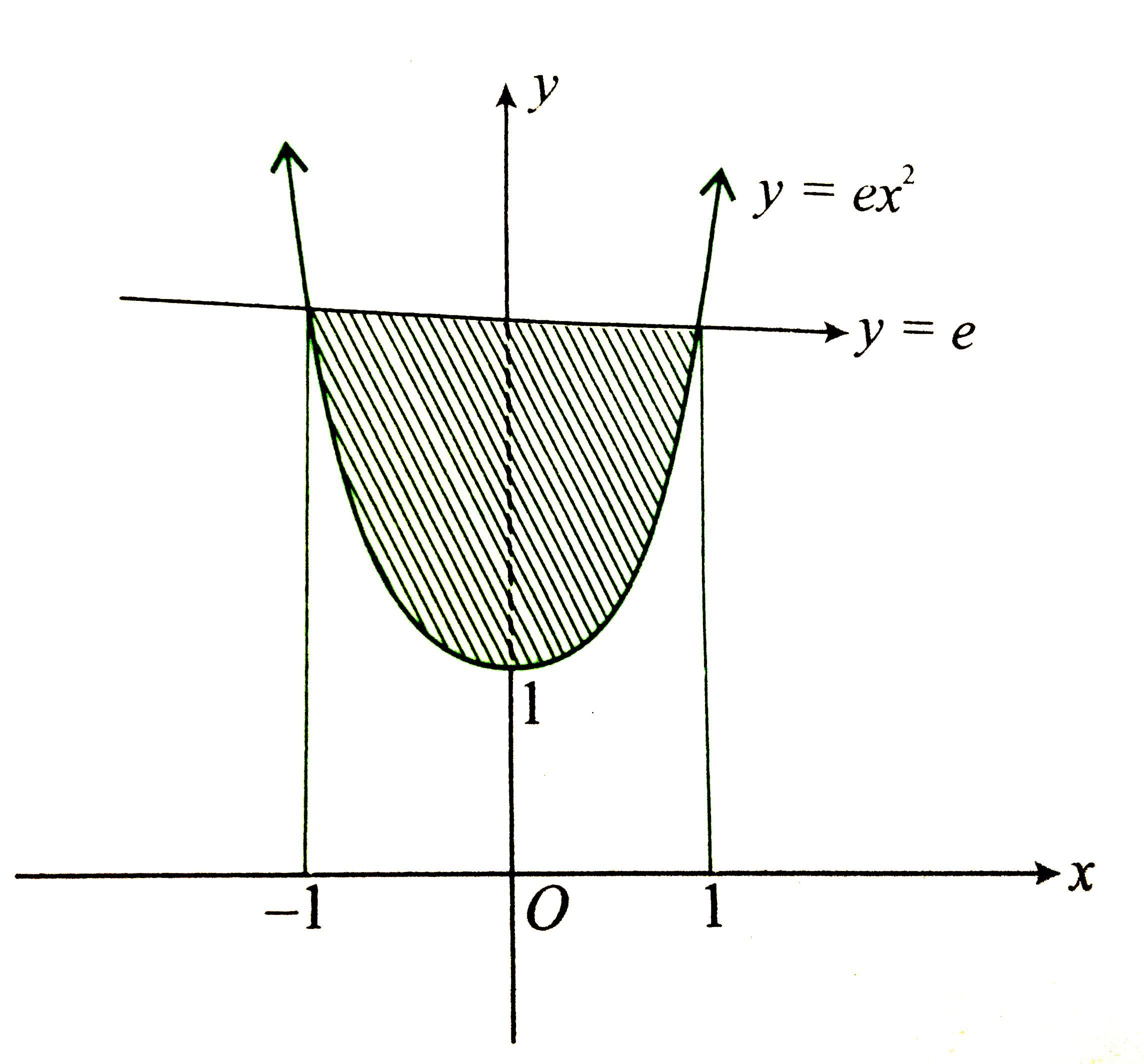
Area, A=1∫−1(e−ex2)dx=2e−1∫−1ex2dx
y=ex2
∴ logey=x2
∴ x=±√logey
∴ Area, A=2e∫1√logeydy
Now put logey=t.
∴ y=et
∴ dy=etdt
So, area A=21∫0√t etdt
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Linkded Comprehension Type21videos
AREA
- BOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Matrix Match Type5videos
AREA
- BOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Exercises - Single Correct Answer Type40videos
AREA
- BOOK - CENGAGE PUBLICATIONCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Subjective Type2videos
APPLICATIONS OF DERIVATIVES
- BOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension11videos
BINOMIAL THEOREM
Similar Questions
Let Ar be the area of the region bounded between the curves y2=(e−kr)x(where k>0,r∈N) and the line y=mx(where m≠0), k and m are some constants
A1,A2,A3,… are in G.P. with common ratio04:56View SolutionMatch the following lists :
 08:07View Solution
08:07View SolutionArea enclosed by the curve y=f(x) defined parametrically as x=1−t21+t2,y=2t1+t2isequa<o πsq.units (b) π2sq.units 3π4sq.units (d) 3π2sq.units
08:04View SolutionProve that for λ>1, the equation xlogx+x=λ has least one solution in [1,λ].
09:23View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is01:44View SolutionFor which of the following values of m is the area of the regions bounded by the curve y=x−x2 and the line y=mx equal 92? (a) −4 (b) −2 (c) 2 (d) 4
03:04View SolutionIf the area bounded by the curves y=x-x^(2) and line y=mx is equal to (9)/(2) sq.units,then m may be
04:24View SolutionArea of the region bounded by the curve y=x^(2),y=|2-x^(2)| and y=2, which liesto the right of the line x=1, is equal to
04:38View SolutionFind the area of the region bounded by the curves x2+y2=4 and (x−2)2+y2=4.
View SolutionThe area of the region bounded by the curve y=x2 and y = x is equal to
03:40View SolutionThe area of the region bounded by the curve y=x2 and y =|x| is equal to
04:47View SolutionThe area of the region bounded by the curves {(x,y):x2+y2≤4,2≤x+y} is __________ .
08:27View SolutionArea of the region bounded by the curve
y={x2,x<0x,x≥0 and the line y=4 is04:47View SolutionFor which of the following values of m, is the area of the region bounded by the curve y=x−x2 and the line y = mx equals 92 ?
04:33View SolutionFor which of the following values of m is the area of the regions bounded by the curve y=x−x2 and the line y=mx equal 92? (a) −4 (b) −2 (c) 2 (d) 4
05:17View SolutionWhich values of m for the following y=x-x^2 And the area bounded by the line y = mx is equal to 9/2.
14:28View Solution

