Draw the graph of f(x)=x2e−|x|
i) Find the point of maxima/minima.
ii) Find the asymptote is any.
iii) Find the range of the function.
iv) Find the number of roots of the equation f(x)=1Solution in Bengali
we have f(x)=x2e−|x|
Clearly, f(x)=f(−x).
So f(x) is an even function.
Hence the graph is symmetrical about the y-axis.
For x≥0,f(x)=x2e−x
Now f′(x)=−x2e−x+2xe−x=xe−x(2−x)
f′(x)=−x2e−x+2xe−x=xe−x(2−x)
f′(x)=0⇒x=0 or x=2
f(0)=0 and limx→∞x2ex=limx→∞2xex=2x→∞ex=0
So x=2 is the pont of maxima.
Hence the graph of f(x) is as shown in the following figure.
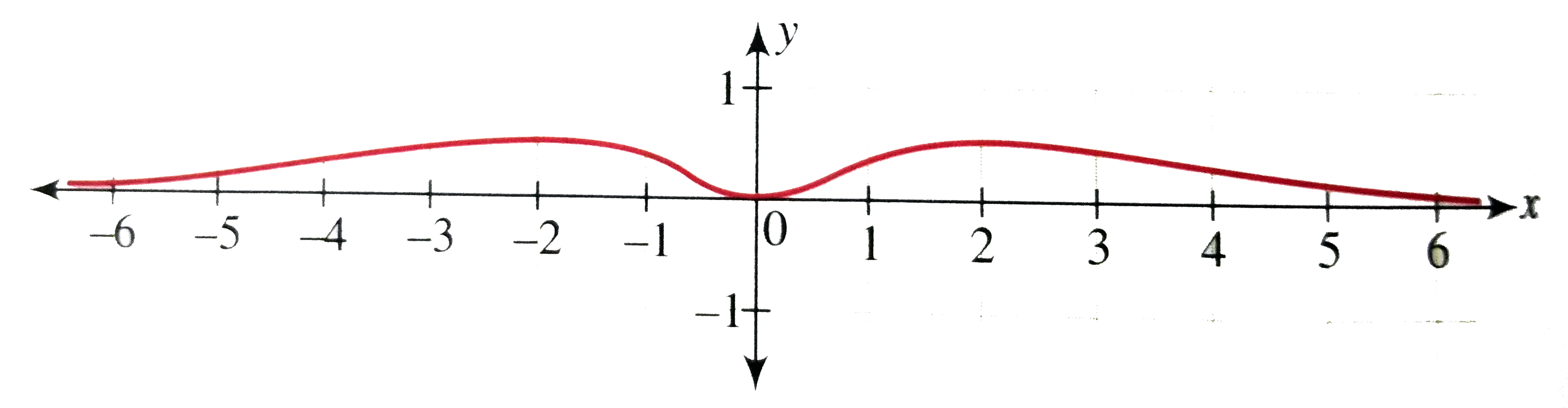
Since, f(x) is differentiable at x=0,the graph touches the x-axis at x=0
The x-axis is also asymptote to the curve.
Also since f(x) is an even function, x=−2 is also the point of maxima.
Range of the function is [0,f(2)] or [0,4/e2]
Clearly, f(x)≠1, hence f(x)=1 has no roots.
Topper's Solved these Questions
Similar Questions
Find the derivative of y=sin2x−cosx
04:13View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
06:25View SolutionLet f be a non-negative function defined on the interval [0,1]. If ∫x0√1−(f′(t))2dt=∫x0f(t)dt,0≤x≤1,and f(0)=0, then
04:35View SolutionDiscuss the maxima and minima of the function f(x)=x23−x43. Draw the graph of y=f(x) and find the range of f(x).
05:57View SolutionFind the points of local maxima or minima of following functions f(x)=(x-1)^(3)(x+2)^(2)
View SolutionFind the points of maxima minima of f(x)=x3−12x. Also draw the graph of this functions.
03:43View SolutionDraw graph of f(x) =x|x−2| and hence find points of local maxima/minima.
01:25View SolutionDraw the graph of f(x)=x2e−|x|
i) Find the point of maxima/minima.
ii) Find the asymptote is any.
iii) Find the range of the function.
iv) Find the number of roots of the equation f(x)=101:15View SolutionFind the points of local maxima/minima of function
f(x)=−(x−1)3(x+1)206:28View SolutionFind the points of local maxima/minima of following functions
(i) f(x)=x+1x
(ii) f(x) = cosec x
Hence find maxima and minima values of f(x).06:24View Solutionవేర్ల ద్వారా శాఖీయోత్పత్తి జరిపే మొక్కbr i) డాలియాbr ii) ముల్లంగిbr iii) క్యారెట్br iv) క్యాబేజిbr
07:05View SolutionDiscuss the maxima and minima of the function f(x)=x23−x43. Draw the graph of y=f(x) and find the range of f(x).
09:27View SolutionDraw the graph of f(x)=x2e−|x|
i) Find the point of maxima/minima.
ii) Find the asymptote is any.
iii) Find the range of the function.
iv) Find the number of roots of the equation f(x)=105:22View SolutionFind the maxima and minima of the following functions :
f(x)=x2 In x ,05:20View SolutionFind the points of maxima minima of f(x) =x3−12x. Also draw the graph of this functions.
08:26View SolutionDraw graph of f(x) =x|x−2| and hence find points of local maxima/minima.
04:36View Solution

