Let two parallel lines L1 and L2 with positive slope are tangent to the circle C1 : x2+y2−2x16y+64=0. If L1 is also tangent to the circle C2 : x2+y2−2x+2y−2=0 and the equation of L2 is a√ax−by+c−a√a=0 where a,b,c in N. then find the value of a+b+c7
The correct Answer is:2√2x−y+11−2√2=0
S1≡x2+y2−2x−16y+64=0
S2≡x2+y2−2x+2y−2=0
C1≡(1,8),r1=1
C2≡(1,−1),r2=2
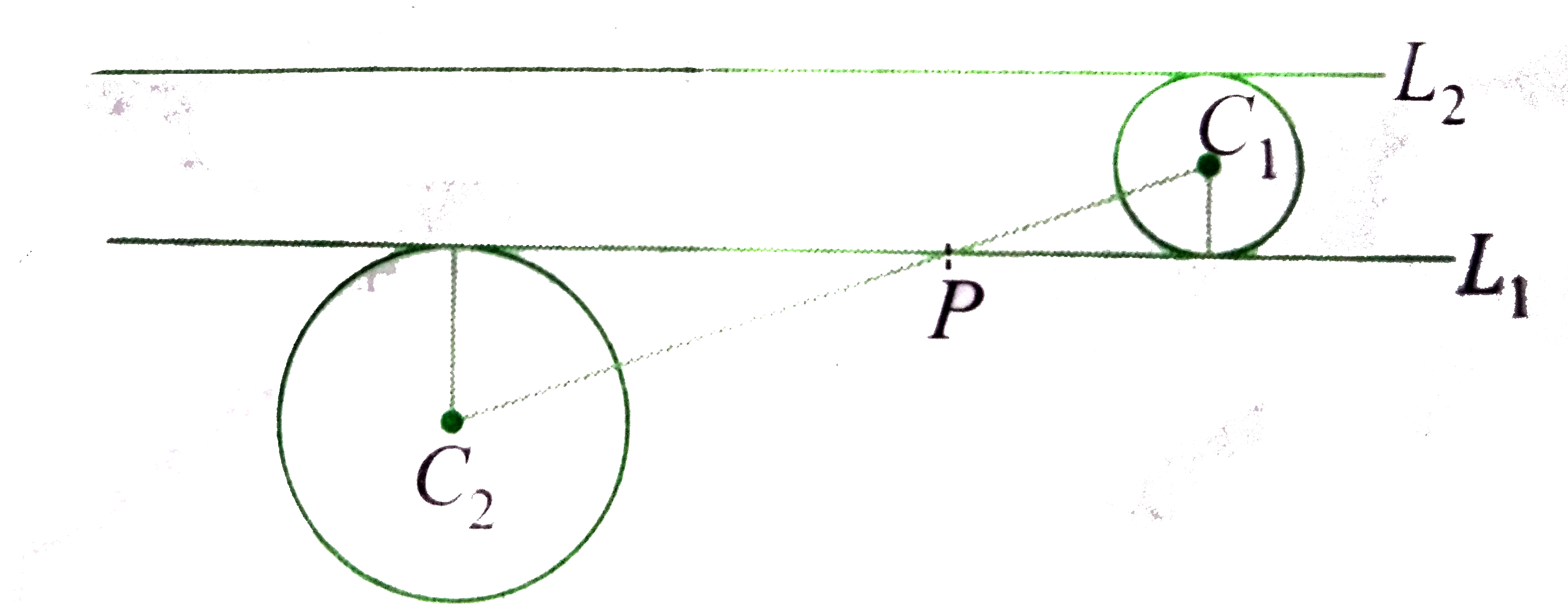
Line L1 is transverse common tangent of S1 and S2.
So, P divides C1C2 internally in the ratio of 1:2.
⇒P≡(1+21+2,−1+161+2)≡(1,5)
Thereforem equation of L1 with positive slope is
(y−5)=m(x−1)
or mx−y−m+5=0
This is also tangnet to S2 .
So, we have
∣∣∣m+1−m+5√m2+1∣∣∣=2
∴m=2√2
So, equation of L1 is 2√2x−y−2√2+5=0
Thus, equation of L2 is 2√2x−y+k=0.
From the figure, distance between parallel lines is 2.
∴∣∣∣k+2√2−53∣∣∣=2
∴k=±6+5−2√2
Therefore, equation of L2 with + ve y-intercept is
√2x−y+11−2√2=0
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.105videos
CIRCLE
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.114videos
CIRCLE
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.85videos
CIRCLE
- BOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension11videos
BINOMIAL THEOREM
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Comprehension Type8videos
CIRCLES
Similar Questions
Let two parallel lines L1 and L2 with positive slope are tangent to the circle C1 : x2+y2−2x16y+64=0. If L1 is also tangent to the circle C2 : x2+y2−2x+2y−2=0 and the equation of L2 is a√ax−by+c−a√a=0 where a,b,c in N. then find the value of a+b+c7
View SolutionIf y=c is a tangent to the circle x2+y2–2x+2y–2=0 at (1, 1), then the value of c is
View Solution
Knowledge Check
- Question 1 - Select One
If a line, y = mx + c is a tangent to the circle, (x−1)2+y2=1 and it is perpendicular to a line L1 , where L1 is the tangent to the circle x2+y2=8 at the point (2, 2), then :
AC2+2c−1=0Bc2−2c+1=0Cc2−2c−1=0Dc2+2c+2=0 - Question 2 - Select One
The value of c, for which the line y=2x+c is a tangent to the circle x2+y2=16, is :
A−16√5B4√5C16√5D20 - Question 3 - Select One
The value of c, for which the line y=2x+c is a tangent to the circle x2+y2=16, is :
A−16√5B4√5C16√5D20
If L1andL2, are the length of the tangent from (0, 5) to the circles x2+y2+2x−4=0andx2+y2−y+1=0 then
View SolutionIf a line, y = mx + c is a tangent to the circle, (x−1)2+y2=1 and it is perpendicular to a line L1 , where L1 is the tangent to the circle x2+y2=8 at the point (2, 2), then :
View SolutionA tangent line L is drawn at the point (2, -4) on the parabola y2=8x. If the line L is also tangent to the circle x2+y2=a, then 'a' is equal to ________.
View SolutionIf y=mx+c is a tangent to the circle (x–3)2+y2=1 and also the perpendicular to the tangent to the circle x2+y2=1 at (1√2,1√2), then
View SolutionIf y=mx+c is a tangent to the circle (x–3)2+y2=1 and also the perpendicular to the tangent to the circle x2+y2=1 at (1√2,1√2), then
View Solution

