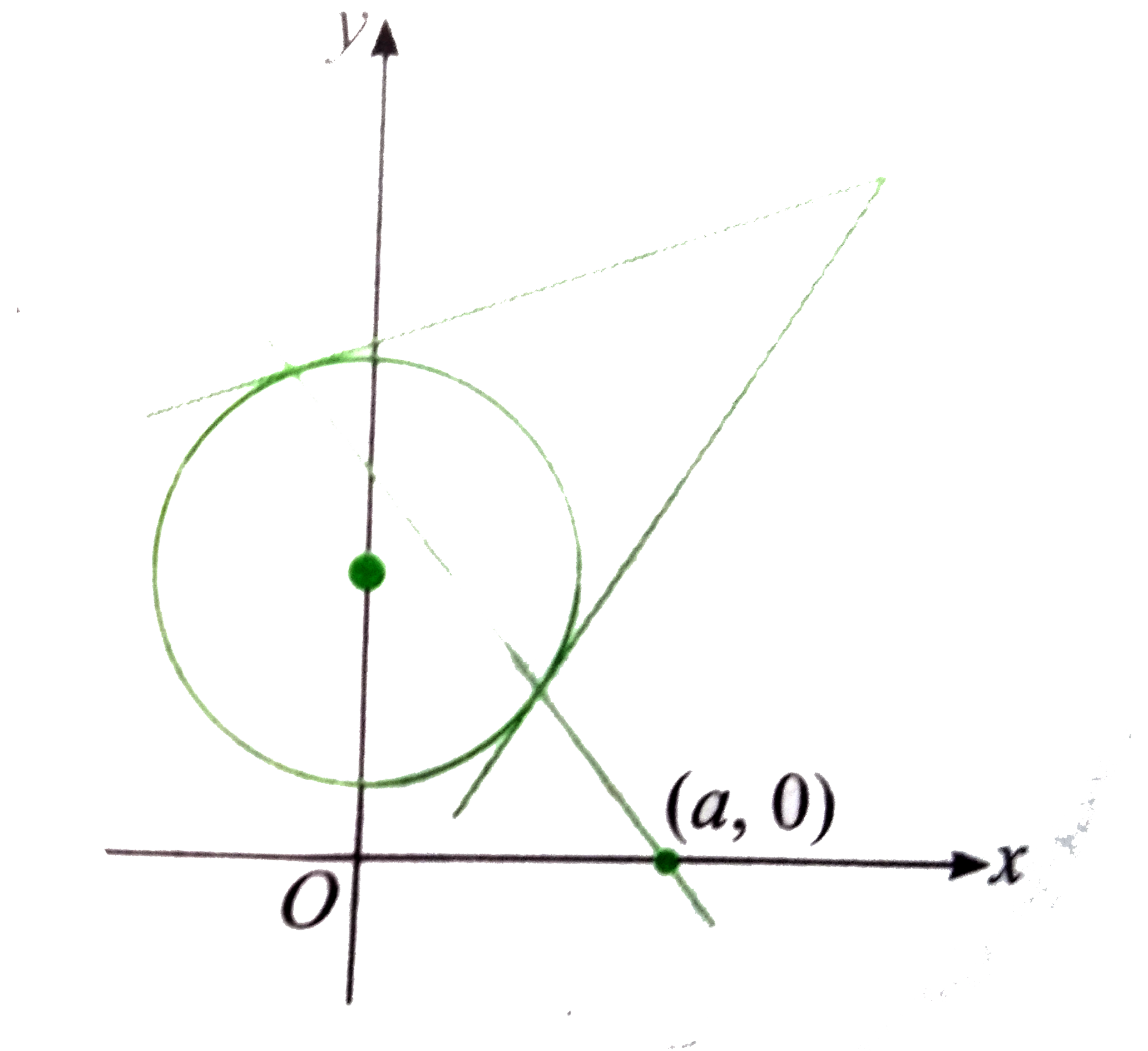
A circle with radius |a| and center on the y-axis slied along it and a variable line through (a, 0) cuts the circle at points PandQ . The region in which the point of intersection of the tangents to the circle at points P and Q lies is represented by y2≥4(ax−a2) (b) y2≤4(ax−a2) y≥4(ax−a2) (d) y≤4(ax−a2)Solution in Bengali
y2≥4(ax−a2)
y2≤4(ax−a2)
y≥4(ax−a2)
y=4(ax−a2)
The correct Answer is:1
Let the center be (0,α) . Then the equation of the circle is x2+(y−α)2=|a|2.
So, the equation of chord of contact for P(h,k) is xh+yk−α(y+k)+α2−a2=0
It passes through (a,0).
Therefore,
α2−αk+ah−a20=0
As α is real,
k2−4(ah−a2)≥0
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - Multiple Correct Anser Type29videos
CIRCLE
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - Linked Comprehension Type (For Problem 1-3)3videos
CIRCLE
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.172videos
CIRCLE
- BOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension11videos
BINOMIAL THEOREM
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Comprehension Type8videos
CIRCLES
Similar Questions
about to only mathematics
02:16View SolutionEach side of a square is of length 6 units and the centre of the square Is ( - 1, 2). One of its diagonals is parallel to x+y=0. Find the co-ordinates of the vertices of the square.
04:41View SolutionThree concentric circles of which the biggest is x2+y2=1, have their radii in A.P If the line y=x+1 cuts all the circles in real and distinct points. The interval in which the common difference of the A.P will lie is:
08:07View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is04:43View Solution
In the diagram as shown, a circle is drawn with centre C(1,1) and radius 1 and a line L. The line L is tangent to the circle at Q. Further L meets the y-axis at R and the x-axis at P in such a way that the angle OPQ equals θ where 0<θ<π2.
Coordinate of Q is04:39View SolutionAny circle through the point of intersection of the lines x+√3y=1 and √3x−y=2 intersects these lines at points PandQ . Then the angle subtended by the arc PQ at its center is 1800 (b) 900 (c) 1200 depends on center and radius
08:08View SolutionA circle with radius |a| and center on the y-axis slied along it and a variable line through (a, 0) cuts the circle at points PandQ . The region in which the point of intersection of the tangents to the circle at points P and Q lies is represented by y2≥4(ax−a2) (b) y2≤4(ax−a2) y≥4(ax−a2) (d) y≤4(ax−a2)
05:31View Solutionउस वृत्त का समीकरण निकालें जो दिए गए वृत्तों
x2+y2−6x+2y+4=0 तथा x2+y2+2x−4y−6=0 के छेदन-बिंदुओं से जाता है तथा जिसका केंद्र रेखा y=x पर स्थित है।04:41View SolutionIf the circle x^(2)+y^(2)=1 A variable tangent of the ellipse x^(2)+2y^(2)=4 Is cut at points P and Q. Then the point of intersection of the tangent lines drawn at P and Q is
04:54View SolutionThe line 2x−y+1=0 is tangent to the circle at the point (2, 5) and the center of the circle lies on x−2y=4 . Then find the radius of the circle.
03:11View SolutionAny circle through the point of intersection of the lines x+√3y=1 and √3x−y=2 intersects these lines at points PandQ . Then the angle subtended by the arc PQ at its center is 1800 (b) 900 (c) 1200 depends on center and radius
01:58View SolutionA circle with radius |a| and center on the y-axis slied along it and a variable line through (a, 0) cuts the circle at points PandQ . The region in which the point of intersection of the tangents to the circle at points P and Q lies is represented by y2≥4(ax−a2) (b) y2≤4(ax−a2) y≥4(ax−a2) (d) y≤4(ax−a2)
03:36View SolutionA tangent at a point on the circle x2+y2=a2 intersects a concentric circle C at two points PandQ . The tangents to the circle X at PandQ meet at a point on the circle x2+y2=b2. Then the equation of the circle is x2+y2=ab x2+y2=(a−b)2 x2+y2=(a+b)2 x2+y2=a2+b2
05:02View SolutionA circle with radius |a| and center on the y-axis slied along it and a variable line through (a, 0) cuts the circle at points PandQ . The region in which the point of intersection of the tangents to the circle at points P and Q lies is represented by y2≥4(ax−a2) (b) y2≤4(ax−a2) y≥4(ax−a2) (d) y≤4(ax−a2)
07:31View SolutionAny circle through the point of intersection of the lines x+√3y=1 and √3x−y=2 intersects there lines at points PandQ . Then the angle subtended by the are PQ at its center is 1800 (b) 900 (c) 1200 depends on center and radius
05:09View SolutionThe line 2x−y+1=0 is tangent to the circle at the point (2, 5) and the center of the circle lies on x−2y=4 . Then find the radius of the circle.
04:13View Solution

