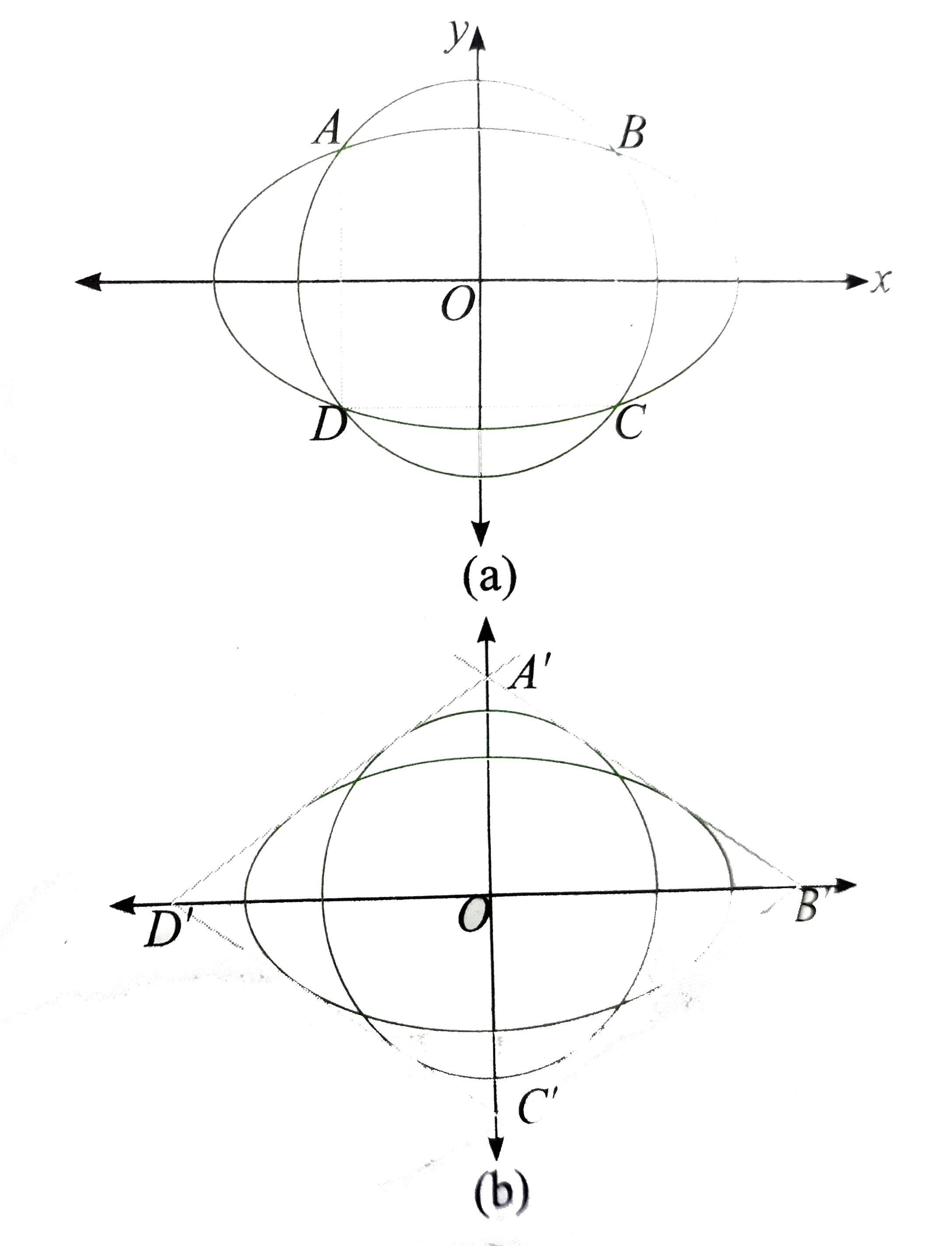
Curves C1:x2+y2=r2andC2:x216+y29=1 intersect at four distinct points A,B,C and D. Their common tangents from a parallelogram PQRS. Q. If ABCD is sqyare, then the value of 25r2 is Solution in Bengali
√120
√12
√15
none of these
Tangent of slope m to the circle and ellipse are, respectively,
y=mx±√r2m2+r2
and y=mx±√16m2+9
For common tangent,
r2m2+r216m2+9
Also, if A',B',C', and D' is squre, then
m=±
orr2+r2=25
or r=5√2
Similar Questions
If tangent at (1, 2) to the circle C1:x2+y2=5 intersects the circle C2:x2+y2=9 at A and B and tangents at A and B to the second circle meet at point C, then the co- ordinates of C are given by
02:59View SolutionC1:x2+y2=r2andC2:x216+y29=1 interset at four distinct points A,B,C, and D. Their common tangents form a peaallelogram A'B'C'D'.
If A'B'C'D' is a square, then the ratio of the area of circle C1 to the area of circumcircle of ΔA'B'C' is02:42View Solutionबिन्दु A से वक्रों C1:x2+y2=8 एवं C2:y2=16x की उभयनिष्ठ स्पर्श रेखाएं खींची जाती है।
C1 एवं C2 के मध्य ऋणात्मक प्रवणता वाली उभयनिष्ट स्पर्श रेखा का y-अन्तःखण्ड है06:09View Solutionमाना C1:x2+y2=1 तथा C2:x2+y2−(λ+6)x+(8−2λ)y−3=0 है। यदि λचार है तो C1 एवं C2 के उभयनिशस्ति बिंदु से C1 पर खींची गयी स्पर्श रेखाओं के प्रतिच्छेद बिंदु का बिन्दुपथ होगा
06:12View SolutionC1andC2 are two curves intersecting at (1,1),C1 satisfy dydx=y2−x22xyandC2 satisfy dydx=2xy−y2+x2 then area bounded by these two curves is
05:40View SolutionConsider the curve
x216+y29=1
Find the points of intersection of the curve with x-axis.01:52View SolutionC1:x2+y2=r2andC2:x216+y29=1 interset at four distinct points A,B,C, and D. Their common tangents form a peaallelogram A'B'C'D'.
if A'B'C'D' is a square, then r is equal to04:01View SolutionThe slopes of the common tangents of the hyperbolas x29−y216=1 and y29−x216=1, are
03:00View Solution

