Question

Find all the value of $m$
for which the equation ${\sin }^{2}x+(m-3)\sin x+m=0$
has real
roots.

Explanation

Put sin x = t , the equation is
`f(t) = t^(2) - (m - 3) t + m = 0` (1)
Since sin x `in` [ -1, 1], the given equation will have real roots if (10 has at least root in [ - 1, 1].
Now (1) has real roots if` (m - 3)^(20 - 4m ge 0` . Thus ,
m `in ( - infty, 1] cup [ 9, infty)`
If both roots in `[-1, 1] then f(1) = 4 gt 0`,
`f(-1) = 1 + m - 3 + m gt ` ,
For m `gt`1, Product of roots is greater then 1, which is not possible .
Therefore , exactly one root of (1) will lie in [-1, 1] .
Hence , `f(-1) f(1) le 0`
`rArr ( 1 + m - 3 + m) ( 1 - m + 3 + m ) le 0 `
`rArr m le` 1
Alternative Solution :
we have
`t^(2) -= ( m - 3) t + M = 0`
or `t^(2) + 3t = m(t - 1) , where t in` [ -1, 1].
So, the graph of `y = t^(2) + 3t` must intersect the graph of `y = m ( t- 1)`
at least once for t `in [-1, 1]`.
Graph of `y = m (t - 1)` is a line through (1, 0) and has veriable slope.
Graph of `y = t^(2) + 3t` is parabola passing though (0, 0) and (-3, 0) and concave upward.
Graph of both the relations are as as shown in the following figure.

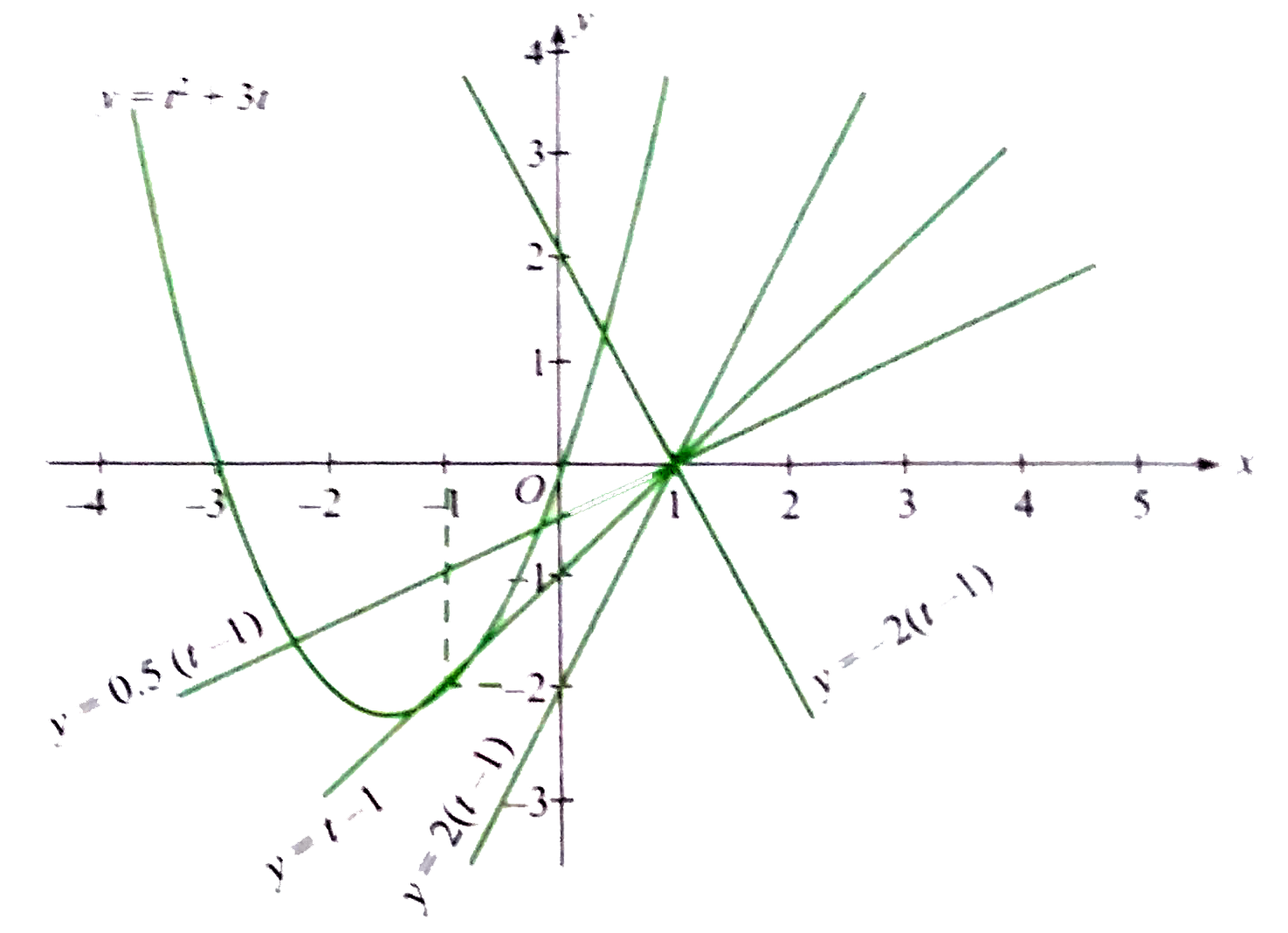
For parabola, `(dy)/(dt) = 2t + 3 . So ((dy)/(dt))_(((-1, -2) ))=1`,
Therefore, equation of tangent to parabola at (-1, -2) is y = t -1,
Which is line through (1, 0) .
Now , if slope of line than 1, then it intersect parabola for t`in [ -1, 1] ` .
Thus, for solution of the equation `x^(2) + 3t = m (t - 1)` , we must have m `le`1.