Question

Consider the inequation $9^x-a{3}^x-a+3\le 0$, where a is real parameter.
The given inequality has at least one negative solution for $a\in$
(a) $(-\infty ,2)$
(b) $(3,\infty )$
(c) $(-2,\infty )$
(d) $(2,3)$

Options

A. `(-oo,2)`
B. `(3,oo)`
C. `(-2,oo)`
D. `(2,3)`

Answer

4

Explanation

Given that `9^(x) - a3^(x) - a+3 le 0`
Let `t = 3^(x)`. Then,
`t^(2) -at - a + 3 le 0`
or `t^(3) + 3 le a(t+1) " "(1)`
where `t in R^(+), AA x in R`
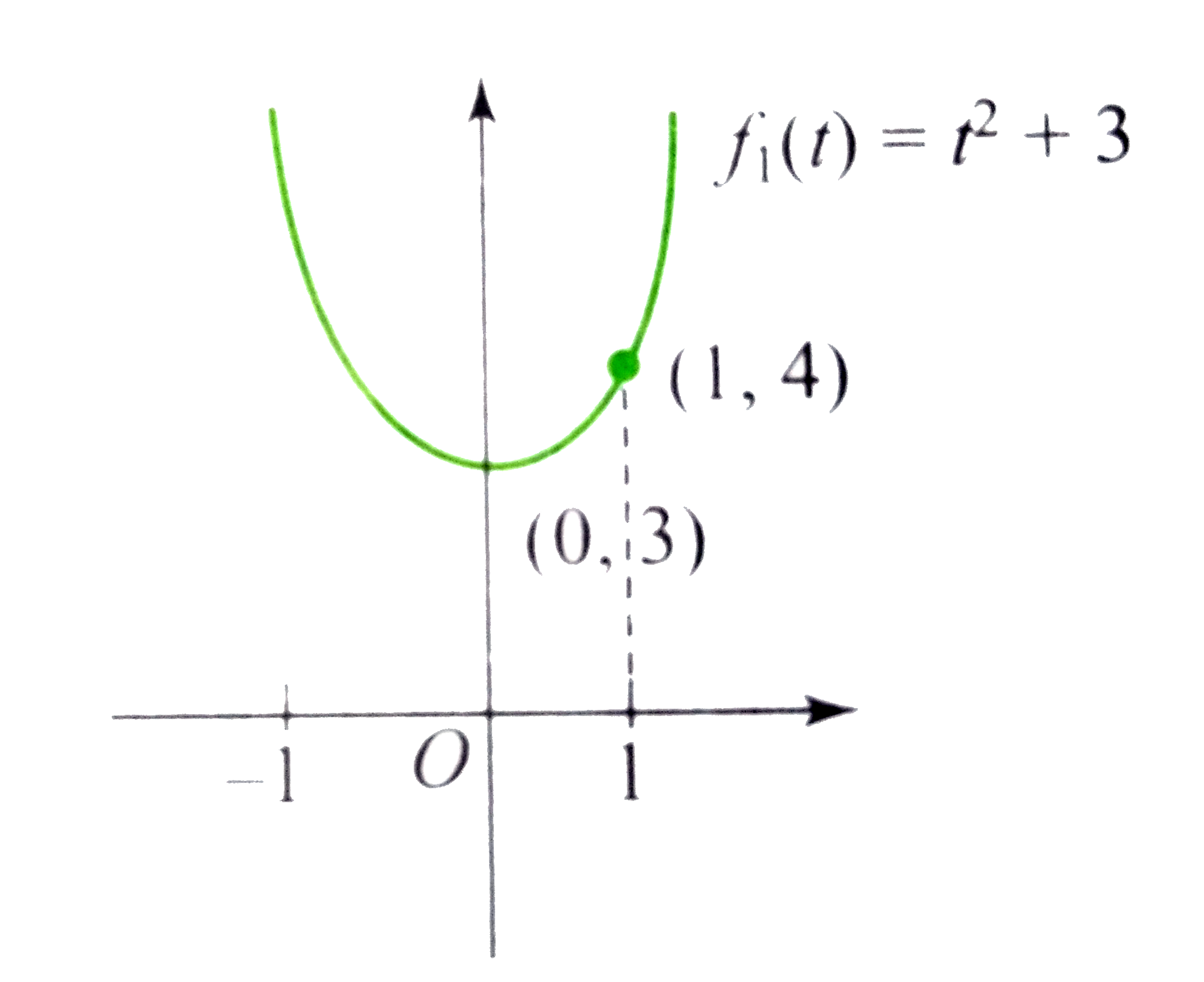
Let `f_(1)(t) = t^(2) + 3` and
`f_(2)(t) = a(t+1)`.
For `x lt 0, t in (0,1)` Than means (1) should have at least one solution in `t in (0,1)`. From the (1), it is obvious that ` a in R^(+)`. Now `f_(2)(t) = a(t+1)` represents a straight line. It should meed the curve `f_(1)(t) = t^(2) + 3`, at least once in `t in (0,1)`
`f_(1)(0) = 3,f_(1)(1)= 4, f_(2)(0) =a,f_(2)(1) =2a`
If `f_(1)(0) = f_(2) (0)`, Then `a=3 , "if" f_(1)(1) =f_(2)(1)`, then a=2 . Hence the required range is `a in (2,3)`