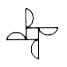A figure has a rotational symmetry of order more than 1, the angle of rotation can be_______
21∘
22∘
23∘
24∘
To solve the question regarding the angle of rotation for a figure with a rotational symmetry of order more than 1, we can follow these steps:
1. Understand Rotational Symmetry:
- A figure has rotational symmetry if it can be rotated about a central point and still look the same at certain angles. The order of rotational symmetry indicates how many times the figure looks the same in a full rotation of 360 degrees.
2. Identify the Angle of Rotation:
- The angle of rotation can be calculated by dividing 360 degrees by the order of symmetry. If the order is more than 1, it means the figure looks the same at multiple points during the rotation.
3. Calculate Possible Angles:
- Since the order of symmetry is more than 1, we can say:
Angle of rotation=360∘n
where n is the order of symmetry and n>1.
4. Determine Factors of 360:
- The angles of rotation must be factors of 360 degrees. The factors of 360 degrees are: 1, 2, 3, 4, 5, 6, 8, 9, 10, 12, 15, 18, 20, 24, 30, 36, 40, 60, 72, 90, 120, 180, and 360.
5. List Possible Angles:
- For an order of symmetry greater than 1, we can take factors of 360 that correspond to n=2,3,4,…. For example:
- If n=2, angle = 3602=180∘
- If n=3, angle = 3603=120∘
- If n=4, angle = 3604=90∘
- If n=5, angle = 3605=72∘
- If n=6, angle = 3606=60∘
- If n=8, angle = 3608=45∘
- If n=9, angle = 3609=40∘
- If n=10, angle = 36010=36∘
- If n=12, angle = 36012=30∘
- If n=15, angle = 36015=24∘
6. Conclusion:
- Since the question specifies that the order of symmetry is more than 1, the angle of rotation can be any of the factors of 360 degrees that correspond to n>1. The smallest angle of rotation that meets this criterion is 24∘ (for n=15).
Final Answer:
The angle of rotation can be 24 degrees.
- A figure has rotational symmetry if it can be rotated about a central point and still look the same at certain angles. The order of rotational symmetry indicates how many times the figure looks the same in a full rotation of 360 degrees.
- The angle of rotation can be calculated by dividing 360 degrees by the order of symmetry. If the order is more than 1, it means the figure looks the same at multiple points during the rotation.
- Since the order of symmetry is more than 1, we can say:
Angle of rotation=360∘n
where n is the order of symmetry and n>1.
- The angles of rotation must be factors of 360 degrees. The factors of 360 degrees are: 1, 2, 3, 4, 5, 6, 8, 9, 10, 12, 15, 18, 20, 24, 30, 36, 40, 60, 72, 90, 120, 180, and 360.
- For an order of symmetry greater than 1, we can take factors of 360 that correspond to n=2,3,4,…. For example:
- If n=2, angle = 3602=180∘
- If n=3, angle = 3603=120∘
- If n=4, angle = 3604=90∘
- If n=5, angle = 3605=72∘
- If n=6, angle = 3606=60∘
- If n=8, angle = 3608=45∘
- If n=9, angle = 3609=40∘
- If n=10, angle = 36010=36∘
- If n=12, angle = 36012=30∘
- If n=15, angle = 36015=24∘
- Since the question specifies that the order of symmetry is more than 1, the angle of rotation can be any of the factors of 360 degrees that correspond to n>1. The smallest angle of rotation that meets this criterion is 24∘ (for n=15).
The angle of rotation can be 24 degrees.
Topper's Solved these Questions
- PREP TEST 1 BOOK - MTG IIT JEE FOUNDATIONCHAPTER - PREP TEST 1 EXERCISE - SECTION-B
29 Videos
- PERIMETER AND AREA BOOK - MTG IIT JEE FOUNDATIONCHAPTER - PERIMETER AND AREA EXERCISE - OLYMPIAD/HOTS CORNER
20 Videos
- PREP TEST 2 BOOK - MTG IIT JEE FOUNDATIONCHAPTER - PREP TEST 2 EXERCISE - SECTION-B
30 Videos
Similar Questions
Knowledge Check
A square has a rotational symmetry of order
A1B2C3D4Which has no rotation of symmetry ?
AhexagonalBOrthochromicCCubicDTriclinicWhich has no rotaition of symmetry ?
AHexagonalBOrthorhombicCCubicDTriclinic
Can we have a rotational symmetry of order more than 1 whose angle of rotation is
(i) 45∘ (ii) 17∘?
View SolutionDefine order of rotational symmetry.
View SolutionState whether the figure shows rotational symmetry. If yes then what is the order of rotational symmetry?

View SolutionThe order of rotational symmetry of regular octagon is
View SolutionWhich of the following letters has rotational symmetry of order more than 1 but not any line of symmetry?
View Solution