Let Sk,k=1,2,….100 denote the sum of the infinite geometric series whose first term is k−1K! and the common ratio is 1k then the value of (100)2100!+100Σk=1∣∣(k2−3k+1)Sk∣∣ is ____________Solution in Punjabi
Sk=a1−r=k−1k!1−1k=kk!=1(k−1)!
Now, 100∑k=2∣∣(k2−3k+1)Sk∣∣=100∑k=2∣∣∣(k2−3k+1)⋅1(k−1)!∣∣∣
=100∑k=2∣∣∣(k−1)(k−2)=k(k−1)!∣∣∣
=∣∣∣10!−21!∣∣∣+∣∣∣21!−32!∣∣∣+∣∣∣32!−43!∣∣∣+.........+∣∣∣9998!−10099!∣∣∣
=(21!−10!)+(21!−32!)+(32!−43!)+.........+(9998!−10099!)
=3−10099=3−(100)2100!
∴(100)2100!+100∑k=2∣∣(k2−3k+1)Sk∣∣=3.
Topper's Solved these Questions
- SEQUENCES AND SERIESBOOK - ARIHANT MATHSCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Subjective Type Questions)
24 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
21 Videos
- SETS, RELATIONS AND FUNCTIONS BOOK - ARIHANT MATHSCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
12 Videos
Similar Questions
The pth, (2p)th and (4p)th terms of an AP, are in GP, then find the common ratio of GP.
View SolutionProve that 2sin2(π6)−sec2(π3)=−72
View SolutionLet R={(3,3),(6,6),(9,9),(6,12),(3,9),(3,12),(12,12),(3,6)} is a relation on set A={3,6,9,12} then R is a) an equivalence relation b) reflexive and symmetric only c) reflexive and transitive only d) reflexive only
View SolutionFind the sum of first 100 terms of the series whose general term is given by ak=(k2+1)k!
View SolutionLet Sk, where k=1,2,....,100, denotes the sum of the infinite geometric series whose first term is k−1k! and the common ratio is 1k. Then, the value of 1002100!+100∑k=2∣∣(k2−3k+1)Sk∣∣ is....
View SolutionLet Sk be sum of an indinite G.P whose first term is 'K' and commmon ratio is 1k+1. Then Σ10k=1Sk is equal to _________.
View Solutionमान लीजिये कि Sk,k=1,2,...,100 उस अनन्त गुणोत्तर श्रेणी का योग प्रदर्शित करता है जिसका प्रथम पद k−1k!तथा सार्वअनुपात 1kहै, तो 1002100!+Σ100k=1∣∣(k2−3k+1)Sk∣∣का मान क्या है ?
View Solutionमाना की Sk=1,2,……..100 उस अनंत गुणोत्तर श्रेणी का योग प्रदर्शित करता है जिसका प्रथम पद k−1k! तथा सार्व अनुपात 1k है तो 1002100!+100∑k=1∣∣(k2−3k+1)Sk∣∣का मान है
View SolutionLet Sk, be the sum of an infinite geometric series whose first term is kand common ratio is kk+1(k>0) . Then the value of ∞∑k=1(−1)kSk is equal to
View Solutionयदि एक अनंत गुणोत्तर श्रेणी जिसका प्रथम पद k और सरवणुपात kk+1(k>0) का योग प्रदर्शित करता है तब ∞∑k=1(−1)kSkका मान होगा
View Solutionमाना की Sk=1,2,……..100 उस अनंत गुणोत्तर श्रेणी का योग प्रदर्शित करता है जिसका प्रथम पद k−1k! तथा सार्व अनुपात 1k है तो 1002100!+100∑k=1∣∣(k2−3k+1)Sk∣∣का मान है
View SolutionLet Sk, where k=1,2,....,100, denotes the sum of the infinite geometric series whose first term is k−1k! and the common ratio is 1k. Then, the value of 1002100!+100∑k=2∣∣(k2−3k+1)Sk∣∣ is....
View SolutionLet Sk be sum of an indinite G.P whose first term is 'K' and commmon ratio is 1k+1. Then Σ10k=1Sk is equal to _________.
View SolutionLet Sk,k=1,2,….100 denote the sum of the infinite geometric series whose first term is k−1K! and the common
ration is 1k then the value of (100)2100!+Σ100k=1∣∣(k2−3k+1)Sk∣∣ is ____________
View SolutionFor k=2,3,…, let Sk denote the sum of the infinite G.P. whose first term is k2+k−2 and common ratio is 1k, then ∞Σk=1Sk2k=
View SolutionLet sk,k=1,2,3,…,100 denote the sum of the infinite geometric series whose first term is k−1⌊k and the common ratio is 1k. Then, the value of
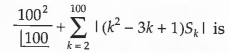 is
is
View Solution
