There are two groups of subjects, one of which consists of 5 science subjects and 3 Engineering subjects and the other consists of 3 science and 5 Engineering subjects. An unbiased die is cast. If number 3 or 5 turns up, a subject from 1 is selected otherwise a subject is selected from group 2. The probability that an Engineering subject is selected ultimately, isSolution in Punjabi
713
917
1324
1120
The correct Answer is:(c)
Step by step video & image solution for There are two groups of subjects, one of which consists of 5 science subjects and 3 Engineering subjects and the other consists of 3 science and 5 Engineering subjects. An unbiased die is cast. If number 3 or 5 turns up, a subject from 1 is selected otherwise a subject is selected from group 2. The probability that an Engineering subject is selected ultimately, is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Exercise For Session 415videos
PROBABILITY
- BOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Exercise (Single Option Correct Type Questions)29videos
PROBABILITY
- BOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Exercise For Session 215videos
PROBABILITY
- BOOK - ARIHANT MATHSCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)28videos
PERMUTATIONS AND COMBINATIONS
- BOOK - ARIHANT MATHSCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)52videos
PRODUCT OF VECTORS
Similar Questions
The probability of getting exactly two heads when tossing a coin three times is
03:03View SolutionThere are 2 vans each having numbered seats, 3 in the front and 4 at the back. There are 3 girls and 9 boys to be seated in the vans. The probablity of 3 girls sitting together in a back row on adjacent seats, is
01:30View SolutionIf P(A)=0.8,P(B)=0.5 , then P(A∩B) lies in the interval
01:11View SolutionThere is a rectangular sheet of dimension (2m−1)×(2n−1), (where m>0,n>0) It has been divided into square of unit area by drawing line perpendicular to the sides. Find the number of rectangles having sides of odd unit length.
03:07View SolutionLet O be the origin and let PQR be an arbitrary triangle. The point S is such that ¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OQ+¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OP+¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OR+¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OS Then the triangle PQR has S as its
02:00View SolutionFor examination, a candidate has to select 7 subjects from 3 different groups A, B, C which contain 4, 5, 6 subjects, respectively. The number of different way in which a candidate can make his selection if he has to select at least 2 subjects form each group is
View SolutionTwo subjects are compulsory for a student in an examination. In how many ways can a student select 5 subjects out of given 10 subjects?
04:25View SolutionIn how many ways can 5 subjects be chosen from 9 subjects if three subjects are compulsory?
02:05View Solutionज्ञात कीजिए कि उपलब्ध 9 विषयों में से एक छात्र 5 विषयों का चयन कितने प्रकार से कर सकता है यदि प्रत्येक छात्र के लिए 2 विशिष्ट विषय अनिवार्य हैं ?
06:56View Solutionज्ञात कीजिए कि 9 विषयो में से 5 विषयों का चयन कितने प्रकार से किया जा सकता है , यदि 3 विषय अनिवार्य है ?
03:10View SolutionThere are two groups of subjects one of which consists of 5 Science and 3 Engineering subjects and the other consists of 3 Science and 5 Engineering subjects. An unbiased die is cost. If 3 or 5 turns up, a subject is selected at random from the first group, other wise the subject is selected at random from the second group. The probability that an Engineering subject is selected ultimatly is
04:04View Solutionஒரு வகுப்பில் உள்ள 40 மாணவர்கள் ,கணிதம் ,அறிவியல் மற்றும் சமூக அறிவியல் ஆகிய மூன்று பாடங்களில் பெற்ற மதிப்பெண்களின் சராசரி மற்றும் திட்ட விலக்கம் கீழே கொடுக்கப்பட்டுள்ளன . இந்த மூன்று பாடங்களின் எது அதிக நிலைத் தன்மைக் கொண்டது ?
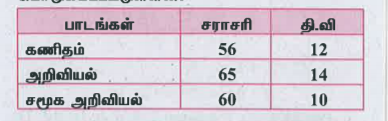 03:06View Solution
03:06View SolutionFor examination, a candidate has to select 7 subjects from 3 different groups A, B, C which contain 4, 5, 6 subjects, respectively. The number of different way in which a candidate can make his selection if he has to select at least 2 subjects form each group is?
01:50View Solutionविषयों के दो समूह इस प्रकार है कि एक में 5 विज्ञान विषय और 3 अभियांत्रिकी विषय है तथा दूसरे समूह में 3 विज्ञान व 5 अभियांत्रिकी विषय है । एक अनभिनत पासा डाला जाता है यदि अंक 3 5 आते है तो प्रथम समूह में यादृच्छ रूप से विषय चुना जाता है अन्यथा दूसरे समूह से विषय चुना जाता है। एक अभियांत्रिकी विषय के चयन की प्रायिकता ज्ञात कीजिए।
01:59View Solutionविषयों के दो समूह हैं जिनमें प्रथम समूह में 5 विज्ञान के विषय है और 3 इंजीनियरिंग के विषय है तथा द्वितीय समूह में 3 विज्ञान के और 5इंजीनियरिंग के विषय है। एक निष्पक्षपाती पाँसे को उछाला जाता है। यदि संख्या 3 या 5 आती है तो प्रथम समूह में से यादृच्छया एक विषय चुना जाता है, अन्यथा द्वितीय समूह से चुना जाता है तो इंजीनियरिंग विषय को चुनने की प्रायिकता ज्ञात कीजिये।
03:11View Solutionविषयो के दो समूह है पहले समूह में 5 विज्ञान के विषय तथा 3 अभियांत्रिकी के विषय है तथा दुसरे में 3 विज्ञान तथा 5 अभियांत्रिकी के विषय है एक शुद्ध पासा फेंका जाता है यदि पासे पर 3 या 5 आता है तो पहले समूह से यादृच्छिक रूप से एक विषय चुना जाता है अन्यथा दुसरे समूह से एक विषय चुना जाता है यदि एक अभियांत्रिकी विषय के चुने जाने की प्रायिकता P है तब -
03:35View Solution

