The numbers 1,2,3,.., n are arranged in a random order. The probability that the digits 1,2,3,..,k(n>k) appears as neighbours in that order is Solution in Punjabi
1n!
K!n!
(n−k)!n!
(n−k+1)!n!
The correct Answer is:(d)
Step by step video & image solution for The numbers 1,2,3,.., n are arranged in a random order. The probability that the digits 1,2,3,..,k(n gt k) appears as neighbours in that order is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROBABILITYBOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Probability Exercise 1: Single Option Single Correct Type Question
1 Videos
- PROBABILITYBOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Exercise (More Than One Correct Option Type Questions)
15 Videos
- PROBABILITYBOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Exercise For Session 4
15 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - ARIHANT MATHSCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
28 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHSCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
52 Videos
Similar Questions
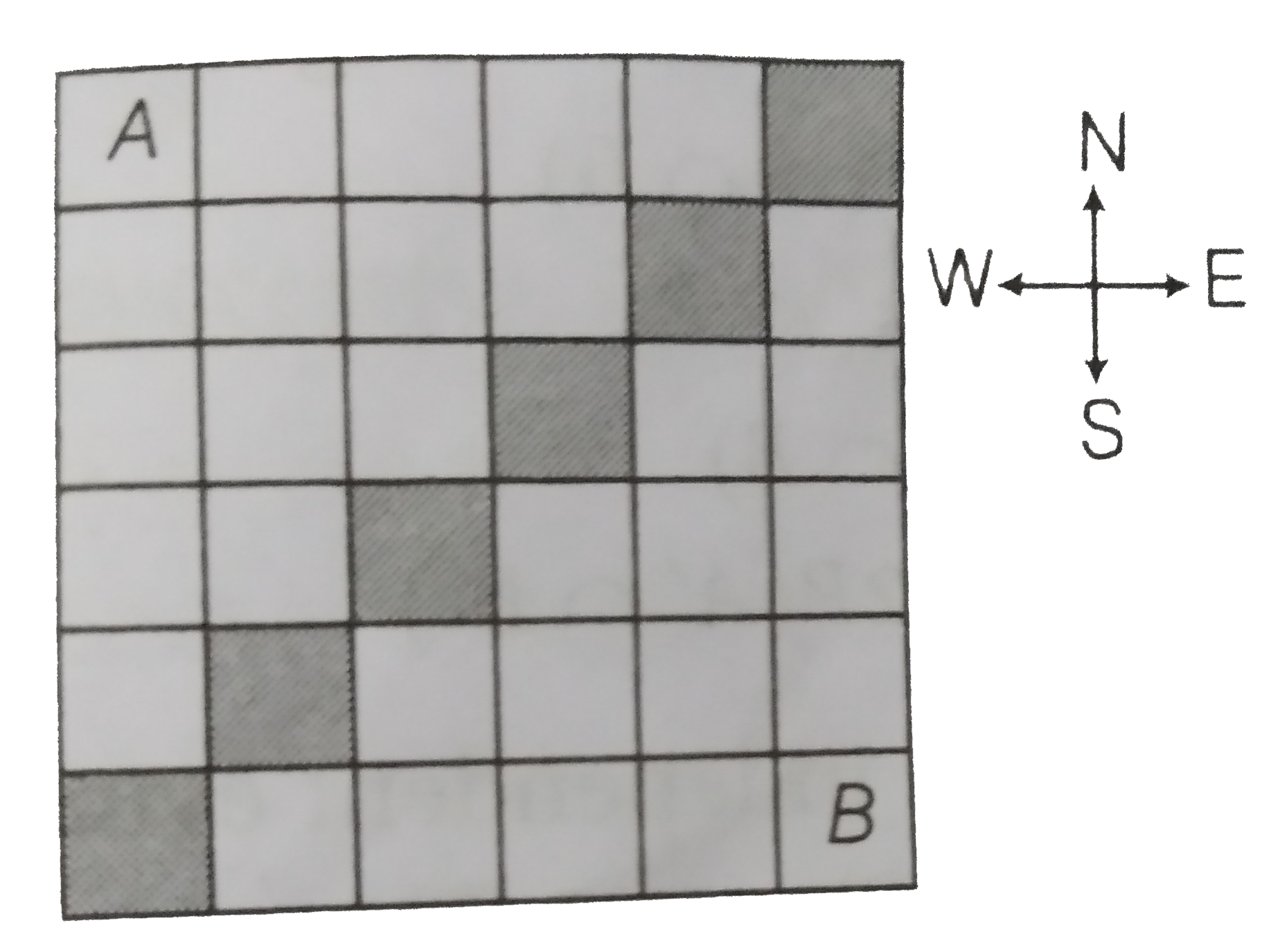
A and B are persons standing in corner square as shown in the figure. They start to move on same time with equal speed, of A can move in East or South diretion and B can move in North or West diretion. If in each step they reach in next square and their choice of direction are equlity. If it is given that A and B meet in shaded region, then the probability that they have met in the top most shaded square, is
View SolutionFor two given event A and B, P(A∩B) is
View SolutionThe probability of getting exactly two heads when tossing a coin three times is
View SolutionThere is a rectangular sheet of dimension (2m−1)×(2n−1), (where m>0,n>0) It has been divided into square of unit area by drawing line perpendicular to the sides. Find the number of rectangles having sides of odd unit length.
View SolutionLet O be the origin and let PQR be an arbitrary triangle. The point S is such that ¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OQ+¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OP+¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OR+¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OS Then the triangle PQR has S as its
View SolutionThe digits 1,2,3,.....9 are written in random order to form a nine digit number.Find the probability that this number is divisible by 11 .
View SolutionThe number of n digit number formed by using digits {1,2,3} such that if 1 appears, it appears even number of times, is
View SolutionIf the numbers 1,2,3….,n are arranged in a line at random what is the probability that the numbers 1 and 2 will appear next to each other?
View SolutionThe range of a random variable X={1,2,3,…………..) and the probabilities are given by
P(X=k)=3ckk!(k=1,2,3,………..) and c is a constant . Then c=
View SolutionA number n is chosen at random from
S = {1,2,3,…, 50} Let
A = { n∈S:n+50n>27}
B ={n∈S:n is a prime } and
C = {n∈S:n is a square } . Then , correct order of their probabilities is
View SolutionFor what value of k will the function f(x) =kx x=1,2,3 ...., n be a probability distribution of a random variable ?
View SolutionA number n is chosen at random from S = {1,2,3…….50}.
Then correct order of their probabilities is
View SolutionLet Sn=n∑k=1(−1)k−1.K2 for n≥1. Given that S2n=−n(2n+1) For n=1,2,3,⋯then Sn=
View SolutionA number n is chosen at random from S={1,2,3,…..50}. Let A={n∈S:n+50n>27}, B={n∈S:nis a prime} and C={n∈S:nis a square} Then correct order of their probability is
View Solution6 boys and 5 girls are arranged in a random order. The probability of arranging the girls as neighbours in specified order is
View SolutionThe numbers 1, 2, 3, ..., n are arrange in a random order. The probability that the digits 1, 2, 3, .., k(k<n) appear as neighbours in that order is
View Solution
