If α and β are roots of x2−(√1−cos2θ)x+θ=0, where 0<θ<π2. Then limθ→0+(1α+1β) is Solution in Punjabi
(a)1√2
(b)−√2
(c)√2
(d)None of these
The correct Answer is:C
Step by step video & image solution for If alpha and beta are roots of x^(2)-(sqrt(1-cos 2 theta))x+theta=0, where 0 lt theta lt (pi)/2. Then lim_(theta to 0^(+))(1/(alpha)+1/(beta)) is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LIMITSBOOK - ARIHANT MATHSCHAPTER - LIMITSEXERCISE - Exercise (More Than One Correct Option Type Questions)
15 Videos
- LIMITSBOOK - ARIHANT MATHSCHAPTER - LIMITSEXERCISE - Exercise (Passage Based Questions)
10 Videos
- LIMITSBOOK - ARIHANT MATHSCHAPTER - LIMITSEXERCISE - SOLVED EXAMPLES
1 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - ARIHANT MATHSCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
8 Videos
- LOGARITHM AND THEIR PROPERTIESBOOK - ARIHANT MATHSCHAPTER - LOGARITHM AND THEIR PROPERTIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
4 Videos
Similar Questions
If limx→∞4x(π4−tan−1(x+1x+2))=y2+4y+5 then y can be equal to
View SolutionIf limx→af(x)=1 and limx→ag(x)=∞ then limx→a{f(x)}g(x)=elimx→a(f(x)−1)g(x)
limx→0(sinxx)sinxx−sinx is equal to
View SolutionTo evaluate limx→a[f(x)], we must analyse the f(x) in right hand neighbourhood as well as in left hand neighbourhood of x=a. E.g. In case of continuous function, we may come across followign cases.
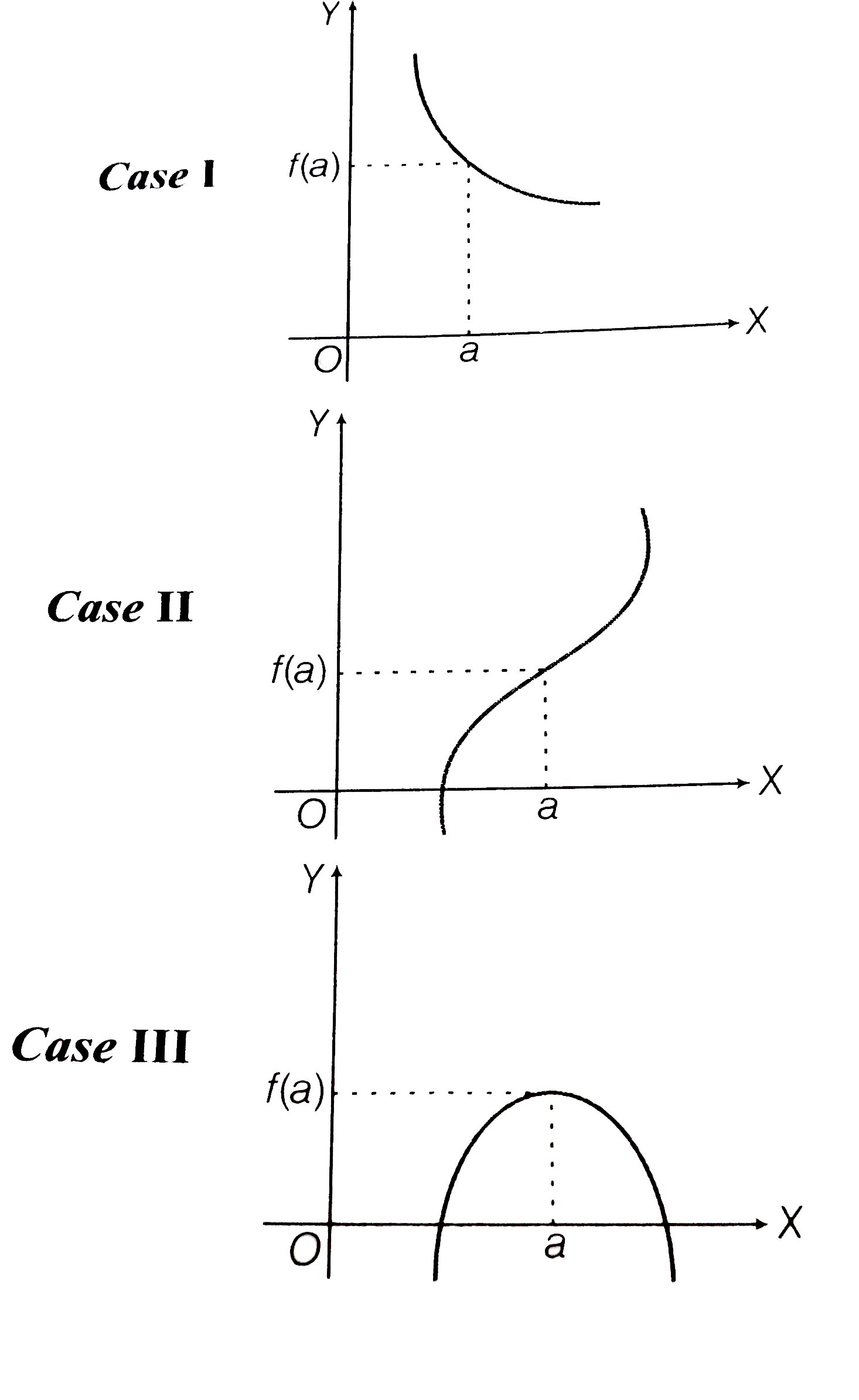
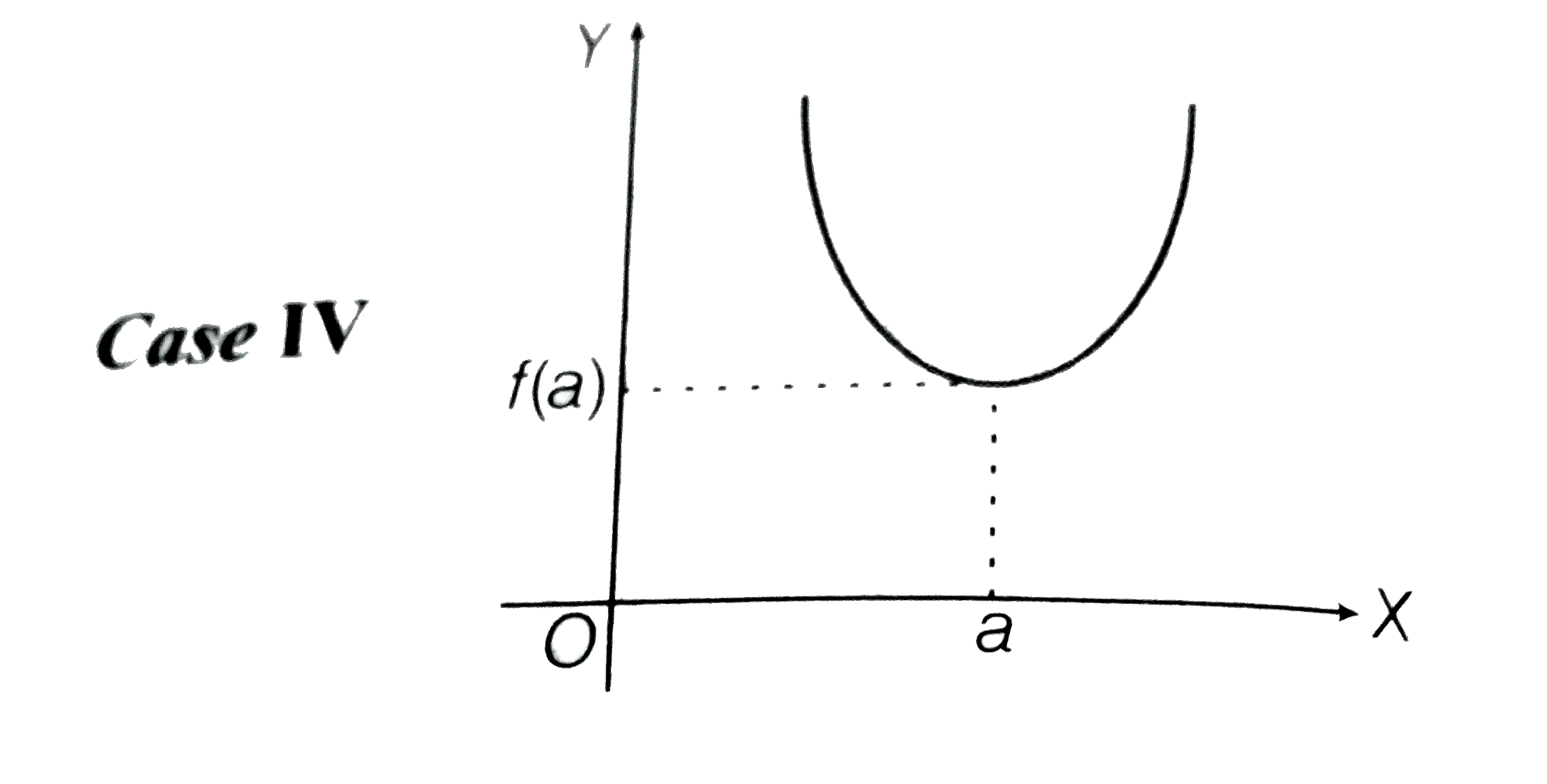
Iff(a) is an integer, the limit will exist in Case III and Case IV but not in Case I and Case II. If f(a) is not an integer, the limit exists in all the cases. ltbgt If f'(1)=−3 and limx→1[f(x)−12] does not exist, (where [.] denotes the greatest integer function), then
View SolutionIf α=3sin−1(611andβ=3cos−1(49), where the inverse trigonometric functions take only the principal values, then the correct options (s) is (are)
View SolutionIn Which quadrant Point (−2,−3) will be lie.
View SolutionIf alpha,beta are roots of z^(2)sin^(2)theta-z sin2 theta+1=0 where '0
View SolutionIf alpha and beta are the roots of the equation (1)/(2)x^(2)-sin2 theta x+cos2 theta=0, then the value of (1)/(alpha)+(1)/(beta) is:
View SolutionIf cos(θ−α)=aandsin(θ−β)=b(0<θ−α,θ−β<π/2), then prove that cos2(α−β)+2absin(α−β)=a2+b2
View SolutionIf 0<θ<π2andsinθ2=√x−12x, then the value of tanθ is-
View SolutionIf α,β are roots of the equation (1+cosθ)tan2x−λtanx=1−cosθ where θ∈(0,π2) and cos2(α+β)=151 then λ is :
View SolutionIf θ+ϕ=α, where 0<θ<π2,0<ϕ<π2andα is constant, find the minimum values of
cosecθ+cosecθ
View Solutionयदि 5cos2θ+2cos2(θ2)+1=0 –π<θ<π तब θ=
View SolutionLet α and β be the roots of the quadratic equation x2 sin θ−x(sinθcosθ+1)+cosθ=0(0<θ<45∘), and α<β.
Then Σ∞n=0(αn+(−1)nβn) is equal to
View SolutionIf cos(θ−α)=aandsin(θ−β)=b(0<θ−α,θ−β<π/2), then prove that cos2(α−β)+2absin(α−β)=a2+b2
View SolutionIf α,β are roots of the equation (1+cosθ)tan2x−λtanx=1−cosθ where θ∈(0,π2) and cos2(α+β)=151 then λ is :
View SolutionIf α+β and α−β are solutions of θ for the equation tan2θ−4tanθ=−1 where 0<α<π2 and 0<β<π2 then find α and β.
View Solution
