Let f(x)=⎧⎪⎨⎪⎩Ax−Bx≤12x2+3Ax+Bx∈(−1,1]4x>1
Statement I f(x) is continuous at all x if A=34,B=−14. Because
Statement II Polynomial function is always continuous.Solution in Punjabi
Statement I is correct, Statement II is also correct, Statement II is the correct explanation of Statement I
Statement I is correct, Statement II is also correct, Statement II is not the correct explanation of Statement I
Statement I is correct, Statement II is incorrect
Statement I is incorrect, Statement II is correct.
The correct Answer is:B
Step by step video & image solution for Let f(x) = {{:(Ax - B,x le 1),(2x^(2) + 3Ax + B,x in (-1, 1]),(4,x gt 1):} Statement I f(x) is continuous at all x if A = (3)/(4), B = - (1)/(4). Because Statement II Polynomial function is always continuous. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CONTINUITY AND DIFFERENTIABILITYBOOK - ARIHANT MATHSCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - EXERCISE 4
3 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - ARIHANT MATHSCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise (Passage Based Questions)
16 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - ARIHANT MATHSCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise (More Than One Correct Option Type Questions)
26 Videos
- COMPLEX NUMBERSBOOK - ARIHANT MATHSCHAPTER - COMPLEX NUMBERSEXERCISE - Complex Number Exercise 8
3 Videos
- COORDINATE SYSTEM AND COORDINATES BOOK - ARIHANT MATHSCHAPTER - COORDINATE SYSTEM AND COORDINATES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
7 Videos
Similar Questions
A man leaves his home early in the morning to have a walk. He arrives at a junction of roads A and B as shown in figure. He takes the following steps in later journies :
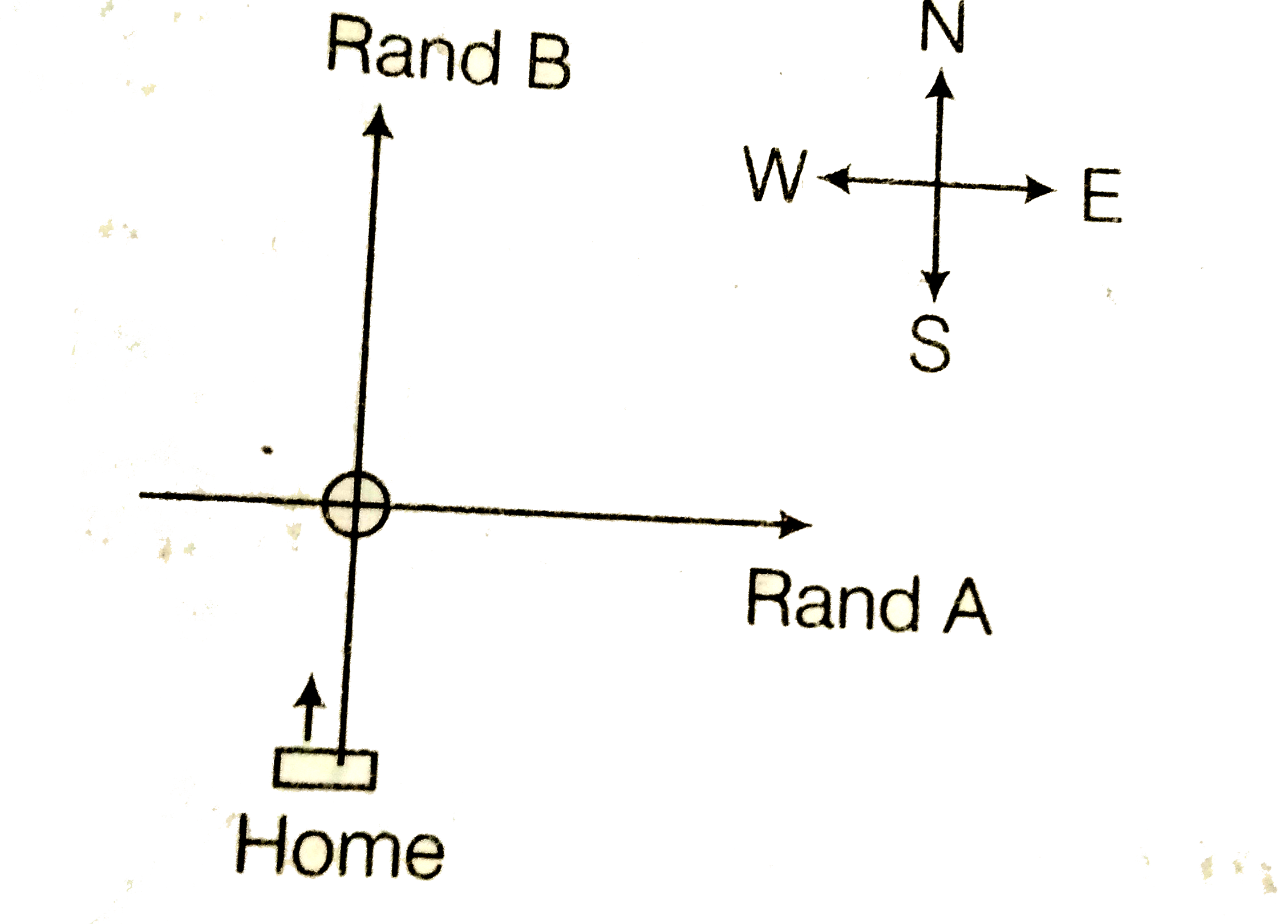
(i) 1 km in North direction.
(ii) Changes direction and moves in North-East direction for 2√2 km.
(iii) Changes direction and moves Southwards for distance of 2 km.
(iv) Finally he changes the direction and moves in South-East direction to reach road A again.
Now if roads A and B are taken as X-axis and y-axis ,then visible point representing the graph of y=f(x)
Then the value of x at which function f(x) is discontinous
View SolutionLet f be a function that is differentiable everywhere and that has the follwong properties :
(i) f(x)>0
(ii) f'(0)=−1
(iii) f(−x)=1f(x)andf(x+h)=f(x).f(h)
A standard result is f'(x)f(x)dx=log|f(x)|+C
The range fo the function Δ=f(|x|) is
View SolutionFunction whose jump (non-negative difference of LHL and RHL) of discontinuity is greater than or equal to one. Is/are
View SolutionFind the modules of 2+3ι
View SolutionIf a vertex of a triangle is (1, 1) and the mid-points of two side through this vertex are (-1, 2) and (3, 2), then centroid of the triangle is
View SolutionIf the function f(x)=⎧⎪⎨⎪⎩3ax+b, x>111, x=15ax−2b, x<1
continuous at x= 1 then ( a, b) =?
View SolutionIf f(x)={ax+1,x≤π2sinx+b,x>π2 is continuous, then
View Solutionयदि फलन f(x), जहाँ f(x)=⎧⎪⎨⎪⎩3ax+b,यदिx>111,यदिx=15ax−2b,यदिx<1x=1 पर संतत हो, तो a और b का मान ज्ञात कीजिए ।
View SolutionLet f(x)=⎧⎪⎨⎪⎩Ax−Bx≤12x2+3Ax+Bx∈(−1,1]4x>1
Statement I f(x) is continuous at all x if A=34,B=−14. Because
Statement II Polynomial function is always continuous.
View SolutionIf f(x) =x+1, if x≤1=3+ax2, if x>1 is continuous on R , then a = ………
View SolutionIf f(x) =x+a, if x<0=x, if 0≤x≤1=b−x, if x>1 is continuous in [−2,2] , then a+b is
View SolutionDiscuss the continuity of the function f defined by {x+4 if x≤1x−4 if x>1.
View SolutionIs the function f defined by
f(x)={x if x≤13 if x>1
continuous at x=0? At x=1? At x=2?.
View SolutionLet f(x)={x+1,x≤13−ax2,x>1 The value of a so that f is continuous is
View Solutionमाना f(x)=⎧⎪⎨⎪⎩Ax−Bx≥−12x2+3Ax+Bx∈(−1,1)4x>1
कथन-I : f (x) सभी x के लिए संतत् है, यदि A=34,B=−14 है। क्योंकि
कथन-II : बहुपद फलन सदैव संतत् होते हैं।
View SolutionLet f(x)=⎧⎪⎨⎪⎩Ax−Bx≤12x2+3Ax+Bx∈(−1,1]4x>1
Statement I f(x) is continuous at all x if A=34,B=−14. Because
Statement II Polynomial function is always continuous.
View Solution
