Two sides of a triangle are given by the roots of the equation x2−2√3x+2=0. The angle between the sides is π3. Find the perimeter of Δ. Solution in Punjabi
The correct Answer is:=a+b+c=2√3+√6
Step by step video & image solution for Two sides of a triangle are given by the roots of the equation x^(2) -2sqrt3 x+2 =0. The angle between the sides is (pi)/(3). Find the perimeter of Delta. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - SOLVED EXAMPLES
1 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise For Sesssion 1
20 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHSCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
52 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHSCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
Similar Questions
Match the statements of Column I with values of Column II.

View SolutionIn the given figure,if AB=AC,∠BAD=30∘andAE=AD, then x is equal to
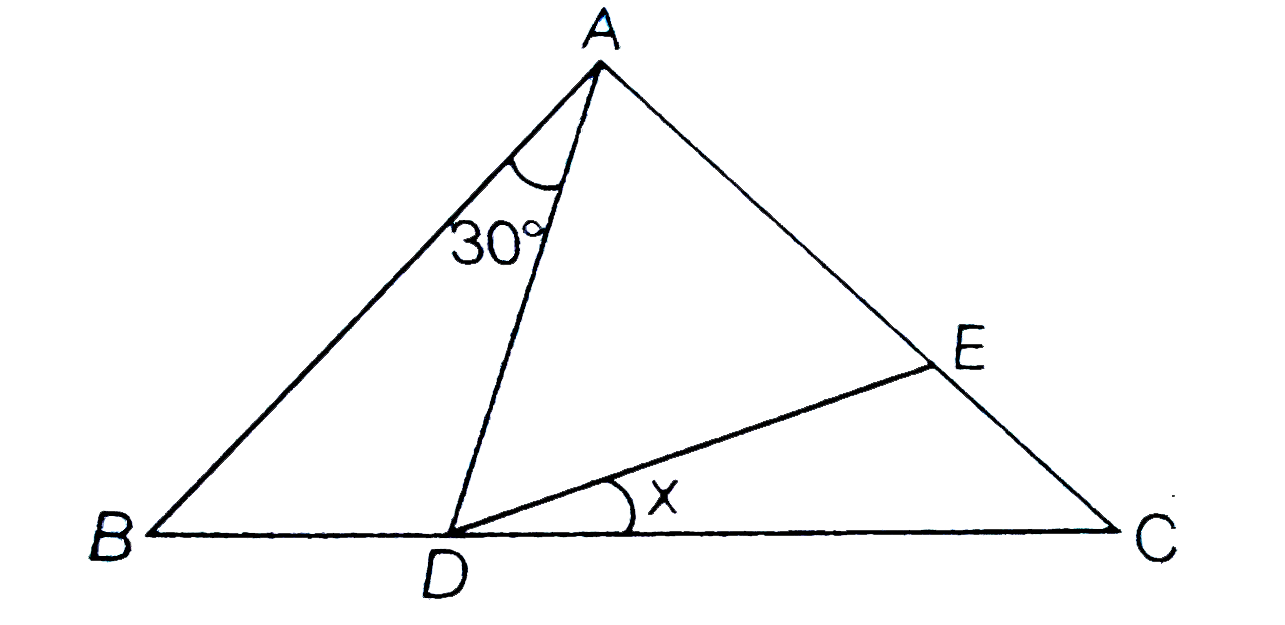
View SolutionLet O be the origin and let PQR be an arbitrary triangle. The point S is such that ¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OQ+¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OP+¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OR+¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OS Then the triangle PQR has S as its
View SolutionLet a,b,c be in A.P. and |a|<1,|b|<1|c|<1.ifx=1+a+a2+....to ∞,y=1+b+b2+....to ∞and,z=1+c+c2+...to ∞, then x,y,z are in
View SolutionTwo sides of a triangle are given by the roots of the equation x2−5x+6=0 and the angle between the sides is π3.Then the perimeter of the triangle is
View SolutionThe roots of x^(2)2sqrt(3)x+2=0 represent twoa triangle.If the angle between them is (pi)/(3). then the perimeter of the triangle is
View SolutionIf two sides of a triangle are roots of the equation x2−7x+8=0 and the angle between these sides is 60∘ then the product of inradius and circumradius of the triangle is
View SolutionTwo sides of a triangle are given by the roots of the equation x2−5x+6=0 and the angle between the sides is π3. Then the perimeter of the triangle is-
View SolutionTwo sides of a triangle are given by the roots of the equation x2−2√3x+2=0. The angle between the sides is π/3. The perimeter of the triangle is
View SolutionThe roots of x2−2√3x+2=0 represent two sides of a triangle. IF the angle between them is π/3, then the perimeter of the triangle is
View SolutionIf two sides of a triangle are the roots of the equation 4x2−(2√6)x+1=0 and the included angle is 60∘, then the third side is
View SolutionTwo sides of a triangle are given by the roots of the equation x2−5x+6=0 and the angle between the sides is π/2. Then the perimeter of the triangle is
View Solutionx2−5x+6=0 সমীকরণের বীজ দুটি হল একটি ত্রিভুজের দুটি বাহু এবং ওই বাহু দুটির অন্তর্ভূত কোণ π3,তাহলে , ত্রিভুজটির পরিসীমা হবে-
View SolutionIf two sides of a triangle are roots of the equation x2−7x+8=0 and the angle between these sides is 60∘ then the product of inradius and circumradius of the triangle is
View SolutionTwo sides of a triangle is given. If the area of a triangle is maximum, then show that the angle between the two sides is π2.
View SolutionTwo sides of a triangles are given by the roots of the equation x2−3(√2+1)x+9√2=0and the angle between the sides is 45∘ , then the triangle is
View Solution
