A particular substance is being cooled by a stream of cold air (temperature of the air is constant and is 5∘C) where rate of cooling is directly proportional to square of difference of temperature of the substance and the air.
If the substance is cooled from 40∘C to 30∘C in 15 min and temperature after 1 hour is T∘C, then find the value of [T]/2, where [.] represents the greatest integer function.Solution in Punjabi
The correct Answer is:9
Step by step video & image solution for A particular substance is being cooled by a stream of cold air (temperature of the air is constant and is 5^(@)C) where rate of cooling is directly proportional to square of difference of temperature of the substance and the air. If the substance is cooled from 40^(@)C to 30^(@)C in 15 min and temperature after 1 hour is T^(@)C, then find the value of [T]//2, where [.] represents the greatest integer function. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHSCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - MAXIMA AND MINIMA EXERCISE 6
2 Videos
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHSCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
31 Videos
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHSCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - MAXIMA AND MINIMA EXERCISE 5
4 Videos
- MATRICES BOOK - ARIHANT MATHSCHAPTER - MATRICES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
49 Videos
- PAIR OF STRAIGHT LINES BOOK - ARIHANT MATHSCHAPTER - PAIR OF STRAIGHT LINES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
2 Videos
Similar Questions
The figure shows a right triangle with its hypotenuse OB along the y-axis and its vertex A on the parabola y=x2.
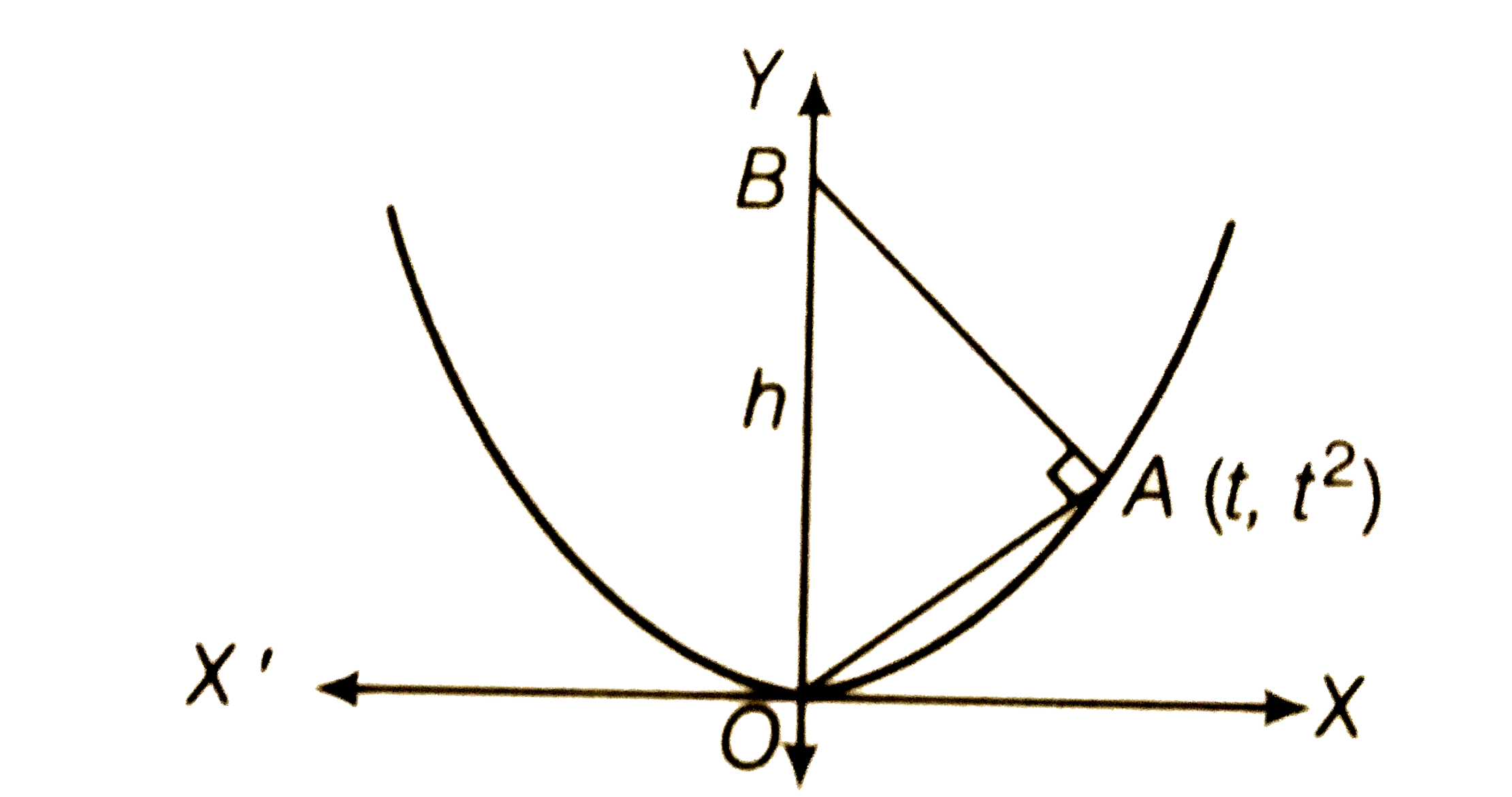
Let h represents the length of the hypotenuse which depends on the x-coordinate of the point A. The value of limt→o(h) is equal to
View SolutionThe least value of α∈R for which 4ax2+1x≥1, for all x>0, is
View SolutionEvaluate ∫(sinx+2cosx)dx.
View SolutionLet A=⎡⎢⎣1000110−24⎤⎥⎦,I=⎡⎢⎣100010001⎤⎥⎦andA−1=[16(A2+cA+dI)] Then value of candd are (a) (−6,−11) (b) (6,11) (c) (−6,11) (d) (6,−11)
View SolutionIf the pair of lines ax2+2(a+b)xy+by2=0 lie along diameters of a circle and divide the circle into four sectors such that the area of one of the sectors is thrice the area of another sector then
View SolutionThe rate at which a substance cools in moving air is proportional to the difference between the temperatures of the substance and that of the air. If the temperature of the air is 290 K and the substance cools from 370 K to 330 K in 10 min, when will the temperature be 295 K?
View SolutionThe temperature T of a cooling object drops at a rate proportional to the difference T−S where S is constant temperature of surrounding medium. If initially T=150∘C; find the temperature of the cooling object at any time t.
View SolutionA body cools from 50^(@)C to 40^(@)C in 5 min. The surroundings temperature is 20^(@)C . In what further times (in minutes) will it cool to 30^(@)C ?
View Solutionउस अवकल समीकरण की कोटि तथा घात जिसका हल c(y+c)2=x3 है, होगी (जहाँ c स्वेच्छ अचर है)
न्यूटन के शीतलता के नियम के अनुसार ''किसी पदार्थ के वायु में ठण्डा होने की दर पदार्थ तथा वायु के तापान्तर के अनुक्रमानुपाती होता है।''
यद वायु का ताप 290 K हो, तो न्यूटन का शीतलता नियम dTdt=−k(T−290) होगा, जहाँ k>0 अचर है तथा T पदार्थ का ताप है।
View SolutionAccording to Newton's law, rate of cooling is proportional to the difference between the temperature of the body and the temperature of the air. If the temperature of the air is 20∘C and body cools for 20 min from 100∘C to 60∘C then the time it will take for it temperature to drop to 30∘ is
View SolutionAccording to Newton's law of cooling, the rate of cooling of a body in air is proportional to the difference between the temperature of the body and the temperature of the surrounding air. If the air temperature is 20∘C and the body cools for 20 minutes from 140∘C→80∘C, then the temperature will be 50∘C in
View SolutionA body cools at the rate of 3∘C/min when its temperature is 50∘C. If the temperature of surroundings is 25∘C then the rate of cooling of the body at 40∘C is
View Solutionन्यूटन का शीतलन नियम क्या है ? एक वस्तु T∘1C से T∘2C तक t सेकंड में शीतल होती है | यदि वातावरण का तापT∘∘C हो तो शीतलन की दर तथा तापांतर में संबंध दर्शाने वाला ज्ञात कीजिए |
View SolutionThe temperature T of a cooling object drops at a rate proportional to the difference T-S where S is constant temperature of surrounding medium if initially T= 150∘ C find the temperature of the cooling object at any time t
View SolutionThe rate at which a substance cools in moving air is proportional to the difference between the temperatures of the substance and that of the air. If the temperature of the air is 290 K and the substance cools from 370 K to 330 K in 10 min, when will the temperature be 295 K?
View SolutionThe rate at which a substance cools in moving air is proportional to the difference between the temperatures of the substance and that of the air. If the temperature of the air is 290 K and the substance cools from 370 K to 330 K in 10 min, when will the temperature be 295 K?
View Solution
