Number of critical points of the function.
f(x)=23√x3−x2+∫x1(12+12cos2t−√t) dt which lie in the interval [−2π,2π] is………. .Solution in Punjabi
The correct Answer is:4
Step by step video & image solution for Number of critical points of the function. f(x)=(2)/(3)sqrt(x^(3))-(x)/(2)+int_(1)^(x)((1)/(2)+(1)/(2)cos2t-sqrt(t)) dt which lie in the interval [-2pi,2pi] is………. . by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHSCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - MAXIMA AND MINIMA EXERCISE 6
2 Videos
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHSCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
31 Videos
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHSCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - MAXIMA AND MINIMA EXERCISE 5
4 Videos
- MATRICES BOOK - ARIHANT MATHSCHAPTER - MATRICES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
49 Videos
- PAIR OF STRAIGHT LINES BOOK - ARIHANT MATHSCHAPTER - PAIR OF STRAIGHT LINES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
2 Videos
Similar Questions
The figure shows a right triangle with its hypotenuse OB along the y-axis and its vertex A on the parabola y=x2.
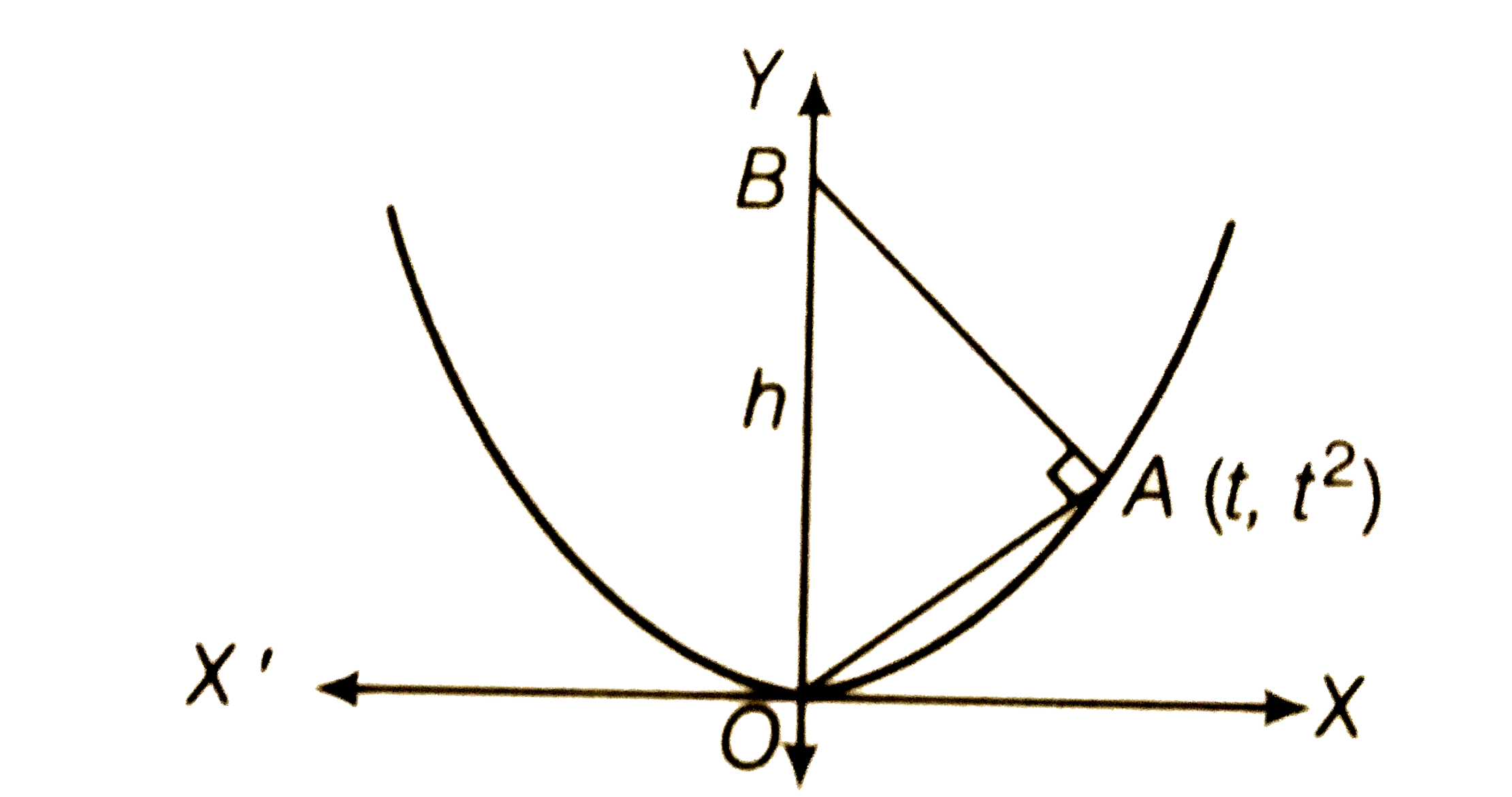
Let h represents the length of the hypotenuse which depends on the x-coordinate of the point A. The value of limt→o(h) is equal to
View SolutionThe least value of α∈R for which 4ax2+1x≥1, for all x>0, is
View SolutionEvaluate ∫(sinx+2cosx)dx.
View SolutionLet A=⎡⎢⎣1000110−24⎤⎥⎦,I=⎡⎢⎣100010001⎤⎥⎦andA−1=[16(A2+cA+dI)] Then value of candd are (a) (−6,−11) (b) (6,11) (c) (−6,11) (d) (6,−11)
View SolutionIf the pair of lines ax2+2(a+b)xy+by2=0 lie along diameters of a circle and divide the circle into four sectors such that the area of one of the sectors is thrice the area of another sector then
View SolutionIf t=2√2−(1+√3)√3−1 and f(x)=2x1−x2 g(x)=3x−x31−3x2 then ddt{f(g(t))}=
View SolutionFind the equation of tangent to y=∫x3x2dt√1+t2atx=1.
View SolutionThe equation of tangent to the curve y=∫x3x2dt√1+t2 at x=1 is √3x+1=y (b) √2y+1=x √3x+y=1 (d) √2y=x
View SolutionFind the critical points of the function f(x)=(2)/(3)sqrt(x^(3))-(x)/(2)+int_(1)^(x)((1)/(2)+(1)/(2)cos2t-t^((1)/(2)))dt
View Solutionequation of tangent to y=int_(x^(2)rarr x^(3))(dt)/(sqrt(1+t^(2))) at x=1 is:
View SolutionThe number of critical points of the function f(x)=int_(0)^(x)e^(t)(t-1)(t-2)(t-3)dt
View Solutionf(x)=∫x20 (sin−1√t)2√t dt
View SolutionThe value of
sin2x∫0sin−1√tdt+cos2x∫0cos−1√tdt, is
View SolutionThe interval in which the function
f(x)=∫x0(tt+2−1t)dtwill be non- increasing is
View SolutionIf f(x)=∫x0{f(t)}−1dt, and ∫10{f(t}−1dt=√2, then f(x)=
View SolutionIf f(x)=∫x21x2cos√tdt, then f'(1)=
View Solution
