Let f:R→R be a continuous function such that
f(x)−2f(x2)+f(x4)=x2.
f(3) is equal toSolution in Punjabi
all four distinct roots
three distinct real roots
two real and two imaginary
all four imaginary roots
The correct Answer is:C
Step by step video & image solution for Let f:R rarr R be a continuous function such that f(x)-2f(x/2)+f(x/4)=x^(2). f(3) is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- INDEFINITE INTEGRAL BOOK - ARIHANT MATHSCHAPTER - INDEFINITE INTEGRAL EXERCISE - Exercise 5 ( Matching Type Questions )
2 Videos
- INDEFINITE INTEGRAL BOOK - ARIHANT MATHSCHAPTER - INDEFINITE INTEGRAL EXERCISE - Exercise (Single Integer Answer Type Questions)
9 Videos
- INDEFINITE INTEGRAL BOOK - ARIHANT MATHSCHAPTER - INDEFINITE INTEGRAL EXERCISE - Exercise (Statement I And Ii Type Questions)
5 Videos
- HYPERBOLABOOK - ARIHANT MATHSCHAPTER - HYPERBOLAEXERCISE - Hyperbola Exercise 11 : Questions Asked in Previous 13 Years Exams
3 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - ARIHANT MATHSCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
8 Videos
Similar Questions
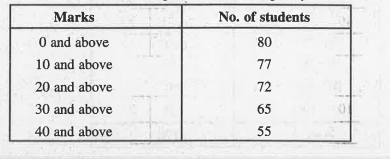
Find the mean marks of the following cummulative frequency table :

View SolutionIf ∫(2x+3)dxx(x+1)(x+2)(x+3)+1=C−1f(x) where f(x) is of the form of ax2+bx+c, then(a+b+c) equals to …….
View SolutionSolve dydx+yx=logx.
View Solutionwhich term of the following sequence : 312,3,332.....is 81?
View SolutionIf α=3sin−1(611andβ=3cos−1(49), where the inverse trigonometric functions take only the principal values, then the correct options (s) is (are)
View SolutionLet f:R rarr R be a function defined by f(x)=x^(3)+x^(2)+3x+sin x. Then f is
View Solutionf:R rarr R;f(x) is continuous function satisfying f(x)+f(x^(2))=2AA x in R, then f(x) is
View Solutionf:R rarr R;f(x) is continuous function satisfying f(x)+f(x^(2))=2AA x in R, then f(x) is
View SolutionLet f:R→R be a continuous function such that f(x)−2f(x2)+f(x4)=x2 Now answer the following
View SolutionLet f:R rarr R be a continuous onto function satisfying f(x)+f(-x)=0AA x in R, If f(-3)=2 and f(5)=4 in [-5,5], then the equation f(x)=0 has
View SolutionLet f:R→R be a function such that f(x)=x3+x2f'(1)+xf''(2)+f'''(3),x∈R. Then f(2) equals
View SolutionLet f:R→R be a continuous function such that
f(x)−2f(x2)+f(x4)=x2.
f(3) is equal to
View SolutionLet f:R→R be a continuous function such that
f(x)−2f(x2)+f(x4)=x2.
The equation f(x)-x-f(0)=0 have exactly
View SolutionLet f:R→R be a continuous function such that
f(x)−2f(x2)+f(x4)=x2.
f'(0) is equal to
View SolutionLet f:R→R be a continuous function such that f(x)−2f(x2)+f(x4)=x2. Now answer the following
f(3)=
View SolutionLet f:R→R be a continuous function such that
f(x)−2f(x2)+f(x4)=x2.
f(3) is equal to
View Solution
