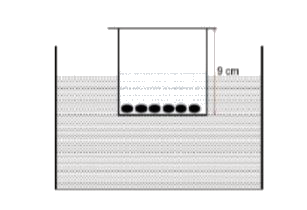
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to

A

6

B

10

C

16

D

24

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:B

Let mass of each coin be m
∴ Location of center of mass after N coins are kept on lid from bottom of container is
40 m × 0 + N m × 9 ( 40 + N ) = 9 N 40 + N c m
Also height of submerged portion after keeping N coins on lid will be
3 ( 40 + N ) 40 c m
∴ Equilibrium will just be stable if
3 ( 40 + N ) 40 , 2 = 9 N ( 40 + N )
⇒ 3 N 2 - 480 N + 4800 = 0 ⇒ N = 10.72

Updated on:21/07/2023
|
Class 12PHYSICSQUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • A wooden cube is found to float in water with ½cm of its vertical side above the water. On keeping a weight of 50 gm over its top, it is just submerged in the water. The specific gravity of wood is

    A0.8
    B0.9
    C0.85
    D0.95

  • In order that a floating object be in a stable equilibrium its centre of buoyancy should be

    Avertical below its centre of gravity
    Bhorizontally inline with its centre of gravity
    Cvertically above its centre of gravity
    Dmay be anywhere

  • A rectangular block is 5cm×5cm×10cm in size. The block is floating in water with 5 cm side vertical. If it floats with 10 cm side vertical, what change will occur in the level of water?

    ANo change
    BIt will rise
    CIt will fall
    DIt may rise or fall depending on the density of block