A particle of charge q=4μC and mass m = 10 mg starts moving from the origin under the action of an electric field →E=4ˆi and magnetic field →B=(0.2T)ˆk. Its velocity at (x, 3, 0) is (4→i+3→j). The value of x is -
11516m
12516m
13516m
14516m
The correct Answer is:B
To solve the problem step by step, we need to analyze the forces acting on the charged particle and apply the work-energy theorem.
Step 1: Identify the Given Information
- Charge of the particle, q=4μC=4×10−6C
- Mass of the particle, m=10mg=10×10−3g=10×10−6kg
- Electric field, →E=4^iN/C
- Magnetic field, →B=0.2^kT
- Velocity at point (x,3,0), →v=4^i+3^j
Step 2: Calculate the Resultant Velocity
The resultant velocity v can be calculated using the components:
v=√(4)2+(3)2=√16+9=√25=5m/s
Step 3: Apply the Work-Energy Theorem
The work done by all forces is equal to the change in kinetic energy:
Wtotal=ΔKE=KEfinal−KEinitial
Initially, the particle is at rest, so:
KEinitial=0
The final kinetic energy is:
KEfinal=12mv2
Substituting the values:
KEfinal=12×(10×10−6)×(5)2=12×10×10−6×25=125×10−6J=1.25×10−4J
Step 4: Calculate the Work Done by the Electric Force
The work done by the electric force is given by:
WE=FE⋅d=(qE)⋅x
Where:
- FE=qE
- E=4N/C
- x is the displacement in the ^i direction.
Calculating the electric force:
FE=qE=(4×10−6)×(4)=16×10−6N
Thus, the work done by the electric field is:
WE=16×10−6⋅x
Step 5: Set Up the Equation
Setting the work done equal to the change in kinetic energy:
16×10−6⋅x=1.25×10−4
Step 6: Solve for x
Rearranging the equation:
x=1.25×10−416×10−6=1.2516×102=12516m
Final Answer
Thus, the value of x is:
x=12516m
- Charge of the particle, q=4μC=4×10−6C
- Mass of the particle, m=10mg=10×10−3g=10×10−6kg
- Electric field, →E=4^iN/C
- Magnetic field, →B=0.2^kT
- Velocity at point (x,3,0), →v=4^i+3^j
The resultant velocity v can be calculated using the components:
v=√(4)2+(3)2=√16+9=√25=5m/s
The work done by all forces is equal to the change in kinetic energy:
Wtotal=ΔKE=KEfinal−KEinitial
Initially, the particle is at rest, so:
KEinitial=0
The final kinetic energy is:
KEfinal=12mv2
Substituting the values:
KEfinal=12×(10×10−6)×(5)2=12×10×10−6×25=125×10−6J=1.25×10−4J
The work done by the electric force is given by:
WE=FE⋅d=(qE)⋅x
Where:
- FE=qE
- E=4N/C
- x is the displacement in the ^i direction.
FE=qE=(4×10−6)×(4)=16×10−6N
Thus, the work done by the electric field is:
WE=16×10−6⋅x
Setting the work done equal to the change in kinetic energy:
16×10−6⋅x=1.25×10−4
Rearranging the equation:
x=1.25×10−416×10−6=1.2516×102=12516m
Thus, the value of x is:
x=12516m
Similar Questions
A particle of charge q and mass m starts moving from the origin under the action of an electric field →E=E0ˆi and →B=B0ˆi with velocity →v=v0ˆj. The speed of the particle will become 2v0 after a time
View SolutionA particle of charge q and mass m starts moving from the origin with a velocity →v.eqv0ˆj under the action of an electric field →E=E5¯i and magnetic field →B=B0→i The speed of the particle will become 2v0 aftet a time t=√xmw0qE0. Find the value of x.
View Solution
Knowledge Check
A particle of charge q, mass starts moving from origin under the action of an electric field →E=E0ˆi and magnetic field →B=B0ˆk . Its velocity at (x,0,0) is v0(6ˆi+8ˆj). The value of is
A25mv20qE0B100mv20qB0C50mv20qE0D14mv20qE0A particle of charge q, mass starts moving from origin under the action of an electric field →E=E0ˆi and magnetic field →B=B0ˆk . Its velocity at (x,0,0) is v0(6ˆi+8ˆj). The value of is
A25mv20qE0B100mv20qB0C50mV20qE0D14mv20qE0A particle of specific charge (charge/mass) α starts moving from the origin under the action of an electric field →E=E0ˆi and magnetic field
→B=B0ˆk Its velocity at (x0,y0.0) is (4ˆi+3ˆj) The value of x0 is .A132αE0E0B162αE0B0C252αE0D5α2B0
A charged particle of charge e and mass m is moving in an electric field →E and magnetic field →B. Constant dimensionless quantities and quantities of dimention [T]−1.
View SolutionA particle of charge q and mass m starts moving from the origin under the action of an electric field →E=E0ˆiand→B=B0ˆi with a velocity →v=v0ˆj. The speed of the particle will become 2v0 after a time.
View SolutionA particle of charge =1μC and mass m=1 gm starts moving from origin at t = 0 under an electric field of 103N/C along x-axis and magnetic field of 10 tesla along the same axis with the velocity of →v=20ˆj m/sec as shown, the speed of the particle at the time of 20√3sec will be :
View SolutionThe electric field acts positive x-axis. A charged particle of charge q and mass m is released from origin and moves with velocity →v=v0ˆj under the action of electric field and magnetic field, →B=B0ˆi. The velocity of particle becomes 2v0 after time √3mv0√2qE0. find the electric field:
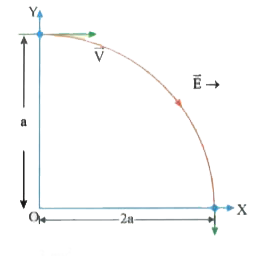
View SolutionA particle of charge +q and mass m moving under the influnce of a uniform electric field Eˆiand a uniform magnetic field Bˆk follows trajectory from P to Q as shown in figure. The velocities at P and Q vˆi and −2vˆj respectively. Which of the following statement(s) is/are correct
View Solution
