In a regiment the ratio between the number of officers to soldiers was 3:31 before battle . In a battle 6 officers and 22 soldiers were killed and the ratio becomes 1:13 , the number of officers in the regiment before battle was
31
38
21
18
To solve the problem, we will follow these steps:
1. Define Variables:
Let the number of officers be O and the number of soldiers be S.
2. Set Up the Initial Ratio:
According to the problem, the ratio of officers to soldiers before the battle is given as 3:31. This can be expressed as:
OS=331
From this, we can express O in terms of S:
O=331S
3. Account for Losses in Battle:
After the battle, 6 officers and 22 soldiers were killed. Therefore, the number of officers and soldiers after the battle will be:
O′=O−6
S′=S−22
4. Set Up the New Ratio:
After the battle, the ratio of officers to soldiers changes to 1:13. This can be expressed as:
O′S′=113
Substituting the expressions for O′ and S′:
O−6S−22=113
5. Cross Multiply to Solve for O and S:
Cross multiplying gives:
13(O−6)=S−22
Expanding this:
13O−78=S−22
Rearranging gives:
S=13O−56
6. Substitute S in Terms of O:
Now we have two equations:
1. O=331S
2. S=13O−56
Substitute the expression for S from the first equation into the second:
S=13(331S)−56
7. Solve for S:
Rearranging gives:
S+56=3931S
Multiplying through by 31 to eliminate the fraction:
31S+1736=39S
Rearranging gives:
39S−31S=1736
8S=1736
Dividing by 8:
S=217
8. Find O:
Now substitute S back into the equation for O:
O=331×217
Calculating gives:
O=65131=21
Thus, the number of officers in the regiment before the battle was 21.
Let the number of officers be O and the number of soldiers be S.
According to the problem, the ratio of officers to soldiers before the battle is given as 3:31. This can be expressed as:
OS=331
From this, we can express O in terms of S:
O=331S
After the battle, 6 officers and 22 soldiers were killed. Therefore, the number of officers and soldiers after the battle will be:
O′=O−6
S′=S−22
After the battle, the ratio of officers to soldiers changes to 1:13. This can be expressed as:
O′S′=113
Substituting the expressions for O′ and S′:
O−6S−22=113
Cross multiplying gives:
13(O−6)=S−22
Expanding this:
13O−78=S−22
Rearranging gives:
S=13O−56
Now we have two equations:
1. O=331S
2. S=13O−56
S=13(331S)−56
Rearranging gives:
S+56=3931S
Multiplying through by 31 to eliminate the fraction:
31S+1736=39S
Rearranging gives:
39S−31S=1736
8S=1736
Dividing by 8:
S=217
Now substitute S back into the equation for O:
O=331×217
Calculating gives:
O=65131=21
Topper's Solved these Questions
Similar Questions
In a regiment the ratio between the number of officers to soldiers was 3:31 before battle. In a battle 6 officers and 22 soldiers were killed and the ratio became 1:13, the number of officers in the regiment before battle was:
View Solution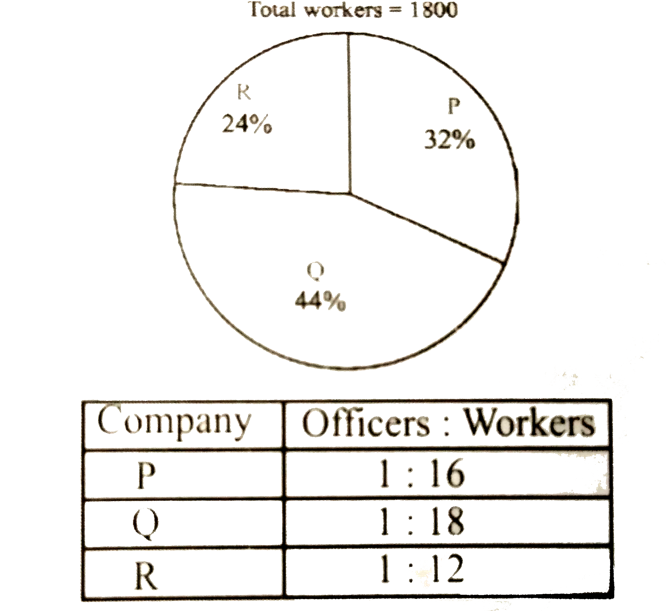
Find the ratio between total number of workers in company P and R together to total number of officers in company P and R together ?
View Solution
Knowledge Check
In a regiment the ratio between the number of officers to soldiers was 3:31 before battle . In a battle 6 officers and 22 soldiers were killed and the ratio becomes 1:13 , the number of officers in the regiment before battle was
A31B38C21D28What was the respective ratio between the number of soldiers recruited for airforce in the year 2005 and the number of soldiers recruited in army in the year 2009?
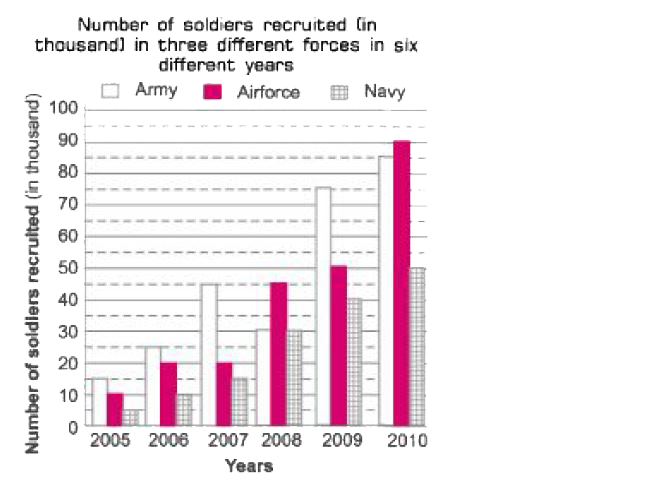 A2:15B5:13C2:17D15:4
A2:15B5:13C2:17D15:4Before a battle there were the ratio of captains to soldiers was 2 : 7. During the war 25 captains and 100 soldiers were martyred. The new ratio of captains to soldiers became 3 : 10. What is the number of soldiers after the war?
A250B200C150D100
Two numbers are in the ratio of 1:3. If 5 is added to both the numbers, the ratio becomes 1:2 Find the numbers.
View SolutionBefore a battle there were the ratio of captains to soldiers was 2: 7. During the war 25 captains and 100 soldiers were martyred. The new ratio of captions to soldiers became 3 : 10. What is the number of soldiers after the war?
युद्ध से पहले कैप्टन और सिपाहियों का अनुपात 2:7 था। युद्ध के दौरान और 25 कैप्टन और 100 सिपाही शहीद हुए। कैप्टन और सिपाहियों का नया अनुपात 3 : 10 हो गय।॥ युद्ध के बाद सिपाहियों की संख्या क्या होगी?
View SolutionTwo numbers are in the ratio 2 : 3. If 2 is subtracted from the first and 2 is added to the second, the ratio becomes 1:2. The sum of the numbers is :
View SolutionThe ratio of two numbers is 2:3. If 20 is subtracted by each number, the ratio becomes 1:2 . The numbers are -
View SolutionTwo numbers are in the ratio 2:3. If 2 is subtracted from the first and 2 is added to the second, the ratio becomes 1:2. The sum of the numbers is :
View Solution
