When ticket prices to a water park are in creased in the ratio 11:12 then the number of daily visitors to the park fall in the ratio 8:7 . If the daily revenues before the increase in ticket prices was Rs. 1,76,000 then find the daily revenues after the increase in ticket price .
264000
112000
192000
168000
To solve the problem step-by-step, we will use the information given about the ratios of ticket prices and the number of visitors, along with the initial revenue.
Step 1: Understand the Ratios
The ticket prices increase in the ratio of 11:12, and the number of visitors decreases in the ratio of 8:7.
Step 2: Define Variables
Let the original price of a ticket be 11x and the original number of visitors be 8y.
Step 3: Calculate Original Revenue
The original revenue can be calculated using the formula:
Revenue=Price×Number of Visitors
So, the original revenue is:
Original Revenue=(11x)×(8y)=88xy
We know from the problem that this original revenue is Rs. 1,76,000:
88xy=176000
Step 4: Find the Value of xy
To find xy, we can divide both sides of the equation by 88:
xy=17600088=2000
Step 5: Calculate New Price and New Number of Visitors
After the increase, the new price of a ticket becomes 12x and the new number of visitors becomes 7y.
Step 6: Calculate New Revenue
Now, we can calculate the new revenue using the new price and new number of visitors:
New Revenue=(12x)×(7y)=84xy
Substituting the value of xy we found earlier:
New Revenue=84×2000=168000
Conclusion
The daily revenue after the increase in ticket prices is Rs. 1,68,000.
The ticket prices increase in the ratio of 11:12, and the number of visitors decreases in the ratio of 8:7.
Let the original price of a ticket be 11x and the original number of visitors be 8y.
The original revenue can be calculated using the formula:
Revenue=Price×Number of Visitors
So, the original revenue is:
Original Revenue=(11x)×(8y)=88xy
We know from the problem that this original revenue is Rs. 1,76,000:
88xy=176000
To find xy, we can divide both sides of the equation by 88:
xy=17600088=2000
After the increase, the new price of a ticket becomes 12x and the new number of visitors becomes 7y.
Now, we can calculate the new revenue using the new price and new number of visitors:
New Revenue=(12x)×(7y)=84xy
Substituting the value of xy we found earlier:
New Revenue=84×2000=168000
The daily revenue after the increase in ticket prices is Rs. 1,68,000.
Topper's Solved these Questions
Similar Questions
If by increasing the price of a ticket in the ratio 8:11 the number of tickets sold fall in the ratio 23:21 then what is the increase (in Rs) in revenue if revenue before increase in price of ticket was Rs. 36 ,800 ?
View SolutionThe entry ticket at a fun park was increased in the ratio 7:9 , due to which footfalls fell in the ratio 13:11 .What is the new daliy collection (in Rs) ,if the daily collection before the price hike was Rs. 2,27,500 ?
View Solution
Knowledge Check
- Question 1 - Select One
When ticket prices to a water park are in creased in the ratio 11:12 then the number of daily visitors to the park fall in the ratio 8:7 . If the daily revenues before the increase in ticket prices was Rs. 1,76,000 then find the daily revenues after the increase in ticket price .
ARs. 264000BRs. 112000CRs. 192000DRs. 168000 - Question 2 - Select One
If by increasing the price of a ticket in the ratio 8:11 the number of tickets sold fall in the ratio 23:21 then what is the increase (in Rs) in revenue if revenue before increase in price of ticket was Rs. 36 ,800 ?
A21250B9400C7850D12850 - Question 3 - Select One
The entry ticket at a fun park was increased in the ratio 7:9, due to which footfalls fell in the ratio 13:11. What is the new daily collection (in Rs.), if the daily collection before the price hike was Rs.2,27,500?
A237500B247500C232500D242500
The entrance ticket at the Akshara theatre in Mumbal is worth 250. When the price of the ticket was lowered, the sale of tickets Increased by 50% while the collections recorded a decrease of 17.5%. Find the deduction in the ticket price.
View SolutionThe ratio of the number of students of three class is 2:3:4 If 12 students are increased in each class then the ratio of becomes 8:11:14 .find the total students of all the three sections before adding 12 students in each class .
View SolutionPreviously, the manufacturing cost of a product was thrice the cost of raw material. Now the cost of raw material increases in the ratio 5:12 and manufacturing cost increases in the ratio of 3:5. The previous cost of the product was Rs. 8. What should be the present selling price so that 25% profit can be made? a. Rs.13.70 b. Rs.14.80 c. Rs.18.50 d. Rs.19.50
View SolutionStudy the following graph carefully to answer the question given below:
Given below is the demand and production of 6 brands (in units) of a product in the year 2016.
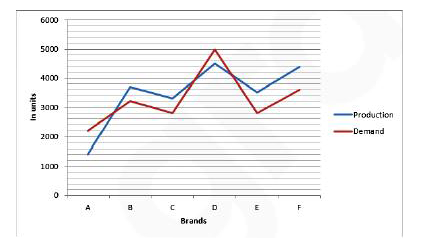
Brand B decreased its price of the product to meet its demand to its production. When the price decreased by 12% the demand increased by 25%. If the ratio between the new price and new demand is 11:20 then find the price of the product before the decrease.View SolutionIn a office the number of officer decrease in the ratio 11:9. There daily wages increase in the ratio 12 : 19. Then the total wages increase of one day is Rs. 19500. Then what was the starting wages.
View Solution

