A uniform ladder is placed against a wall, if the ground and wall are equally rough the coefficient of friction being tanθ limiting inclination of the ladder to the vertical is 2θ.
The correct Answer is:T
Similar Questions
A ladder 20 m long is placed against a wall so that the foot of the ladder is 10 m from the wall. The angle of inclination of the ladder to the horizontal will be :
View SolutionA ladder of length l and mass m is placed against a smooth vertical wall, but the ground is not smooth. Coefficient of friction between the ground and the ladder is μ. The angle θ at which the ladder will stay in equilibrium is
View Solution
Knowledge Check
A ladder of length l and mass m is placed against a smooth vertical wall, but the ground is not smooth. Coefficient of friction between the ground and the ladder is μ. The angle θ at which the ladder will stay in equilibrium is
Aθ=tan−1(μ)Bθ=tan−1(2μ)Cθ=tan−1(μ2)Dnone of theseA uniform ladder rests in limiting equilibrium with its lower end on a rough horizontal plane with coefficient of friction μ and its upper end against a smooth vertical wall. If θ is the inclination of the ladder with the wall, then θ is equal to
Atan−1μBcot−1μCcot−1(2μ)Dtan−1(2μ)A uniform ladder rests in limiting equilibrium with its lower end on a rough horizontal plane with coefficient of friction μ and its upper end against a smooth vertical wall. If θ is the inclination of the ladder with the wall, then θ is equal to
Atan−1μBcot−1μCcot−1(2μ)Dtan−1(2μ)
(i) A uniform ladder of length L and weight w rests against a vertical wall and makes an angle θ with the horizontal ground. If the coefficient of friction at the point of contact of the ladder with the wall and ground is μ, show that the greatest height x, measured along the ladder from the foot to which a man of weight w may climb without the ladder slipping is given by
xL=μ(W+w)W(1+μ2)(μ+tanθ)−w2W
(ii) If the wall be smooth and coefficient of friction between ladder and ground be 0.25, show that
x=L4(1−wW)tanθ−wL2W
View SolutionA ladder is placed with one edge on the wall 15 feet above the ground and the other end on the ground 8 feet away from the wall. Find the length of the ladder.
View SolutionA uniform ladder of length l rests against a smooth, vertical wall (figure). If the mass of the ladder is m and the the coefficient of static friction between the ladder and the ground is μs=0.375 and the minimum angle θmin at which the ladder does not slip.
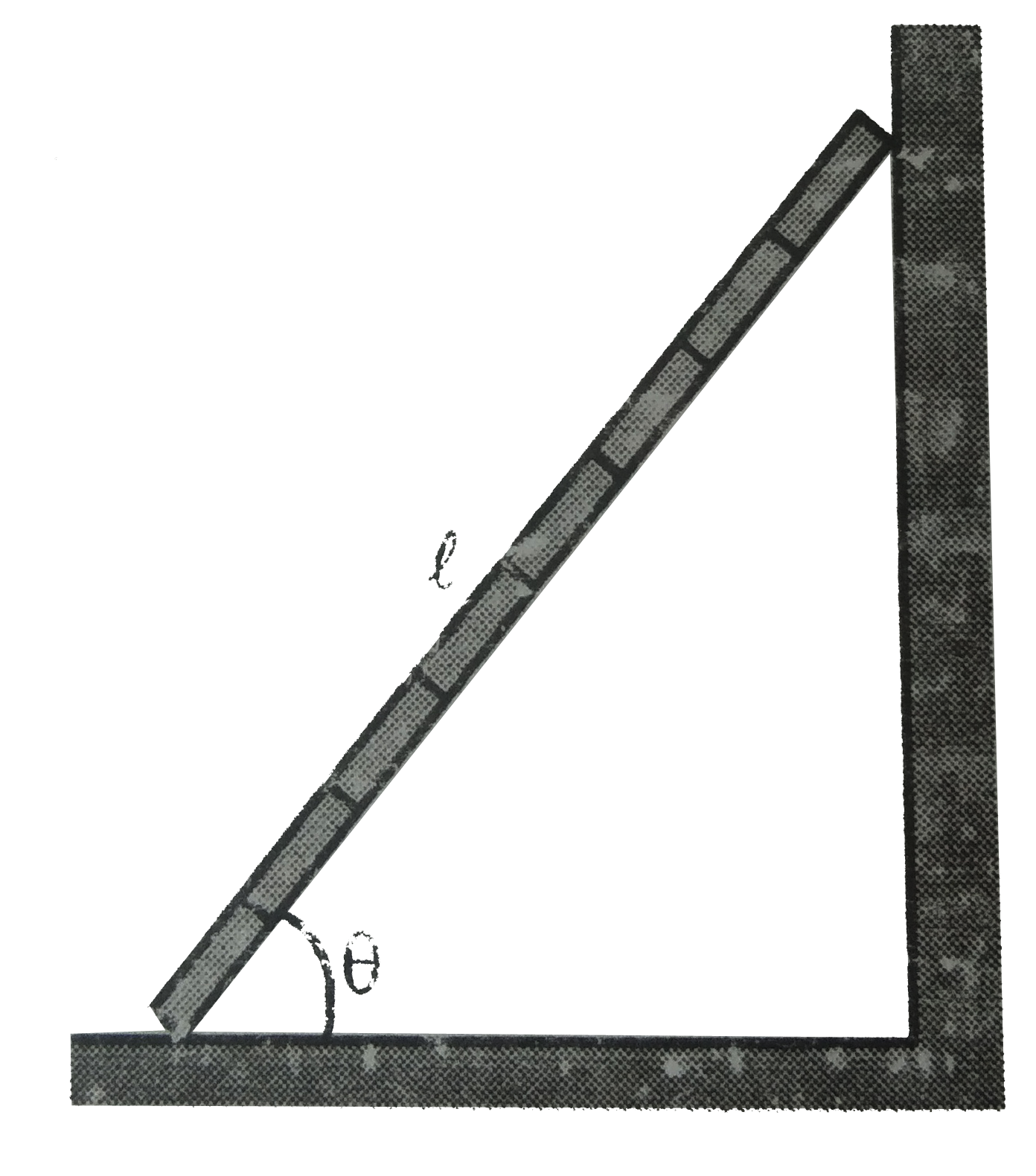
A uniform ladder at rest, leading against a smooth wall. Th ground is rough.
View SolutionA ladder, 10 metre long rests with one end against a smooth vertical wall and the other end on the ground which is rough, the coefficient of friction being 12. The foot of the ladder being 2 metre from the wall. A amn whose weight is 4 times that of the ladder can ascend before it be givens to slip is
View SolutionThe foot of a ladder leaning against a wall of length 5 metres rests on a level ground 5√3 metres from the base of the wall. The angle of inclination of the ladder with the ground is
View Solution
