Two fixed point charges 4Q and 2Q. are separated by a distance X. Where should the third point charge q be placed for it to be in equilibriam ? Solution in Punjabi
To solve the problem of finding the position where the third point charge q should be placed for it to be in equilibrium between two fixed point charges 4Q and 2Q separated by a distance x, follow these steps:
1. Identify the Forces:
- The third charge q will experience electrostatic forces due to both 4Q and 2Q.
- Let d be the distance from 4Q to q.
- Consequently, the distance from 2Q to q will be x−d.
2. Write the Force Expressions:
- The force F1 due to 4Q on q is given by Coulomb's law:
F1=k4Qqd2
- The force F2 due to 2Q on q is:
F2=k2Qq(x−d)2
3. Set Up the Equilibrium Condition:
- For q to be in equilibrium, the magnitudes of F1 and F2 must be equal:
k4Qqd2=k2Qq(x−d)2
- Simplify by canceling common factors k, Q, and q:
4d2=2(x−d)2
4. Solve for d:
- Cross-multiply to solve for d:
4(x−d)2=2d2
- Expand and simplify:
4(x2−2xd+d2)=2d2
4x2−8xd+4d2=2d2
4x2−8xd+2d2=0
- Divide the entire equation by 2:
2x2−4xd+d2=0
- Rearrange into a quadratic equation:
d2−4xd+2x2=0
5. Solve the Quadratic Equation:
- Use the quadratic formula d=−b±√b2−4ac2a, where a=1, b=−4x, and c=2x2:
d=4x±√(4x)2−4⋅1⋅2x22⋅1
d=4x±√16x2−8x22
d=4x±√8x22
d=4x±2√2x2
d=2x±√2x
6. Select the Appropriate Solution:
- Since d must be a positive distance less than x:
d=2x−√2x
- Simplify:
d=x(2−√2)
- The third charge q will experience electrostatic forces due to both 4Q and 2Q.
- Let d be the distance from 4Q to q.
- Consequently, the distance from 2Q to q will be x−d.
- The force F1 due to 4Q on q is given by Coulomb's law:
F1=k4Qqd2
- The force F2 due to 2Q on q is:
F2=k2Qq(x−d)2
- For q to be in equilibrium, the magnitudes of F1 and F2 must be equal:
k4Qqd2=k2Qq(x−d)2
- Simplify by canceling common factors k, Q, and q:
4d2=2(x−d)2
- Cross-multiply to solve for d:
4(x−d)2=2d2
- Expand and simplify:
4(x2−2xd+d2)=2d2
4x2−8xd+4d2=2d2
4x2−8xd+2d2=0
- Divide the entire equation by 2:
2x2−4xd+d2=0
- Rearrange into a quadratic equation:
d2−4xd+2x2=0
- Use the quadratic formula d=−b±√b2−4ac2a, where a=1, b=−4x, and c=2x2:
d=4x±√(4x)2−4⋅1⋅2x22⋅1
d=4x±√16x2−8x22
d=4x±√8x22
d=4x±2√2x2
d=2x±√2x
- Since d must be a positive distance less than x:
d=2x−√2x
- Simplify:
d=x(2−√2)
Topper's Solved these Questions
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - ELECTRIC CHARGES AND ELECTROSTATIC FORCE EXERCISE - NUMERICAL PROBLEMS13videos
ELECTRIC CHARGES AND ELECTROSTATIC FORCE
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - DIPERSION OF LIGHTEXERCISE - MOST EXPECTED QUESTIONS5videos
DIPERSION OF LIGHT
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - ELECTRIC CURRENT, RESISTANCE AND E.M.F.EXERCISE - MOST EXPECTED QUESTIONS14videos
ELECTRIC CURRENT, RESISTANCE AND E.M.F.
Similar Questions
How many electrons make up one Coulomb of charge ?
06:23View SolutionWhat do you mean by dispersion of light?
07:08View SolutionDefine SI unit of resistance.
04:25View SolutionTwo fixed point charges +4e and +e units are separated by a distance a. Where should a third point charge be placed for it to be in equilibrium?
08:47View SolutionTwo charges q and 3q are placed fixed on x-axis separated by distance 'd'. Where should a third charge 2q be placed such that it will not experience any force ?
08:47View SolutionTwo point metal charges−q and +2q are placed at a certain distance apart.Where should a third point charge be placed so that it is in equilibrium?
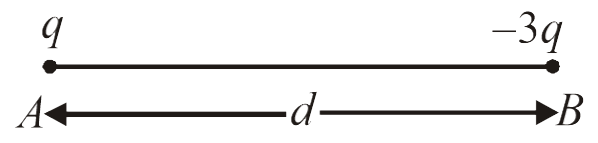
05:57View SolutionTwo charge q and –3q are placed fixed on x–axis separated by distance d. Where should a third charge2q be placed such that it will not experience any force ?
 05:39View Solution
05:39View SolutionTwo charge q and –3q are placed fixed on x–axis separated by distance d. Where should a third charge2q be placed such that it will not experience any force ?
 View Solution
View SolutionTwo points charges +4 and +q are separated by distance r, where should a third point charge Q be placed that the whole system remains in equilibrium
07:15View SolutionThe two stationary point charges 4Q and 2Q lie x opposite to each other. Where should the third point charge q be placed in equilibrium?
03:25View SolutionTwo charges Q and -3Q are placed fixed on x-axis separated by a distance 'd' Where should a third charge 2Q be placed such that it will not experience any force?
04:38View SolutionTwo charges q and - 3q are placed fixed on x-axis separated by distance'd'. Where should a third charge 2q be placed such that it will not experience any force ?
04:57View SolutionTwo charges q and -3q are placed fixed on x-axis separated by distance d. where should a third charge 2q be placed such that it will not experience any force?
03:16View SolutionTwo charges q and −3q are placed fixed on x-axis separated by distance d. Where should a third charge 2q be placed such that it will not experience any force ?
07:03View SolutionTwo fixed point charges +4q and +q units are separeted by a distance 'x'. Where should a third point charge- q_0 be placed for it to be in equilibrium?
06:12View SolutionTwo fixed charges +4q and +q are at a distance 3 m apart. At what point between the charge, a third charge +q must be placed to keep it in equilibrium ?
05:03View Solution

