State Gauss' law and using this law , derive an expression for the electric field intensity due to a uniformly charged thin spherical shell at a point outside the shell.Solution in Punjabi
Gauss.s Theorem : It states that total electric flux through a closed surface is equal to 1ε0 times the magnitude of charge enclosed by it.
ϕ=∮→E.−→dS=qε0
Electric field due to uniformly charged spherical shell : Consider a spherical shell of radius R. .O. is the centre of the spherical shell ad + Q charge be uniformly distributed over the surface of the shell.
Let σ = Surface charge density
∴ Total charge on the shell = Q=σ(4πR2)
(i) →E at a point outside the shell :
Let P be a point outside the shell at a distance r (OP = r), where electric field intensity is to be determined. Draw a spherical Gaussian surface of radius r with centre at O. Consider a small area elements dS on this Gaussian surface
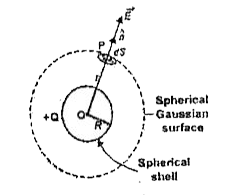
By symmetry consideration, the magnitude of →E is same at all points on this surface and direction is radially outward.
Now flux through area dS is given by
dϕE=→E.−→dS=→E.ˆndS
where ˆn is a unit vector normal to area dS
or dϕE=E(1)=dscos0∘
= E dS [∴ˆE∣∣ˆn,∴θ=θ]
Total flux through whole surface
ϕE=∮EdS
Using Gauss.s theorem,
ϕE=Qε0
From eqns, (2) and (3)
E∮dS=Qε0
[∴Area of Gaussian spherical surface=4πr2]
E(4πr2)=Qε0
⇒E=Q4πr2ε0=14πε0Qr2
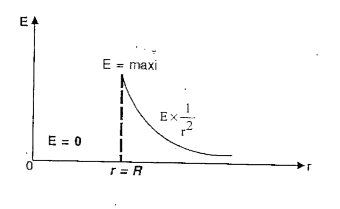
which is also the expression for electric field due to a point charge It means that for a point lying outside the uniformaly charged spherical shell, the whole charged spherical shell behaves like a point charge.
Using eqn. (1) in eqn. (4), we have
E=14πε0σ(4πR2)r2
E=σR2ε0r2
(ii) →E at a point on the surface of shell (r = R) :
From eqn. (4), we get
E=Q4πε0R2
But Q=(4πR2)σ
∴E=(4πR2)4πε0R2=σε0orE=σε0
→E at a point inside the shell :
It the point P lies inside the shell at a distance r<R, then radius of Gaussian surface will be less than the radus of shell. Therefore, in thi case, Gaussian surface encloses no charge, i.e., q= 0 Using Gauss.s theorem,
∮→E.−→dS=qεon0=0
∴E=0
i.e., electric field intensity at all points inside a uniformly charged shell is zero.
The variation of →E with distance from the centre of uniformly charged spherical shell is shown in fig. given below.
Topper's Solved these Questions
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - GAUSS'S THEOREMEXERCISE - MOST EXPECTED NUMERICALS4videos
GAUSS'S THEOREM
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - GAUSS'S THEOREMEXERCISE - SHORT ANSWER TYPE QUESTIONS8videos
GAUSS'S THEOREM
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - FORCE ON A CURRENTEXERCISE - NUMERICAL PROBLEMS10videos
FORCE ON A CURRENT
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - HUYGENS' PRINCIPLEEXERCISE - LONG ANSWER TYPE QUESTIONS11videos
HUYGENS' PRINCIPLE
Similar Questions
A box encloses an electric dipole of 3μCand−3C, if the dipole length is 6 cm, then find total electric flux associated with box.
06:11View SolutionWhat is the use of Gaussian surface in electrostatics ?
04:55View SolutionA currentof 5.0 A is flowing in eah of the two parallel conductors of infinite length separated by certain distance apart in vacuum in the same directions. Each conductor expereinces a force of 2×10−4Nm−1 due to the other. Find the separation between the two conductors.
04:20View SolutionState Huygen's principle of propagation of light and prove the laws (Snell's law) of refraction on its basis.
05:18View Solutionगॉस के नियम का उपयोग करके सिद्ध कीजिए कि एकसमान आवेशित गोलीय कोश के अन्दर विद्युत् क्षेत्र की तीव्रता शून्य होती है।
04:34View SolutionUsing Gauss' law deduce the expression for the electric field due to a uniformly charge spherical conducting shell of radius R at a point (i) outside the shell.
04:13View SolutionUsing Gauss' law deduce the expression for the electric field due to a uniformly charge spherical conducting shell of radius R at a point (ii) inside the shell.
05:18View SolutionState Gauss's law. Derive an expression for electric intensity at a point outside the uniformly charged shell.
05:18View SolutionDerive an expression for electric intensity E due to a uniformly charged thin spherical shell at a point outside the shell.
05:28View SolutionFind expression for electric field intensity due to a uniformly charged thin spherical shell at a point outside the shell.
10:17View SolutionState Gauss's theorem. Obtain an expression for elactric field at any point outside a charged spherical hollow conductor (shell).
06:26View SolutionState Gauss's theorem. Obtain an expression for elactric field at any point outside a charged spherical hollow conductor (shell).
08:05View SolutionState Gauss's theorem with the help of diagram, derive an expression for the electric field intensity due to uniformly charged thin spherical shell at a point outside
06:13View SolutionState Gauss's theorem with the help of diagram, derive an expression for the electric field intensity due to uniformly charged thin spherical shell at a point on the surface of the spherical shell.
07:26View SolutionDerive an expression for electric field due to a uniformly charged spherical shell. i) at a point outside the shell
01:49View SolutionDerive the expression for electric field intensity due to a uniformly charged thin spherical shell at a point outside the shell
01:49View Solution

