State Gauss' theorem in electrostatics. Derive an expression for the electric field intensity at any point of to an infinite plane sheet of charge.Solution in Punjabi
Gauss.s Theorem : It states that total electric flux through a closed surface enclosing a charge is equal to 1ε0 times the magnitude of charge enclosed. Mathematical form of Gauss.s Las :
Electric flux =ϕ=∮→E.−→dSqε0
Electric field intensity due to an infinite plane thin sheet of charge :
Consider a thin, infinite plane sheet of charge.
Let σ = Surface charge density on both sides
Consider a point P at a distance r from the sheet.
Draw a cylindrical Gaussian surface of cross - section S through the point P with lenght r on each side of the cap.
Electric field E is perpendicular to its end circular Cpas I and II.
Charge enclosed by the Gaussian surface = q=σS
Using Gauss.s theorem
∮→E.−→dS=qε0=σSε0
Gaussian surface is divided into three parts, I II and III
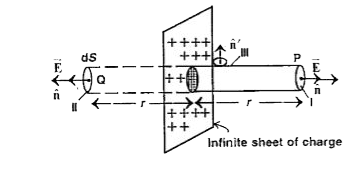
∴ Eqn. (i) becomes ∮I→E.−→dS+II–––∮→E.−→dS+∮III→E.−→dS=σSε0
Angle between →Eand−→dS is 90∘ for the curved III. In this case ∮III→E.−→dS=E.dScos90∘=0
Hence eqn. (ii) becomes ∮I→E.−→dS=undereset(II)∮→E.−→dS=σSε0
Since angle between →Eand−→dS for surface I and II is zero, so
∮I→E.dScos0∘+∮IIE.dScos0∘=σSε0or∮IE.dS+∮IIEdS=σSε0
Since electric field intensity E is Constant at very point of the Gaussian surface so
∴E∮IdS+E∮IIdS=σAε0orES+ES=σSε0[∴∮dS=S=Area of end cap]
or 2ES=σε0
or E=σ2ε0
If the plane sheet has a finite thickness : then charges on both sides of the sheet are to be considered. Thus equation (i) is written as ∫→E.−→dS=2σSε0
Then above eqn. becomes 2ES = 2simgmaε0 i.e., E=σε0 [theregore∫→E.−→dS=2ES]
Topper's Solved these Questions
- GAUSS'S THEOREMBOOK - BETTER CHOICE PUBLICATIONCHAPTER - GAUSS'S THEOREMEXERCISE - MOST EXPECTED NUMERICALS
4 Videos
- GAUSS'S THEOREMBOOK - BETTER CHOICE PUBLICATIONCHAPTER - GAUSS'S THEOREMEXERCISE - SHORT ANSWER TYPE QUESTIONS
8 Videos
- FORCE ON A CURRENTBOOK - BETTER CHOICE PUBLICATIONCHAPTER - FORCE ON A CURRENTEXERCISE - NUMERICAL PROBLEMS
10 Videos
- HUYGENS' PRINCIPLEBOOK - BETTER CHOICE PUBLICATIONCHAPTER - HUYGENS' PRINCIPLEEXERCISE - LONG ANSWER TYPE QUESTIONS
11 Videos
Similar Questions
A box encloses an electric dipole of 3μCand−3C, if the dipole length is 6 cm, then find total electric flux associated with box.
View SolutionWhat is the use of Gaussian surface in electrostatics ?
View SolutionA currentof 5.0 A is flowing in eah of the two parallel conductors of infinite length separated by certain distance apart in vacuum in the same directions. Each conductor expereinces a force of 2×10−4Nm−1 due to the other. Find the separation between the two conductors.
View SolutionState Huygen's principle of propagation of light and prove the laws (Snell's law) of refraction on its basis.
View SolutionState Gauss's law in electrostatics. Using this law derive an expression for the electric field due to a uniformly charged infinite plane sheet.
View Solutionस्थिर विद्युत् के अन्तर्गत गॉस का प्रमेय लिखिए एवं सिद्ध कीजिए | आवेश के अनन्त समतल चादर के समीप विद्युत् क्षेत्र की तीव्रता के लिए व्यंजक ज्ञात कीजिए |
View SolutionState Gauss's law in electrostatics. Applying Gauss's law derive the expression for electric intensity due to an infinite plane sheet of charge.
View SolutionState Gauss's theorem in electrostatics. Apply this theorem to derive an expression for electric field intensity at a point outside a uniformly charged thin spherical shell.
View SolutionState Gauss's law in electrostatics. Using this law derive an expression for the electric field due to a uniformly charged infinite plane sheet.
View SolutionState Gauss.,s theorem. Derive an expression for eletric field intensity at a point to an infinite plane sheet of charges.
View SolutionState Gauss.s theorem in electrostatics. Using it, derive an experssion for electric field intensity at a point due to infinite sheet of charge. How does the electric field change for a thick sheet of charge?
View SolutionState Gauss theorem in electrostatics. Derive the expression for electric field at a point due to an infinitely long straight charged conductor.
View SolutionDerive an expression for an electric field due to a charged infinite plane sheet.
View Solutionस्थिरवैद्युतिकी में गाउस की प्रमेय बताइये । एक अनन्त लम्बे सीधे आवेशित तार के निकट के एक बिन्दु पर विद्युत क्षेत्र तीव्रता के लिये व्यंजक व्युत्पन्न करने के लिये इस प्रमेय का प्रयोग कीजिये।
View SolutionState and prove Gauss. theorem in electrostatics. Apply it to find the electric field intensity due to a charged plane lamina in its neighbourhood.
View SolutionState Gauss' theorem in electrostatics. Apply it to find the electric field intensity due to a charged plane lamina in its neighbourhood.
View Solution
