Question

What is electric potential enerrgy due to a system of charges? Derive an expression for it.

Explanation

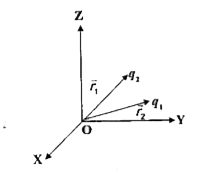
Electric potential energy due to two point charge system : Let us consider two point charges `q_(1)` and `q_(2)` lying at points A and B respectively in space. Let `vecr_(1)` and `vecr_(2)` be their position vectors W.r.t. origin O as shown in fig. below.
Move both the charges to such positions so that they are at infinite distance apart from each other.
First of all move one of the charges say `q_(1)`, from infinity and bring it to its location, i.e., at A. For this no work has to be done, because there is supposed to be no electric field at A,

i.e., work done = 0 in moving charge q, from infinity to point A.
Now move other charge `q_(2)` from infinity to its location at B. Since, `q_(2)`, is moved in the electrostatic field of charge `q_(1)` lying at A, hence, work has to be done.
The amount of work done in moving the charge `q_(2)` from infinity to point B in the electric field of charge `q_(1)` is given by
W = Work done in moving charge q, from infinity to point `B(vecr_(2))`.
or W= (Work done in moving unit positive charge from infinity to point B) `xxq_(2)` = (Electric potential due to charge `q_(1)` at point B) `xxq_(2)`
( `:.` Potential is the amount of work in bringing a unit positive charge from infinity to that point)
`W=((1)/(4piin_(0))(q_(1))/(r_(12)))q_(2)`
where `r_(12)` = distance between charges `q_(1)` and `q_(2)`.
Since electrostatic force is conservative, this work gets stored in the system of two point charges in the form of electric potential energy of the system. Therefore,
`U=(q_(1)q_(2))/(4piin_(0)r_(12))`
Relation between potential of a charge and its potential energy :
Potential energy of the system of two point charges is given as
`U=(1)/(4piin)(q_(1)q_(2))/(r_(12))`
`U=((1)/(4piin_(0))(q_(1))/(r_(12)))q_(2)`
(Potential due to charge `q_(1)` ) `xxq_(2)`
`U=Vq_(2)`
In general `U=qV`