Statement-1: The stream of water emerging from a wate tap "necks down" as it falls.
Statement-2: The volume flow rate at different levels is same.
Statement-1, is true, Statement-2 is true, Statement-2 is correct explanation for statement-1
Statement-1 is true, statement-2 is true, statement-2 is NOT a correct explantion for statement-1
statement-1 is true, statement-2 is false
statement-1 is false statement-2 is true
The correct Answer is:A
To solve the problem, we need to analyze the two statements given:
Statement-1: The stream of water emerging from a water tap "necks down" as it falls.
Statement-2: The volume flow rate at different levels is the same.
1. Understanding Volume Flow Rate:
The volume flow rate (Q) is defined as the volume of fluid that passes through a given surface per unit time. It can be expressed as:
Q=A⋅v
where A is the cross-sectional area and v is the velocity of the fluid.
2. Applying the Continuity Equation:
For an incompressible fluid (like water), the volume flow rate must remain constant along the stream. Therefore, at two points (1 and 2) in the stream:
Q1=Q2⟹A1⋅v1=A2⋅v2
where A1 and v1 are the area and velocity at point 1, and A2 and v2 are the area and velocity at point 2.
3. Considering the Effect of Gravity:
As the water falls, it accelerates due to gravity. This means that the velocity of the water increases as it falls:
v2>v1
Since the volume flow rate must remain constant, if v2 increases, then A2 must decrease to keep the product A⋅v constant.
4. Conclusion on Areas:
From the equation A1⋅v1=A2⋅v2, if v2>v1, it follows that:
A2<A1
This indicates that the cross-sectional area of the stream of water decreases (or "necks down") as it falls.
5. Final Evaluation of Statements:
- Statement-1 is true: The stream of water does neck down as it falls.
- Statement-2 is also true: The volume flow rate at different levels is indeed the same, as derived from the continuity equation.
Thus, we conclude that both statements are true, and Statement-2 correctly explains Statement-1.
Final Answer:
Both statements are true, and Statement-2 is the correct explanation of Statement-1.
---
Statement-2: The volume flow rate at different levels is the same.
The volume flow rate (Q) is defined as the volume of fluid that passes through a given surface per unit time. It can be expressed as:
Q=A⋅v
where A is the cross-sectional area and v is the velocity of the fluid.
For an incompressible fluid (like water), the volume flow rate must remain constant along the stream. Therefore, at two points (1 and 2) in the stream:
Q1=Q2⟹A1⋅v1=A2⋅v2
where A1 and v1 are the area and velocity at point 1, and A2 and v2 are the area and velocity at point 2.
As the water falls, it accelerates due to gravity. This means that the velocity of the water increases as it falls:
v2>v1
Since the volume flow rate must remain constant, if v2 increases, then A2 must decrease to keep the product A⋅v constant.
From the equation A1⋅v1=A2⋅v2, if v2>v1, it follows that:
A2<A1
This indicates that the cross-sectional area of the stream of water decreases (or "necks down") as it falls.
- Statement-1 is true: The stream of water does neck down as it falls.
- Statement-2 is also true: The volume flow rate at different levels is indeed the same, as derived from the continuity equation.
Both statements are true, and Statement-2 is the correct explanation of Statement-1.
Topper's Solved these Questions
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 3 (Comprehension based questions)
24 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 4 A (Elasticity)
7 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 2 (One or more than one correct answer)
53 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B
19 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
Similar Questions
Water falls from a tap with A0=4m2, A=1m2 and h=2 m, then velocity v is
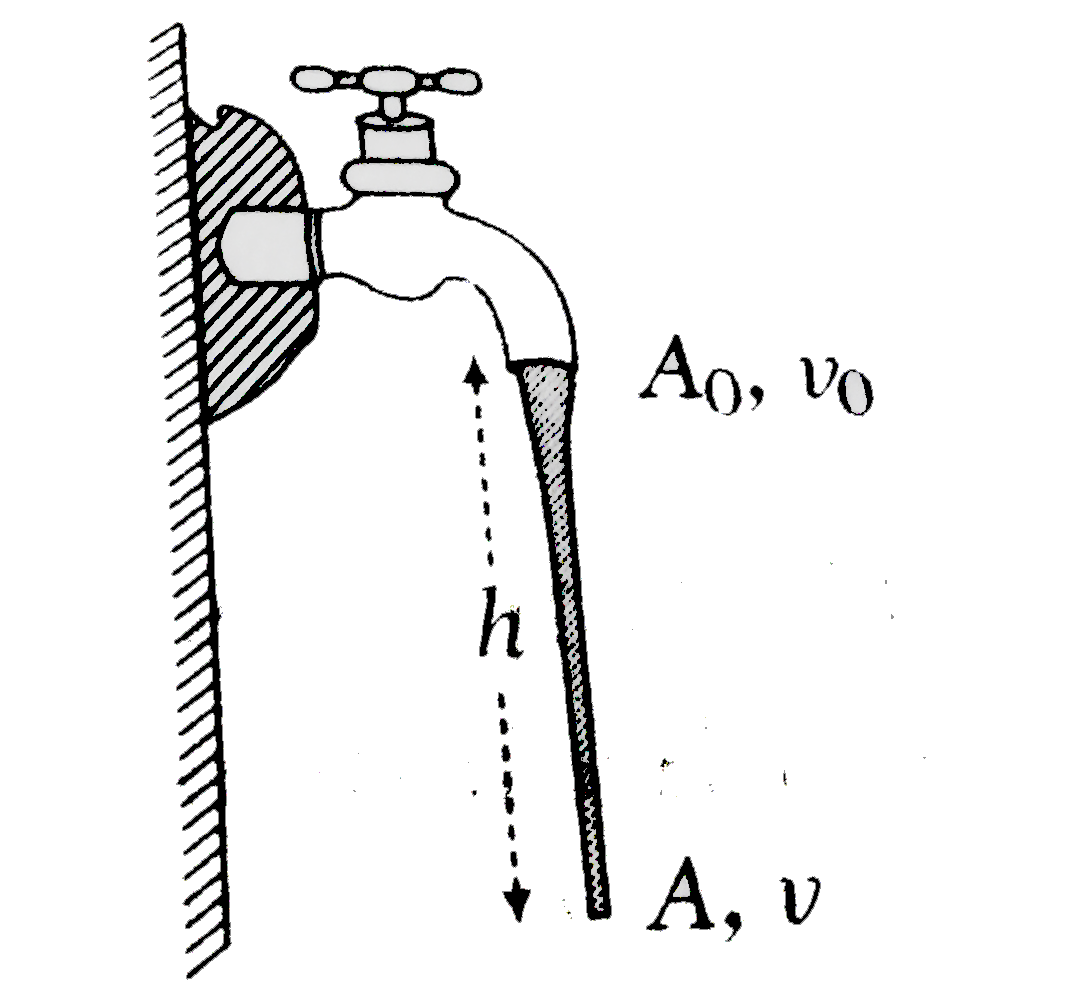
View SolutionStatement-1: Elasticity restoring forces may be conservative.
Statement-2: The value of strain for same stress are different while increasing the load and while decreasing the load.
View SolutionStatement-1 : The freezing of water is an endothermic process.
Statement-2 : Heat must be removed from the water to make it freeze.
View SolutionStatement-1: A parachute descends slowly whereas a stone dropped from same height falls rapidly.
Statement-2: The viscous force of air on parachute is larger than that of o a falling stone.
View SolutionStatement 1: Primary, secondary and tertiary amines can be separated by Hinsburg reagent and
Statement 2: These amines have different rate of reaction with benzens sulfonyl chloride.
View SolutionStatement -1: Entropy change in reversible adiabatic expansion of an ideal gas is zero.
Statement-2: The increase in entropy due to volume increase just compensates the decrease in entropy due to fall in temperature.
View SolutionStatement-1: Standing waves can not be obtained in air if the interfering waves are of diffeerent amplitudes.
Statement-2: In a stationary wave, different particles in a loop have different amplitudes but same phase.
View SolutionStatement-1: The shape of an automobile is so designed that its front resembles the stream line pattern of the fluid through which is moves.
Statement-2: The shape of the automobile is made stream lines in order to reduced resistance offered by the fluid.
View SolutionFour numbers are chosen at random (without replacement) from the set {1, 2, 3, ....., 20}. Statement-1: The probability that the chosen numbers when arranged in some order will form an AP Is 185 . Statement-2: If the four chosen numbers form an AP, then the set of all possible values of common difference is {1, 2, 3, 4, 5}. (1) Statement-1 is true, Statement-2 is true; Statement-2 is not the correct explanation for Statement-1 (2) Statement-1 is true, Statement-2 is false (3) Statement-1 is false, Statement-2 is true (4) Statement-1 is true, Statement-2 is true; Statement-2 is the correct explanation for Statement-1
View SolutionStatement-1: Water is considered unsuitable for use in thermometers.
Statement-2: Thermal Expansion of water is non uniform.
View Solution
