When an object moves through a fluid, as when a ball falls through air or a glass sphere falls through water te fluid exerts a viscous foce F on the object this force tends to slow the object for a small sphere of radius r moving is given by stoke's law, Fw=6πηrv. in this formula η in the coefficient of viscosity of the fluid which is the proportionality constant that determines how much tangential force is required to move a fluid layer at a constant speed v, when the layer has an area A and is located a perpendicular distance z from and immobile surface. the magnitude of the force is given by F=ηAv/z. For a viscous fluid to move from location 2 to location 1 along 2 must exceed that at location 1, poiseuilles's law given the volumes flow rate Q that results from such a pressure difference P2−P1. The flow rate of expressed by the formula Q=πR4(P2−P1)8ηL poiseuille's law remains valid as long as the fluid flow is laminar. For a sfficiently high speed however the flow becomes turbulent flow is laminar as long as the reynolds number is less than approximately 2000. This number is given by the formula Re=2¯vρRη In which ¯v is the average speed ρ is the density η is the coefficient of viscosity of the fluid and R is the radius of the pipe. Take the density of water to be ρ=1000kg/m3
Q. If the sphere in previous question has mass of 1×10−5kg what is its terminal velocity when falling through water? (η=1×10−3Pa−s)
A. 1.3m/s
B. 3.4m/s
C. 5.2m/s
D. 6.5m/s
1.3m/s
3.4m/s
5.2m/s
6.5m/s
The correct Answer is:C
To find the terminal velocity of a sphere falling through water, we can use Stokes' law, which relates the viscous force acting on the sphere to its velocity. The formula given by Stokes' law is:
Fw=6πηrv
Where:
- Fw is the viscous force,
- η is the coefficient of viscosity of the fluid,
- r is the radius of the sphere,
- v is the velocity of the sphere.
At terminal velocity, the viscous force equals the weight of the sphere. Therefore, we can set the two forces equal:
Fw=mg
Where:
- m is the mass of the sphere,
- g is the acceleration due to gravity (approximately 10m/s2).
Setting these equal gives us:
6πηrvt=mg
Now, we can solve for the terminal velocity vt:
vt=mg6πηr
Step 1: Identify the given values
- Mass of the sphere, m=1×10−5kg
- Coefficient of viscosity, η=1×10−3Pa.s
- Radius of the sphere, r=1mm=1×10−3m
- Acceleration due to gravity, g=10m/s2
Step 2: Substitute the values into the terminal velocity formula
Now we substitute the known values into the formula:
vt=(1×10−5kg)(10m/s2)6π(1×10−3Pa.s)(1×10−3m)
Step 3: Calculate the numerator
Calculating the numerator:
mg=(1×10−5)(10)=1×10−4N
Step 4: Calculate the denominator
Calculating the denominator:
6πηr=6π(1×10−3)(1×10−3)=6π×10−6≈1.884×10−5N.s/m
Step 5: Calculate terminal velocity
Now substituting the values back into the equation for vt:
vt=1×10−41.884×10−5≈5.3m/s
Conclusion
The terminal velocity of the sphere falling through water is approximately 5.2m/s.
Final Answer
Thus, the correct answer is C. 5.2 m/s.
- Fw is the viscous force,
- η is the coefficient of viscosity of the fluid,
- r is the radius of the sphere,
- v is the velocity of the sphere.
- m is the mass of the sphere,
- g is the acceleration due to gravity (approximately 10m/s2).
- Mass of the sphere, m=1×10−5kg
- Coefficient of viscosity, η=1×10−3Pa.s
- Radius of the sphere, r=1mm=1×10−3m
- Acceleration due to gravity, g=10m/s2
Now we substitute the known values into the formula:
Calculating the numerator:
Calculating the denominator:
Now substituting the values back into the equation for vt:
The terminal velocity of the sphere falling through water is approximately 5.2m/s.
Thus, the correct answer is C. 5.2 m/s.
Topper's Solved these Questions
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 4 A (Elasticity)
7 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 4 A (Surface Tension)
5 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 3 (Assertion & Reason)
32 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B
19 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
Similar Questions
In the figure shown the straing versus stress graph for two values of young's modulus ?
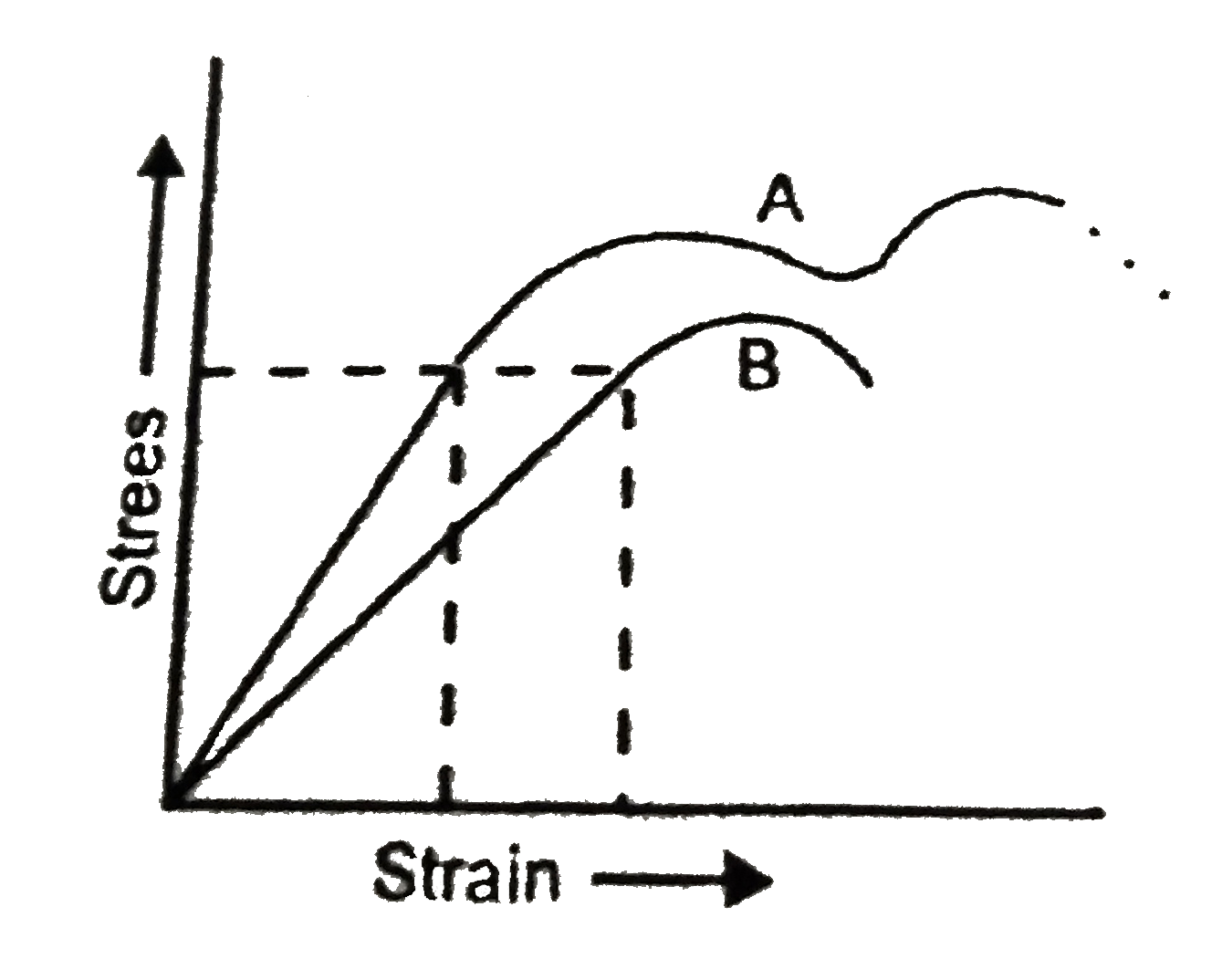
(i) which material is more ductile ? Explain.
(ii) Which material is more brittle ? Explain.
(iii) Which material is stronger ? Explain.
View Solution_E01_194_Q01.png)
PQRS is a rectangular frame of copper wire shown in figure the side RS of the frame is movable. If a soap film is formed on it then what is the diameter of the wire to maintain equilibirum (given surface tension of soap solution =0.045N/m and density of copper =8.96×103kg/m3)
View SolutionStatement-1: A solid sphere and a hollow sphere of same material and same radius are floating in a liquid. Same percentage of volume of both the spheres is immersed in the liquid.
Statement-2: Up-thrust force acts on volume of liquid displaced. It has nothing to do whether the body is solid or hollow.
View SolutionTwo particles of mases m1 and m2 in projectile motion have velocitie →v1 and →v2, respectively at time t=0. they collide at time t0. Their velocities become →v′1 and →v′2 at time 2t0 while still moving in air. The value of
∣∣(m1→v′1+m2→v′2)∣∣−|(m1→v1+m2→v2)| is
View SolutionA cube has a side of length 1.2×10−2m. Its volume upto correct significant figures is
View SolutionA sphere of mass M and radius R is falling in a viscous fluid. The terminal velocity attained by the falling object will be alphaortional to :
View SolutionWhen an object moves through a fluid, as when a ball falls through air or a glass sphere falls through water te fluid exerts a viscous foce F on the object this force tends to slow the object for a small sphere of radius r moving is given by stoke's law, Fw=6πηrv. in this formula η in the coefficient of viscosity of the fluid which is the proportionality constant that determines how much tangential force is required to move a fluid layer at a constant speed v, when the layer has an area A and is located a perpendicular distance z from and immobile surface. the magnitude of the force is given by F=ηAv/z. For a viscous fluid to move from location 2 to location 1 along 2 must exceed that at location 1, poiseuilles's law given the volumes flow rate Q that results from such a pressure difference P2−P1. The flow rate of expressed by the formula Q=πR4(P2−P1)8ηL poiseuille's law remains valid as long as the fluid flow is laminar. For a sfficiently high speed however the flow becomes turbulent flow is laminar as long as the reynolds number is less than approximately 2000. This number is given by the formula Re=2¯vρRη In which ¯v is the average speed ρ is the density η is the coefficient of viscosity of the fluid and R is the radius of the pipe. Take the density of water to be ρ=1000kg/m3
Q. Blood vessel is 0.10 m in length and has a radius of 1.5×10−2m blood flows at rate of 10−7m3/s through this vessel. The pressure difference that must be maintained in this flow between the two ends of the vessel is 20 Pa what is the viscosity sufficient of blood?
View SolutionA small metal sphere of radius a is falling with a velocity υ through a vertical column of a viscous liquid. If the coefficient of viscosity of the liquid is η, then the sphere encounters an opposing force of
View Solutionजब एक गोलाकार वस्तु किसी द्रव में चलती है तो द्रव उसपर गति की विपरीत दिशा में एक बल लगता है। इस बल का परिमाण F, द्रव का श्यानता गुणांक (coefficient of viscosity) η, गोले की त्रिज्या r तथा गोले के वेग v पर निर्भर करता है। विमाओं की सहायता से F के लिए η, v तथा r में सूत्र प्राप्त करे।
View SolutionViscous force on a small sphere of radius r moving in a fluid varies directly with
View SolutionA small metal sphere of radius a is falling with a velocity through a verticle column of a viscous liquid. If the coefficient of viscosity of the liquid is η, then the sphere encounters an opposing force of
View SolutionA small metal sphere of radius is rapidly falling down through a vertical viscous fluid column. The viscosity of this fluid eta This is the force that the metal sphere will feel against the motion
View SolutionA sphere of mass M and radius R is falling in a viscous fluid .The terminal velocity attained by the falling object will be proportional to ……
View SolutionA sphere of mass M and radius R is faling in a viscous fluid. The terminal velocity attained by the falling object will be proportional to
View SolutionA small metal sphere of radius a is falling with a velocity v through a vertical column of a viscous liquid. If the coefficient of viscosity of the liquid is eta , then the sphere encounters an opposing force of
View SolutionViscous force on a small sphere of radius r moving in a fluid varies directly with
View Solution
