Light wavelength 6000 Å is incident normally on a slit of width 24×10−5 cm. find out the angular position of seconds minimum from central maximum?
To solve the problem of finding the angular position of the second minimum from the central maximum in a single-slit diffraction pattern, we can follow these steps:
Step 1: Understand the given data
- Wavelength of light, λ=6000Å=6000×10−10m
- Width of the slit, a=24×10−5cm=24×10−7m
Step 2: Use the formula for minima in single-slit diffraction
The condition for minima in single-slit diffraction is given by:
asinθ=nλ
where n is the order of the minimum. For the second minimum, n=2.
Step 3: Substitute the values into the formula
Substituting n=2 into the formula gives:
asinθ=2λ
Now substituting the values of a and λ:
24×10−7sinθ=2×(6000×10−10)
Step 4: Simplify the equation
Calculating 2×(6000×10−10):
2×6000=12000and12000×10−10=1.2×10−6
Now the equation becomes:
24×10−7sinθ=1.2×10−6
Step 5: Solve for sinθ
Dividing both sides by 24×10−7:
sinθ=1.2×10−624×10−7=1.224=0.05
Step 6: Calculate θ
Now, we find θ by taking the inverse sine:
θ=sin−1(0.05)
Using a calculator or trigonometric tables, we find:
θ≈2.89∘
Step 7: Conclusion
The angular position of the second minimum from the central maximum is approximately 2.89∘.
- Wavelength of light, λ=6000Å=6000×10−10m
- Width of the slit, a=24×10−5cm=24×10−7m
The condition for minima in single-slit diffraction is given by:
asinθ=nλ
where n is the order of the minimum. For the second minimum, n=2.
Substituting n=2 into the formula gives:
asinθ=2λ
Now substituting the values of a and λ:
24×10−7sinθ=2×(6000×10−10)
Calculating 2×(6000×10−10):
2×6000=12000and12000×10−10=1.2×10−6
Now the equation becomes:
24×10−7sinθ=1.2×10−6
Dividing both sides by 24×10−7:
sinθ=1.2×10−624×10−7=1.224=0.05
Now, we find θ by taking the inverse sine:
θ=sin−1(0.05)
Using a calculator or trigonometric tables, we find:
θ≈2.89∘
The angular position of the second minimum from the central maximum is approximately 2.89∘.
Topper's Solved these Questions
Similar Questions
A light wave is incident normally over a slit of width 24×10−5cm. The angular position of second dark fringe from the central maxima is 30∘. What is the wavelength of light?
View SolutionLights of wavelength 550 nm falls normally on a slit of width k22.0×10−5cm. The angular position of the central maximum will be (in radian) :
View SolutionLight of wavelength 600 nm is incident normally on a slit of width 0.2 mm. The angular width of central maxima in the diffraction pattern is
View SolutionLight of wavelength 6328Å is incident normally on a slit of width 0.2 mm. Angular width of the central maximum on the screen will be :
View SolutionLight of wavelength 5000Å is incident over a slit of width 1μm. The angular width of central maxima will be
View SolutionA plane wave of wavelength 6250Å is incident normally on a slit of width 2×10−2 cm The width of the principal maximum of diffraction pattern on a screen at a distance of 50 cm will be
View SolutionLight of wavelength 589.3nm is incident normally on the slit of width 0.1mm. What will be the angular width of the central diffraction maximum at a distance of 1m from the slit?
View SolutionLight of wavelength 5000 Å is incident on a slit of width 0.1 mm. Find out the width of the central bright line on a screen distance 2m from the slit?
View SolutionA monochromatic light of wavelength 500nm is incident normally on a single slit of width 0.2 nm of produce a diffraction pattern. Find the angular width of the central maximum obtained on the screen.
Estimate the number of fringes obtained in Young's double slit experimental with fringe width 0.5mm, which can be accommodated within the region of total angular spread of the central maximum due to single slit.View SolutionIn the arrangement shown in figure, light of wavelength 6000Å is incident on slits S1 and S2 have been opened such that S3 is the position of first maximum above the central maximum and S4 is the closest position where intensity is same as that of the ligth used, below the central maximum Point O is equidistant from S1 and S2 and O' is equidistant from S3 and S4. Then intensity of inciden light is I0
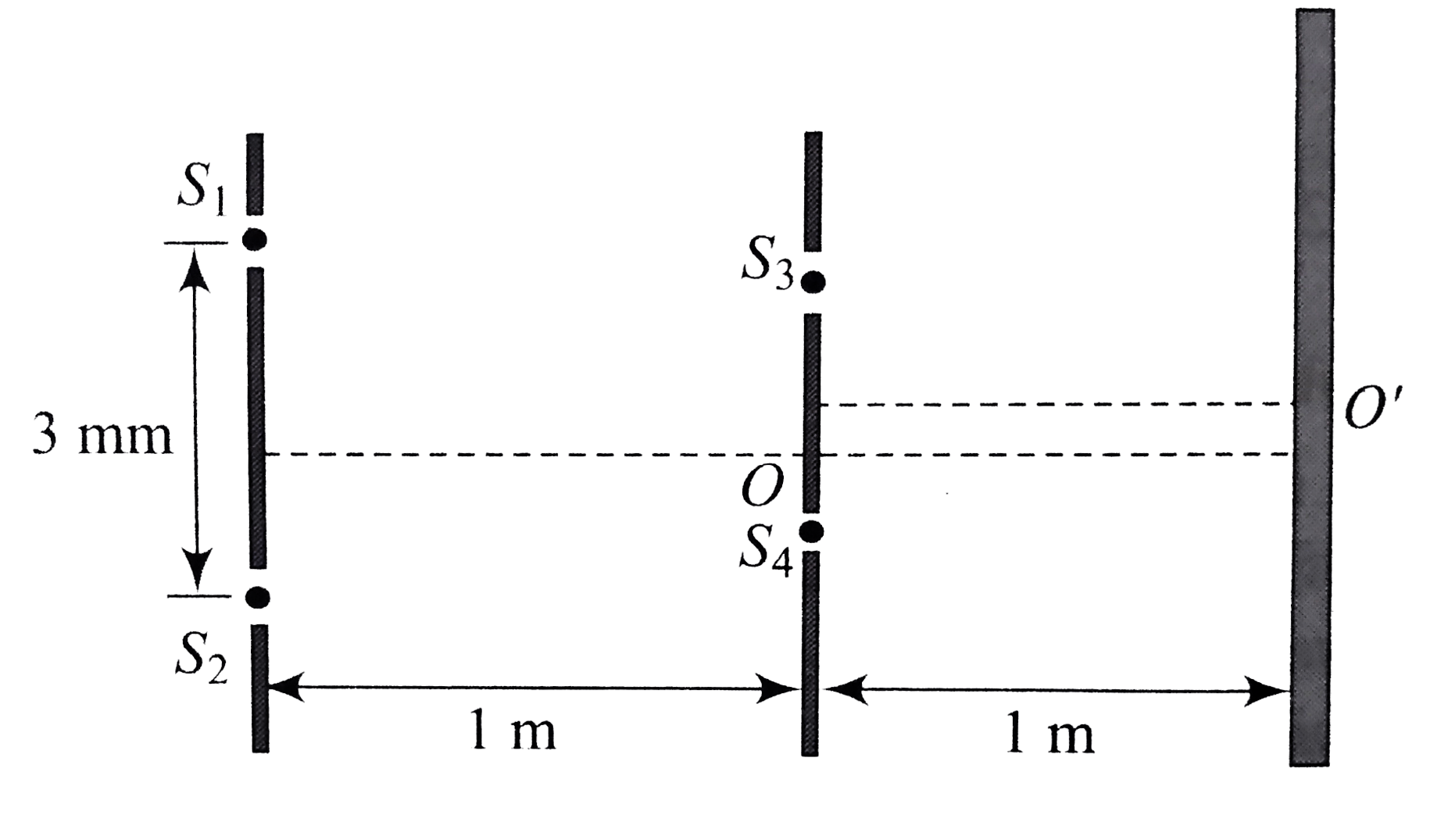
Find the intensity at O' (on the screen).View Solution

