In a fraunhofer's diffraction obtained by a single slit aperture, the value of path difference for nth order of minima is-
nλ
2nλ
(2n−1)λ2
(2n−1)λ
The correct Answer is:A
To determine the path difference for the nth order minima in Fraunhofer diffraction through a single slit, we can follow these steps:
1. Understanding the Setup:
- We have a single slit of width d and we are observing the diffraction pattern on a screen at a distance. The light passing through the slit will spread out and create a pattern of bright and dark fringes.
2. Path Difference Concept:
- For destructive interference (minima) to occur, the path difference between light rays coming from different parts of the slit must be equal to an odd multiple of half the wavelength. This is because we want the waves to cancel each other out.
3. Condition for Minima:
- The condition for the nth order minima can be expressed as:
Path Difference=nλ
where n is an integer (1, 2, 3, ...), and λ is the wavelength of the light.
4. Deriving the Path Difference:
- For a single slit, the path difference for light coming from the top edge of the slit to the bottom edge when viewed at an angle θ is given by:
dsinθ
where d is the width of the slit.
5. Setting Up the Equation:
- For the nth order minima, we set the path difference equal to nλ:
dsinθ=nλ
6. Final Expression:
- Rearranging gives us the condition for the nth order minima:
sinθ=nλd
Conclusion:
The path difference for the nth order minima in Fraunhofer diffraction through a single slit is given by:
dsinθ=nλ
- We have a single slit of width d and we are observing the diffraction pattern on a screen at a distance. The light passing through the slit will spread out and create a pattern of bright and dark fringes.
- For destructive interference (minima) to occur, the path difference between light rays coming from different parts of the slit must be equal to an odd multiple of half the wavelength. This is because we want the waves to cancel each other out.
- The condition for the nth order minima can be expressed as:
Path Difference=nλ
where n is an integer (1, 2, 3, ...), and λ is the wavelength of the light.
- For a single slit, the path difference for light coming from the top edge of the slit to the bottom edge when viewed at an angle θ is given by:
dsinθ
where d is the width of the slit.
- For the nth order minima, we set the path difference equal to nλ:
dsinθ=nλ
- Rearranging gives us the condition for the nth order minima:
sinθ=nλd
The path difference for the nth order minima in Fraunhofer diffraction through a single slit is given by:
dsinθ=nλ
Topper's Solved these Questions
- WAVE OPTICSBOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 2 (Brain Teasures)
22 Videos
- WAVE OPTICSBOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 3 (Miscellaneous type Questions)
27 Videos
- WAVE OPTICSBOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Example 15
1 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (J-A)
7 Videos
Similar Questions
In Fraunhoffer diffraction from a single slit, wave front incident on the slit is
View SolutionIn a single slit diffraction pattern
View SolutionIn the diffractin pattern of a single slit aperture, the width of the central fringe compared to widths of te other fringes is-
View SolutionIn a Fraunhofer diffraction experiment at a single slit using a light of wavelength 400 nm, the first minimum is formed at an angle of 30∘. The direction θ of the first secondary maximum is given by :
View SolutionThe fraunhofer diffraction pattern of a single slit is formed at the focal plane of a lens of focal length 1m. The width of the slit is 0.3 mm. if the third minimum is formed at a distance of 5 mm from the central maximum then calculate the wavelength of light.
View SolutionThe condition for observing Fraunhofer diffraction from a single slit is that the light wavefront incident on the slit should be
View SolutionIn a fraunhofer's diffraction by a slit, if slit width is a, wave length λ focal length of lens is f, linear width of central maxima is-
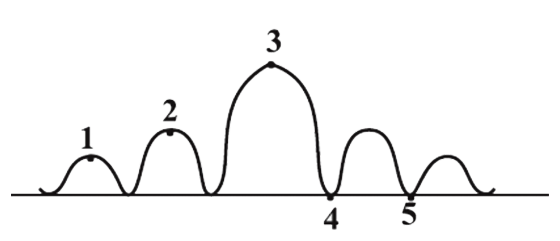
View SolutionThe diffraction pattern of a single slit is shown in the figure. The point at which the path difference of the extreme rays is two times the wavelength is
View SolutionConsider Fraunhoffer diffraction pattern obtained with a single slit illuminated at normal incidence. AT the angular position of the first diffraction minimum the phase difference (in radian) between the wavelengths from the opposite edges of the slit is
View SolutionConsider Fraunhoffer diffraction pattern obtained with a single slit illuminated at normal incidence. At the angular position of the first diffraction minimum the phase difference (in radians) between the wavelets from the opposite edges of the slit is
View Solution
