Object A strikes the stationary object B with a certain give speed u head-on in an elastic collision. The mass of A is fixed, you may only choose the mass of B appropriately for following cases. Then after the collision:
For B to have the greatest speed, choose mB=mA
For B to have the greatest momentum, choose mB<<mA
For B to have the greatest speed, choose mB<<MA
For the maximum fraction of kinetic energy transfer, choose mB=mA
The correct Answer is:(B, C, D)
To solve the problem of an elastic collision between two objects, A and B, we will analyze the situation step by step.
Given:
- Object A strikes stationary object B with speed u.
- Mass of A is mA (fixed).
- Mass of B is mB (to be chosen).
Step 1: Understand the Collision
In an elastic collision, both momentum and kinetic energy are conserved. We need to derive the equations for the final velocities of both objects after the collision.
Step 2: Write the Conservation of Momentum Equation
The conservation of momentum before and after the collision can be expressed as:
mAu+mB⋅0=mAv1+mBv2
Where:
- v1 is the final velocity of A.
- v2 is the final velocity of B.
Step 3: Write the Conservation of Kinetic Energy Equation
The conservation of kinetic energy can be expressed as:
12mAu2+12mB⋅0=12mAv21+12mBv22
Step 4: Simplify the Equations
From the momentum equation, we can simplify:
mAu=mAv1+mBv2(1)
From the kinetic energy equation, we can simplify:
mAu2=mAv21+mBv22(2)
Step 5: Use the Formulas for Final Velocities
For a head-on elastic collision, the final velocities can be derived using the formulas:
v1=mA−mBmA+mBu
v2=2mAmA+mBu
Step 6: Analyze Conditions for Maximum Speed and Kinetic Energy Transfer
1. For B to have the greatest speed:
- We want mB to be much less than mA. This maximizes v2:
v_2 \approx 2u \quad \text{(if m_B \ll m_A )}
2. For maximum kinetic energy transfer:
- Set mB=mA. In this case, A will come to rest, and B will move with speed u:
v1=0andv2=u
3. For B to have the greatest momentum:
- Again, mB should be less than mA. Since momentum p=mv, if v2 is maximized, pB is also maximized.
Conclusion
- For B to have the greatest speed, choose mB≪mA.
- For maximum kinetic energy transfer, choose mB=mA.
- For B to have the greatest momentum, choose mB≪mA.
Final Answer
- The correct choices for the mass of B depend on the specific conditions outlined above.
- Object A strikes stationary object B with speed u.
- Mass of A is mA (fixed).
- Mass of B is mB (to be chosen).
In an elastic collision, both momentum and kinetic energy are conserved. We need to derive the equations for the final velocities of both objects after the collision.
The conservation of momentum before and after the collision can be expressed as:
mAu+mB⋅0=mAv1+mBv2
Where:
- v1 is the final velocity of A.
- v2 is the final velocity of B.
The conservation of kinetic energy can be expressed as:
12mAu2+12mB⋅0=12mAv21+12mBv22
From the momentum equation, we can simplify:
mAu=mAv1+mBv2(1)
From the kinetic energy equation, we can simplify:
mAu2=mAv21+mBv22(2)
For a head-on elastic collision, the final velocities can be derived using the formulas:
v1=mA−mBmA+mBu
v2=2mAmA+mBu
1. For B to have the greatest speed:
- We want mB to be much less than mA. This maximizes v2:
v_2 \approx 2u \quad \text{(if m_B \ll m_A )}
- Set mB=mA. In this case, A will come to rest, and B will move with speed u:
v1=0andv2=u
- Again, mB should be less than mA. Since momentum p=mv, if v2 is maximized, pB is also maximized.
- For B to have the greatest speed, choose mB≪mA.
- For maximum kinetic energy transfer, choose mB=mA.
- For B to have the greatest momentum, choose mB≪mA.
- The correct choices for the mass of B depend on the specific conditions outlined above.
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-I40videos
CENTRE OF MASS
- BOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II43videos
CENTRE OF MASS
- BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON11videos
BASIC MATHEMATICS USED IN PHYSICS &VECTORS
- BOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)3videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
Similar Questions
A particle A suffers an oblique elastic collision particle B that is at rest initially. If their masses with a are the same, then after the collision
View SolutionConsider the elastic collision f two bodies A and B of equal mass. Initially B is at rest and A moves with velocity v. After the collision -
View SolutionBall A of mass m strickes a stationary ball B of mass M and undergoes an elastic collision. After collision bill A has a speed one third of its initial speed. The ratio of M/m is
View SolutionA body of mass M moving with a speed u has a ‘head on’, perfectly elastic collision with a body of mass m initially at rest. If M>>m , the speed of the body of mass m after collision, will be nearly :
View SolutionA moving body of mass m makes a head on elastic collision with another body of mass 2m which is initially at rest. Find the fraction of kinetic energy lost by the colliding particles after collision.
View SolutionAn object A of mass m with initial velocity u collides with a statinary object B after elestic collision A moves with u4 calculate mass of B.
View SolutionParticle A makes a head on elastic collision with another stationary particle B. They fly apart in opposite directions with equal speeds. The mass ratio will be
View SolutionA body of mass m1 moving at a constant speed undergoes an elastic head on collision with a body of mass m2 initially at rest. The ratio of the kinetic energy of mass m1 after the collision to that before the collision is -
View SolutionA particle (a mud pallet, say) of mass m strikes a smooth stationary wedge of mass M with as velocity v0 at an angle θ with horizontal. If the collision is perfectly inelastic, find the
a. velocity of the wedge just after the collision.
b. Change in KE of the system (M+m) in collision.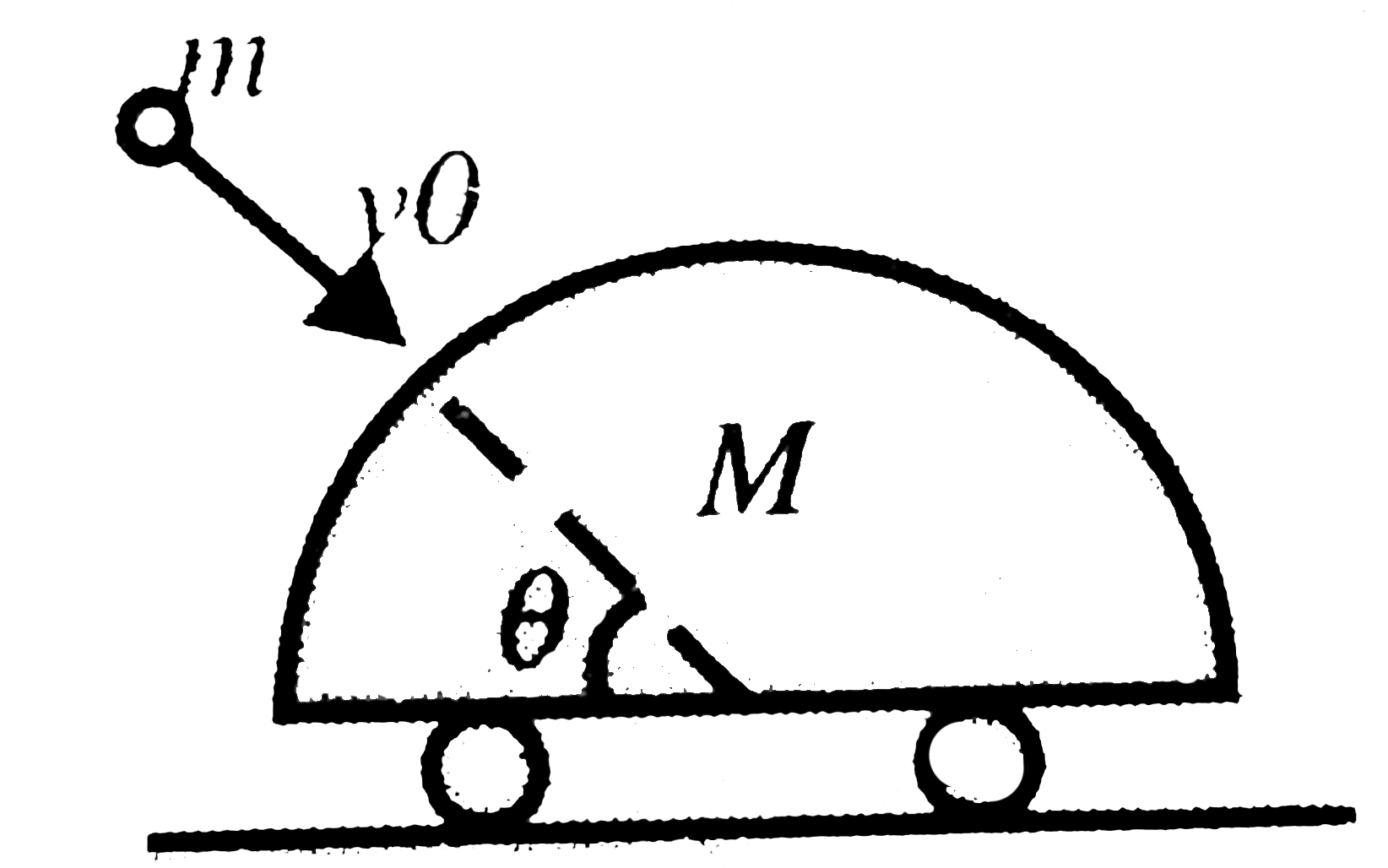 View Solution
View SolutionA particle of mass m0, travelling at speed v0. Strikes a stationary particle of mass 2m0. As a result of the particle of mass m0 is deflected through 45∘ and has a final speed of v0√2. Then the speed of the particle of mass 2m0 after this collision is
View Solution

