A particle moving with kinetic energy makes an elastic head-on collision with a stationary particle which has twice its mass. During the impact :-
the minimum kinetic energy of the system is
the change in elastic potential energy of the system is
momentum and total energy are conserved at every instant.
the ratio of kinetic energy to potential energy of the system first decreases and then increases.
The correct Answer is:A, B, C, D
To solve the problem step by step, we will analyze the elastic collision between two particles, one of which is moving and the other is stationary.
Step 1: Understand the Given Information
- A particle with kinetic energy KE1=3J is moving.
- The mass of this particle is m.
- The second particle, which is stationary, has a mass of 2m.
- We need to analyze the situation during the elastic collision.
Step 2: Calculate the Initial Velocity of the Moving Particle
The kinetic energy of the moving particle is given by the formula:
KE=12mv2
Setting this equal to the given kinetic energy:
12mu2=3
From this, we can solve for u2:
u2=6m
Thus, the initial velocity u of the first particle is:
u=√6m
Step 3: Apply Conservation of Momentum
In an elastic collision, momentum is conserved. The initial momentum of the system is:
pinitial=mu+2m⋅0=mu
Let v1 be the final velocity of the first particle and v2 be the final velocity of the second particle. The momentum conservation equation becomes:
mu=mv1+2mv2
Dividing through by m:
u=v1+2v2(1)
Step 4: Apply Conservation of Kinetic Energy
In an elastic collision, kinetic energy is also conserved. Thus:
KEinitial=KEfinal
12mu2=12mv21+12(2m)v22
Simplifying gives:
u2=v21+2v22(2)
Step 5: Solve the System of Equations
We have two equations (1) and (2):
1. u=v1+2v2
2. u2=v21+2v22
Substituting v1=u−2v2 from equation (1) into equation (2):
u2=(u−2v2)2+2v22
Expanding the equation:
u2=u2−4uv2+4v22+2v22
This simplifies to:
0=−4uv2+6v22
Factoring out v2:
v2(6v2−4u)=0
Thus, either v2=0 (which is not possible since the second particle moves after the collision) or:
6v2=4u⟹v2=2u3
Step 6: Find v1
Substituting v2 back into equation (1):
u=v1+2(2u3)
This gives:
u=v1+4u3
Rearranging:
v1=u−4u3=−1u3
Step 7: Calculate the Kinetic Energies After Collision
The kinetic energy of the first particle after the collision:
KE′1=12mv21=12m(−u3)2=12mu29=12m6m⋅9=13J
The kinetic energy of the second particle after the collision:
KE′2=12(2m)v22=m(2u3)2=m4u29=m4⋅69m=83J
Step 8: Total Kinetic Energy After Collision
The total kinetic energy after the collision is:
KE′total=KE′1+KE′2=13+83=3J
Conclusion
The minimum kinetic energy of the system is 1J and the change in the elastic potential energy is 2J.
- A particle with kinetic energy KE1=3J is moving.
- The mass of this particle is m.
- The second particle, which is stationary, has a mass of 2m.
- We need to analyze the situation during the elastic collision.
The kinetic energy of the moving particle is given by the formula:
KE=12mv2
Setting this equal to the given kinetic energy:
12mu2=3
From this, we can solve for u2:
u2=6m
Thus, the initial velocity u of the first particle is:
u=√6m
In an elastic collision, momentum is conserved. The initial momentum of the system is:
pinitial=mu+2m⋅0=mu
Let v1 be the final velocity of the first particle and v2 be the final velocity of the second particle. The momentum conservation equation becomes:
mu=mv1+2mv2
Dividing through by m:
u=v1+2v2(1)
In an elastic collision, kinetic energy is also conserved. Thus:
KEinitial=KEfinal
12mu2=12mv21+12(2m)v22
Simplifying gives:
u2=v21+2v22(2)
We have two equations (1) and (2):
1. u=v1+2v2
2. u2=v21+2v22
u2=(u−2v2)2+2v22
Expanding the equation:
u2=u2−4uv2+4v22+2v22
This simplifies to:
0=−4uv2+6v22
Factoring out v2:
v2(6v2−4u)=0
Thus, either v2=0 (which is not possible since the second particle moves after the collision) or:
6v2=4u⟹v2=2u3
Substituting v2 back into equation (1):
u=v1+2(2u3)
This gives:
u=v1+4u3
Rearranging:
v1=u−4u3=−1u3
The kinetic energy of the first particle after the collision:
KE′1=12mv21=12m(−u3)2=12mu29=12m6m⋅9=13J
The kinetic energy of the second particle after the collision:
KE′2=12(2m)v22=m(2u3)2=m4u29=m4⋅69m=83J
The total kinetic energy after the collision is:
KE′total=KE′1+KE′2=13+83=3J
The minimum kinetic energy of the system is 1J and the change in the elastic potential energy is 2J.
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-III42videos
CENTRE OF MASS
- BOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-IV A32videos
CENTRE OF MASS
- BOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-I40videos
CENTRE OF MASS
- BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON11videos
BASIC MATHEMATICS USED IN PHYSICS &VECTORS
- BOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)3videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
Similar Questions
A particle moving with kinetic energy E makes a head on elastic collision with an identical particle at rest. During the collision
View SolutionA particle moving with kinetic energy K makes a head on elastic collision with an identical particle at rest. Find the maximum elastic potential energy of the system during collision.
View SolutionA particle of mass m moving with velocity v makes head-on elastic collision with a sationary particle of mass 2m. Kinetic energy lost by the lighter particle during period of deformation is
View Solutionparticle of mass m, moving with velocity 'u' makes head on collision with identical stationary particle, which is elastic. Theforce of interaction increases to maximum and then becomes zero in time 'T' as shown in graph. What is the maximum value F0 of interaction force ?
 View Solution
View SolutionAn α-particle with a kinetic energy of 2.1eV makes a head on collision with a hydrogen atom moving towards it with a kinetic energy of 8.4eV. The collision
View SolutionA neutron makes a head-on elastic collision with a stationary deuteron. The fraction energy loss of the neutron in the collision is
View SolutionA neutron makes a head-on elastic collision with a stationary deuteron. The fraction energy loss of the neutron in the collision is
View SolutionA particle of mass m1 experiences a perfectlly elastic collision with a stationary particle of mass m2. Match the entries in column I to all the entries in column II.
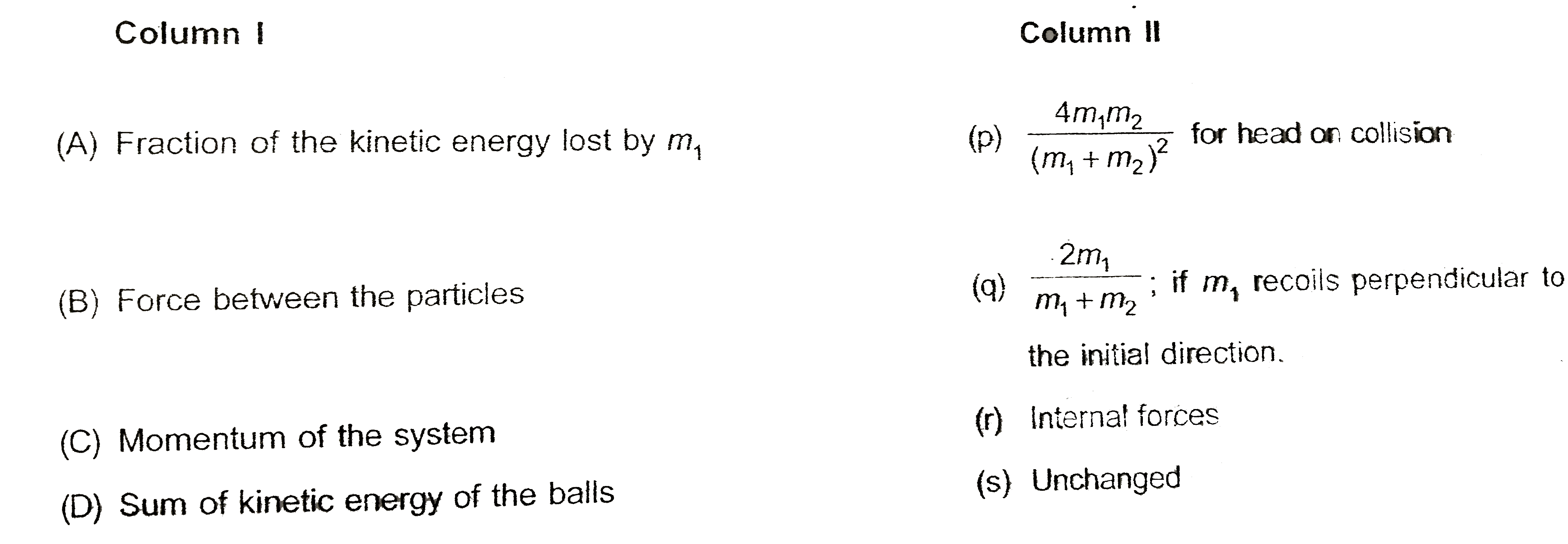 View Solution
View SolutionA particle of mass m travelling with velocity v and kinetic energy E collides elastically to another particle of mass nm, at rest. What is the fraction of total energy retained by the particle of mass m?
View SolutionParticle A makes a head on elastic collision with another stationary particle B. They fly apart in opposite directions with equal speeds. The mass ratio will be
View Solution

