A particle of mass m, kinetic energy K and momentum p collides head on elastically with another of mass 2 m at rest. Match the following columns after collision.)
column1column2A momentum of first particleP 3/4 pB Momentum of second particleQ -K/9C Kinetic energy of first particleR -p/3D Kinetic energy of second particleS 8K/9
The correct Answer is:(A) → r; (B) → t; (C ) → t; (D) → s
By applying conservation of momentum
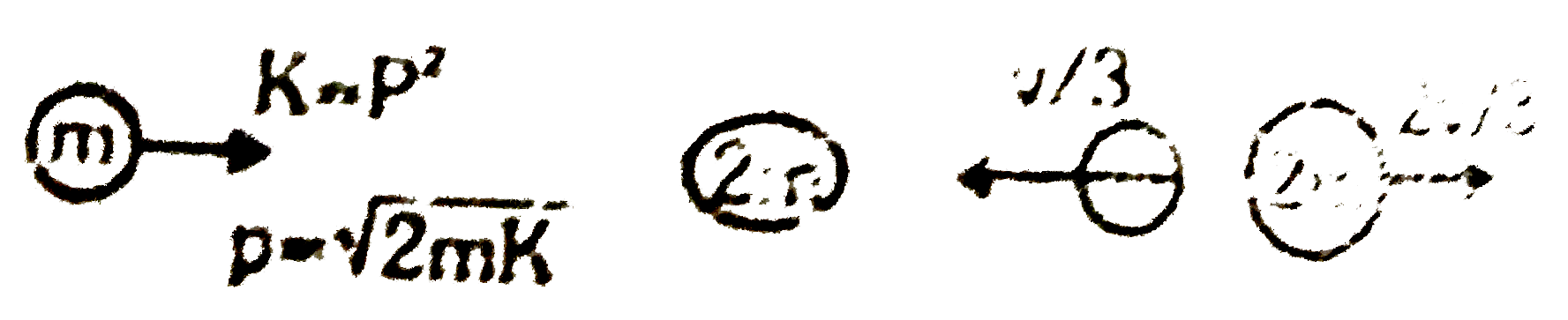
m1u1+2m(0)=mv1+2mv2 …..(i)
Also u=v2−v1 …….(ii)
v2=2v3 and v1=−v3,F2=4mv3=4p3,
P1=−mv3=−P3,K2=8K9,K1=K9
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-IV A
32 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-IV B
14 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II
43 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
A particle of mass m, kinetic energy K and momentum p collides head on elastically with another particle of mass 2m at rest. After collision, :
Column IColumn II(A)Momentum of first particle(p)3/4p(B)Momentum of second particle(q)−K/9(C)Kinetic energy of first particle(r)−p/3(D)Kinetic energy of second particle(s)8K9(t)None
View SolutionA particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 2m at rest. Find loss of kinetic energy of system in the collision.
View SolutionA particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 2m at rest. Find loss of kinetic energy of system in the collision.
View SolutionA particle of mass m moving with speed V collides elastically with another particle of mass 2m. Find speed of smaller mass after head on collision
View SolutionA particle of mass m has momentum p. Its kinetic energy will be
View SolutionMatch the following: (P = momentum of particle, K = kinetic energy of particle)
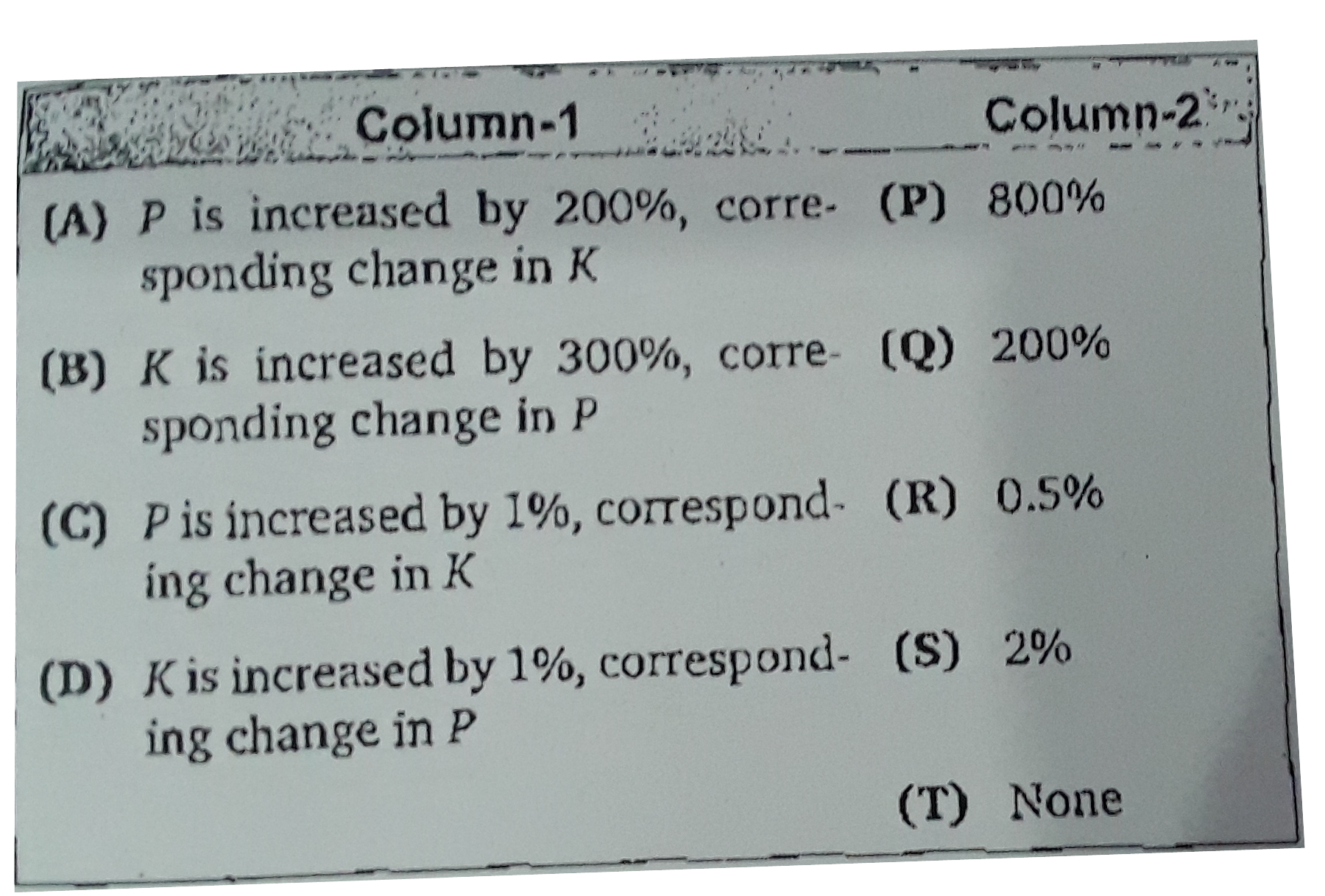
View SolutionMatch the following: (P = momentum of particle, K = kinetic energy of particle)

View SolutionA particle of mass m1 experiences a perfectlly elastic collision with a stationary particle of mass m2. Match the entries in column I to all the entries in column II.
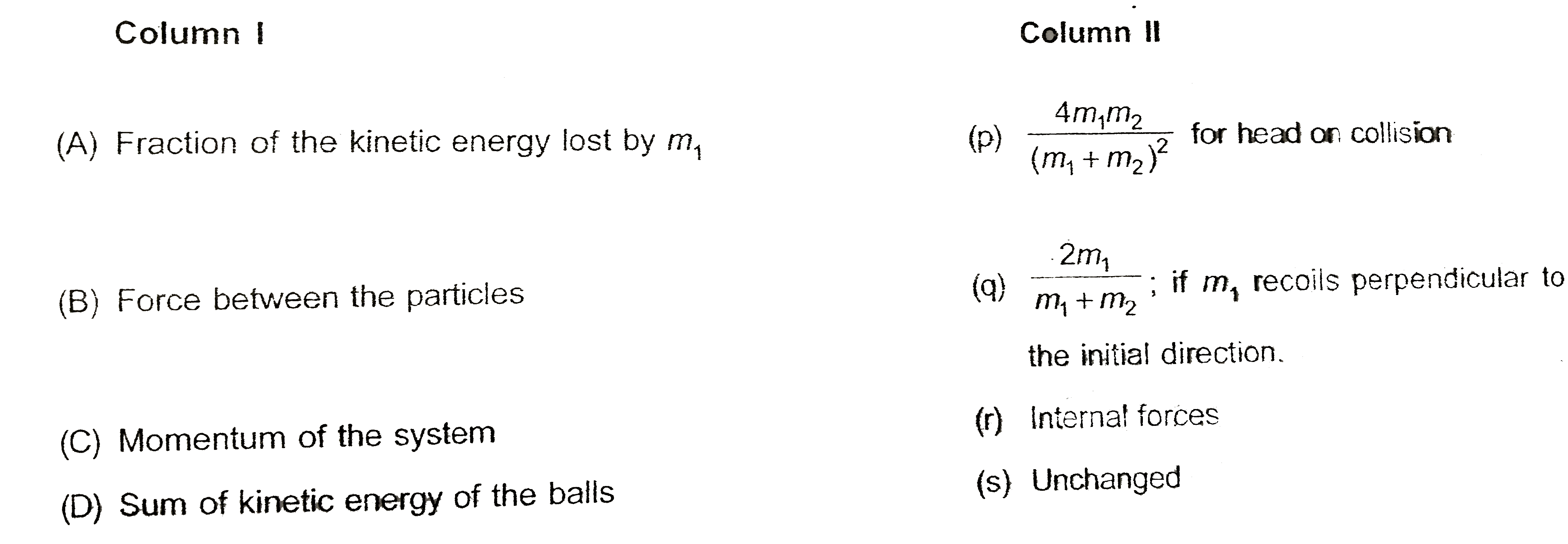
View SolutionA particle of mass m travelling with velocity v and kinetic energy E collides elastically to another particle of mass nm, at rest. What is the fraction of total energy retained by the particle of mass m?
View SolutionA particle moving with kinetic energy K makes a head on elastic collision with an identical particle at rest. Find the maximum elastic potential energy of the system during collision.
View Solution
