A uniform wire of length l carrying current radiates energy at a rate P to environment while its temperature remains the same. Magnitude of magnetic flux density on the surface of wire is B and its diameter is d. If electric field strenght in wire is given by , then find n.
The correct Answer is:1
To solve the problem, we need to find the value of n in the given equation for electric field strength E. Let's break down the steps systematically.
Step 1: Understand the given parameters
We have a uniform wire of length l carrying a current I which radiates energy at a rate P. The magnetic flux density on the surface of the wire is B, and the diameter of the wire is d.
Step 2: Relate power, electric field, and current
The power P radiated by the wire can be expressed in terms of electric field E and current I:
P=E⋅I⋅l
Step 3: Express current in terms of magnetic flux density
The magnetic field B around a long straight wire carrying current I is given by:
B=μ0I2πr
For a wire of diameter d, the radius r is d2. Therefore, we can express B as:
B=μ0Iπd
From this, we can solve for the current I:
I=Bπdμ0
Step 4: Substitute current into the power equation
Substituting I back into the power equation:
P=E⋅(Bπdμ0)⋅l
Rearranging gives:
E=Pμ0Bπdl
Step 5: Compare with the given electric field equation
We are given that:
E=nμ0PBπld
Now we can set the two expressions for E equal to each other:
Pμ0Bπdl=nμ0PBπld
Step 6: Solve for n
Since μ0, P, B, π, l, and d are common in both sides of the equation, we can cancel them out:
1=n
Thus, we find:
n=1
Final Answer
The value of n is 1.
---
We have a uniform wire of length l carrying a current I which radiates energy at a rate P. The magnetic flux density on the surface of the wire is B, and the diameter of the wire is d.
The power P radiated by the wire can be expressed in terms of electric field E and current I:
P=E⋅I⋅l
The magnetic field B around a long straight wire carrying current I is given by:
B=μ0I2πr
For a wire of diameter d, the radius r is d2. Therefore, we can express B as:
B=μ0Iπd
From this, we can solve for the current I:
I=Bπdμ0
Substituting I back into the power equation:
P=E⋅(Bπdμ0)⋅l
Rearranging gives:
E=Pμ0Bπdl
We are given that:
E=nμ0PBπld
Now we can set the two expressions for E equal to each other:
Pμ0Bπdl=nμ0PBπld
Since μ0, P, B, π, l, and d are common in both sides of the equation, we can cancel them out:
1=n
Thus, we find:
n=1
The value of n is 1.
Topper's Solved these Questions
- TEST PAPERSBook:ALLENChapter:TEST PAPERSExercise:part-2 physic
Explore 48 Videos
- TEST PAPERSBook:ALLENChapter:TEST PAPERSExercise:PHYSICS
Explore 17 Videos
- TEST PAPERSBook:ALLENChapter:TEST PAPERSExercise:Part-1 Physics
Explore 15 Videos
- TEST PAPER 4Book:ALLENChapter:TEST PAPER 4Exercise:PHYSICS
Explore 44 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR Book:ALLENChapter:UNIT & DIMENSIONS, BASIC MATHS AND VECTOR Exercise:Exercise (J-A)
Explore 7 Videos
Similar Questions
A wire of length L carries a current i along the x-axis. A magnetic field B=B0(ˆi+ˆj+ˆk) exists in the space. Find the magnitude of the magnetic force acting on the wire.
View SolutionA wire of length Lmetre , carrying a current Iampere is bent in the form of a circle . The magnitude of its magnetic moment is …………. MKSunits .
View SolutionA wire of length l carries a current I along the x-asis. A magnetic field exists which is given as →B=B0(→i+→j+→k) T. find the magnitude of the magnetic force acting on the wire.
View SolutionAn infinitely long wire carrying current I is along Y-axis such taht its one end is at point A(0,b) while the wire extends upto +∞. The magnitude of magnetic field strength at point (a,0) is
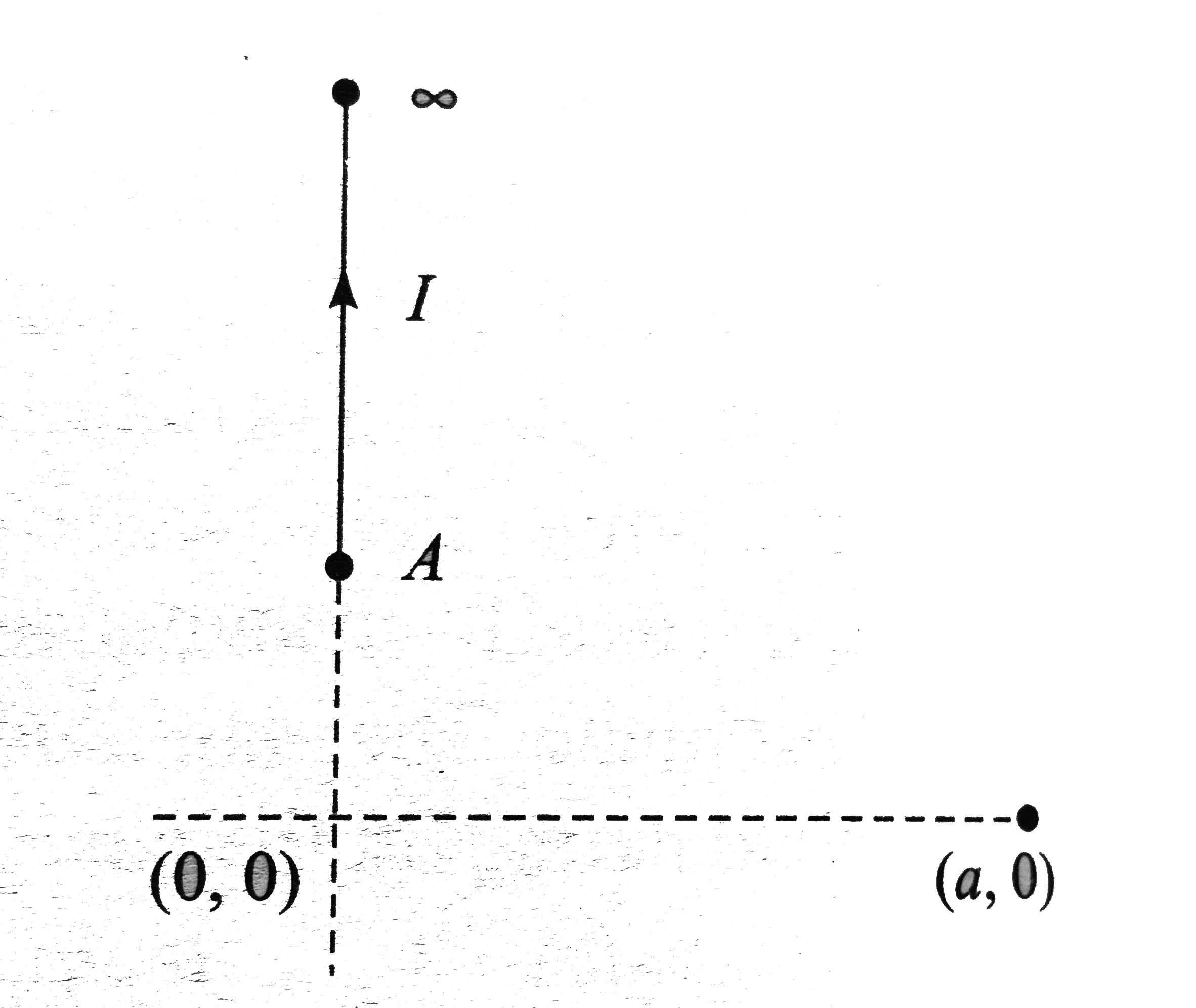
View SolutionAn infinitely long wire carrying current I is along Y-axis such taht its one end is at point A(0,b) while the wire extends upto +∞. The magnitude of magnetic field strength at point (a,0) is

View SolutionAn infinitely long wire carrying current I is along Y-axis such taht its one end is at point A(0,b) while the wire extends upto +∞. The magnitude of magnetic field strength at point (a,0) is

View SolutionMagnetic field at the centre of a rectangle of length l& width d carrying a current i is given by nμ0iπd then find value of N (here l>>d)
View SolutionA straight wire of mass 400 g and length 2m carries a current of 2A. It is suspended in mid air by a uniform horizontal field B. What is the magnitude of the magnetic field ?
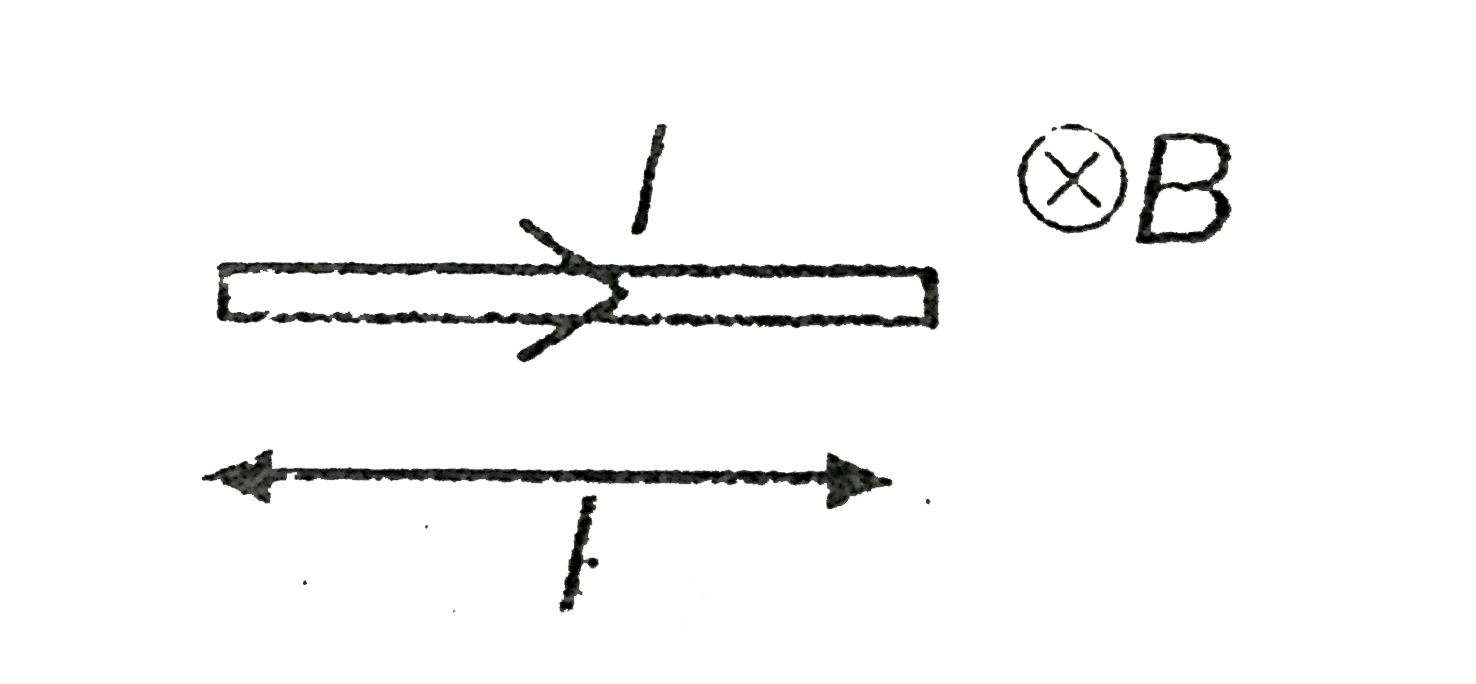
View SolutionA straight wire of mass 400 g and length 2m carries a current of 2A. It is suspended in mid air by a uniform horizontal field B. What is the magnitude of the magnetic field ?

View SolutionFind the magnitude and direction of magnetic field at point P due to the current carrying wire as shown in

View Solution