Assertion:− A clothline hangs between two poles. No matter how tightly the line is stretched, it always sags a little at the centre.
Reason :− In above case, horizontal stiring cannot balance vertical weight.
If both Assertion & Reason are True & the Reason is a correct explanation of the Assertion.
If both Assertion & Reason are True but Reason is not a correct explanation of the Assertiion.
If Assertion is True but the Reason is False.
If both Assertion & Reason are False
The correct Answer is:A
To solve the problem, we need to analyze the assertion and reason provided in the question.
1. Understanding the Assertion: The assertion states that a clothline hangs between two poles and always sags a little at the center, regardless of how tightly it is stretched. This sagging occurs due to the weight of the cloth or any object hanging on the line.
Hint: Consider the forces acting on the clothline and how they interact.
2. Analyzing the Forces: When a clothline is stretched tightly, it experiences tension. However, the weight of the cloth hanging on the line creates a downward force. The tension in the line has both horizontal and vertical components.
Hint: Visualize the tension in the line and how it is distributed.
3. Components of Tension: The tension (T) in the clothline can be resolved into two components: a horizontal component (T cos θ) and a vertical component (T sin θ). The vertical component is responsible for balancing the weight of the cloth.
Hint: Remember that the vertical component must balance the weight for the line to remain straight.
4. Balancing Forces: For the clothline to be in equilibrium, the total upward force (the vertical components of tension) must equal the downward force (the weight of the cloth). This can be expressed as:
2Tsinθ=W
where W is the weight of the cloth.
Hint: Think about how the angle θ affects the vertical component of the tension.
5. Conclusion on the Assertion: Since the tension in a perfectly horizontal string would have no vertical component, it cannot balance the weight of the cloth. Thus, the assertion that the clothline always sags is true.
Hint: Reflect on why the horizontal tension alone cannot support the weight.
6. Understanding the Reason: The reason provided states that in the case of a horizontal string, it cannot balance the vertical weight. This is correct because, without a vertical component of tension, the weight would not be supported.
Hint: Consider how the angle of the string affects the vertical support.
Final Conclusion:
Both the assertion and the reason are true. The assertion correctly describes the behavior of the clothline, and the reason explains why this behavior occurs.
Summary:
- The assertion is true: A clothline always sags due to the weight of the cloth.
- The reason is true: A horizontal string cannot balance the vertical weight without a vertical component of tension.
2Tsinθ=W
where W is the weight of the cloth.
Both the assertion and the reason are true. The assertion correctly describes the behavior of the clothline, and the reason explains why this behavior occurs.
- The assertion is true: A clothline always sags due to the weight of the cloth.
- The reason is true: A horizontal string cannot balance the vertical weight without a vertical component of tension.
Topper's Solved these Questions
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - Exercise (Physics)
330 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Video
- TEST PAPER 1BOOK - ALLENCHAPTER - TEST PAPER 1EXERCISE - PHYSICS
11 Videos
Similar Questions
Assertion : Distance between two particles moving with consant velocities always remains constant.
Reason : In the above case, relative motion between them is uniform.
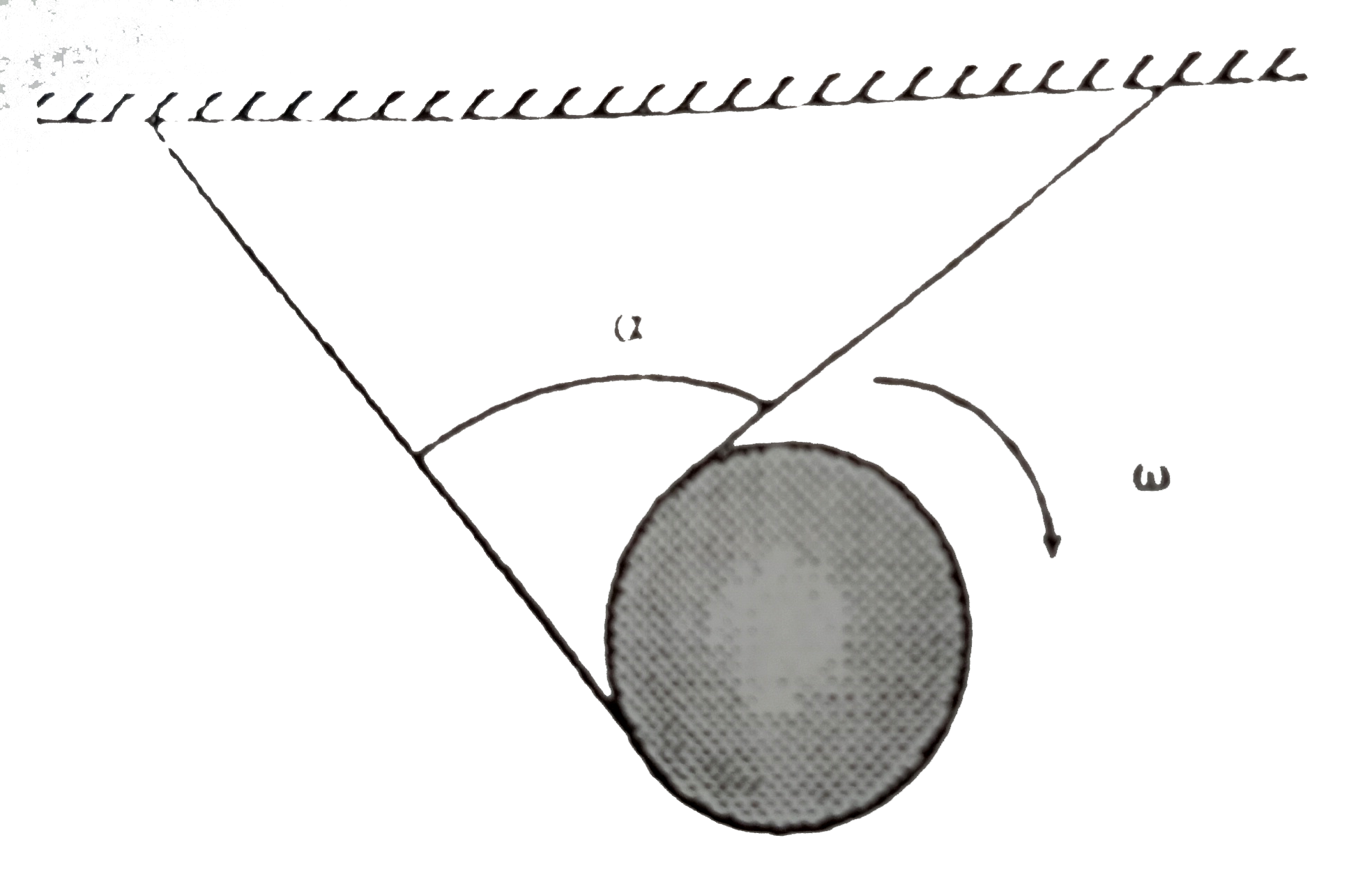
View SolutionA heavy disc with radius R is rolling down hanging on two non-stretched string wound around the disc very tightly. The free ends of the string are attached to a fixed horizontal support. The strings are always tensed during the motion. At some instant, the angular velocity of the disc is ω, and the angle between the strings is α. Find the velocity of centre of mass of the disc at this moment
View SolutionA wire of cross section A is stretched horizontally between two clamps located 2lm apart. A weight Wkg is suspended from the mid-point of the wire. If the mid-point sags vertically through a distance x<l, the strain produced is
View SolutionA circular ring of radius 3cm hangs horizontally from a point 4 cm vertically above the centre by 4 strings attached at equal intervals to its circumference. If the angle between two consecutive strings be θ , then cosθ is equal to (A)45 (B) 425 (C) 1625 (D) none of these
View SolutionA circular ring of radius 3cm hangs horizontally form a point 4cm vertically above the centre by 4 strings attached at equal intervals to its circumference. If the angle between two consecutive strings be θ , then cosθ is equal to 45 (b) 425 (d) 1625 (d) none of these
View SolutionWrite the names given to the vertical columns and horizontal rows in the Modern Periodic Table. How does the metallic charcter of elements very on moving down a vertical column ? How does the size of atomic radius vary on moving left to right in a horizontal row ? Give reason in pupport of your answer in the above two cases.
View Solution