A particle of mass m rests on a horizontal floor with which it has a coefficient of static friction μ. It is desired to make the body move by applying the minimum possible force F. Find the magnitude of F and the direction in which it has to be applied.
tan−1μ,μmg√(1+μ2)
tan−1μ,mg√(1+μ2)
tan−1μ,μmg√(1−μ2)
tan−1μ,mg√1−μ2
The correct Answer is:A
To solve the problem of finding the minimum force F required to move a particle of mass m resting on a horizontal floor with a coefficient of static friction μ, we will follow these steps:
Step 1: Identify the Forces Acting on the Particle
The forces acting on the particle include:
- The gravitational force mg acting downward.
- The normal force N acting upward.
- The applied force F at an angle θ to the horizontal.
- The frictional force f acting opposite to the direction of motion.
Step 2: Resolve the Applied Force into Components
The applied force F can be resolved into two components:
- Horizontal component: Fcosθ
- Vertical component: Fsinθ
Step 3: Write the Equation for the Normal Force
Since there is no vertical motion, the net force in the vertical direction is zero. Therefore, we can write:
N+Fsinθ=mg
Rearranging gives us:
N=mg−Fsinθ(1)
Step 4: Write the Equation for the Frictional Force
The frictional force f can be expressed in terms of the normal force:
f=μN
Substituting equation (1) into this gives:
f=μ(mg−Fsinθ)(2)
Step 5: Set Up the Equation for Horizontal Forces
For the particle to just start moving, the horizontal component of the applied force must equal the frictional force:
Fcosθ=f
Substituting equation (2) into this gives:
Fcosθ=μ(mg−Fsinθ)
Step 6: Rearrange the Equation
Rearranging the equation gives:
Fcosθ+μFsinθ=μmg
Factoring out F:
F(cosθ+μsinθ)=μmg
Thus, we can solve for F:
F=μmgcosθ+μsinθ(3)
Step 7: Minimize the Force F
To find the minimum force, we need to maximize the denominator cosθ+μsinθ. We can use calculus to find the maximum by differentiating:
ddθ(cosθ+μsinθ)=−sinθ+μcosθ=0
This gives:
μ=tanθ⇒θ=tan−1(μ)
Step 8: Substitute Back to Find F
Now substituting θ=tan−1(μ) into equation (3):
- Calculate cosθ and sinθ:
sinθ=μ√1+μ2,cosθ=1√1+μ2
Substituting these values into the denominator:
cosθ+μsinθ=1√1+μ2+μ⋅μ√1+μ2=1+μ2√1+μ2=√1+μ2
Thus, substituting back into equation (3):
F=μmg√1+μ2(4)
Final Result
The magnitude of the minimum force F required to move the particle is:
F=μmg√1+μ2
The forces acting on the particle include:
- The gravitational force mg acting downward.
- The normal force N acting upward.
- The applied force F at an angle θ to the horizontal.
- The frictional force f acting opposite to the direction of motion.
The applied force F can be resolved into two components:
- Horizontal component: Fcosθ
- Vertical component: Fsinθ
Since there is no vertical motion, the net force in the vertical direction is zero. Therefore, we can write:
N+Fsinθ=mg
Rearranging gives us:
N=mg−Fsinθ(1)
The frictional force f can be expressed in terms of the normal force:
f=μN
Substituting equation (1) into this gives:
f=μ(mg−Fsinθ)(2)
For the particle to just start moving, the horizontal component of the applied force must equal the frictional force:
Fcosθ=f
Substituting equation (2) into this gives:
Fcosθ=μ(mg−Fsinθ)
Rearranging the equation gives:
Fcosθ+μFsinθ=μmg
Factoring out F:
F(cosθ+μsinθ)=μmg
Thus, we can solve for F:
F=μmgcosθ+μsinθ(3)
To find the minimum force, we need to maximize the denominator cosθ+μsinθ. We can use calculus to find the maximum by differentiating:
ddθ(cosθ+μsinθ)=−sinθ+μcosθ=0
This gives:
μ=tanθ⇒θ=tan−1(μ)
Now substituting θ=tan−1(μ) into equation (3):
- Calculate cosθ and sinθ:
sinθ=μ√1+μ2,cosθ=1√1+μ2
Substituting these values into the denominator:
cosθ+μsinθ=1√1+μ2+μ⋅μ√1+μ2=1+μ2√1+μ2=√1+μ2
Thus, substituting back into equation (3):
F=μmg√1+μ2(4)
The magnitude of the minimum force F required to move the particle is:
F=μmg√1+μ2
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-II
112 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - SWOE
44 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)
4 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
24 Videos
Similar Questions
A train starting from rest is moving along a straight track with a constant acceleration fo 2.5m/s2. A passenger at rest in the train observes a particle of mass 2kg to be at rest on the floor with which it has a coefficient of friction μs=μk=0.5 .Six seconds after the starting of the train , a horizontal force F = 13N is applied to the particle for two seconds duration. The passenger now observes the particle to move perpendicular to the direction of the train.
(a) calculate the kinetic energy of the particle with respect to the passenger at the end of 8 seconds after starting of the train.
( b) repeat the calculate of ( a) for an observer on the ground.
View SolutionA crate of mass 100 kg rests on the floor. The coefficient of static friction is 0.4. If a force of 250 N(parallel to the floor) is applied to the create, what's the magnitude of the force of static friction on the crate ?
View Solution
Knowledge Check
A crate of mass 100 kg is at rest on a horizontal floor. The coefficient of static friction between the crate and the floor is 0.4, and the coefficient of kinetic friction is 0.3 . A force F of magnitude 344 N is then applied to the crate, parallel to the floor. Which of the following is true ?
AThe crate will accelerate across the floor at 0.5m/s2.BThe static friction force, which is the reaction force to F as guaranteed by Newton 's Third Law, will also have a magnitude of 344 N.CThe crate will slide across the floor at a constant speed of 0.5m/s.DThe crate will not move.
A block of mass m is kept on a horizontal table. If the static friction coefficient is μ., find the frictional force acting on the block.
View SolutionA block of mass M is kept on as rough horizontal surface. The coefficient of static frictionbetween the block and the surface is μ. The block is to be pulled by applying a force to it. What minimum force is needed to slide the block? In which direction should this force act?
View SolutionA block of mass M is kept on as rough horizontal surface. The coefficient of static friction between the block and the surface is μ. The block is to be pulled by applying a force to it. What minimum force is needed to slide the block? In which direction should this force act?
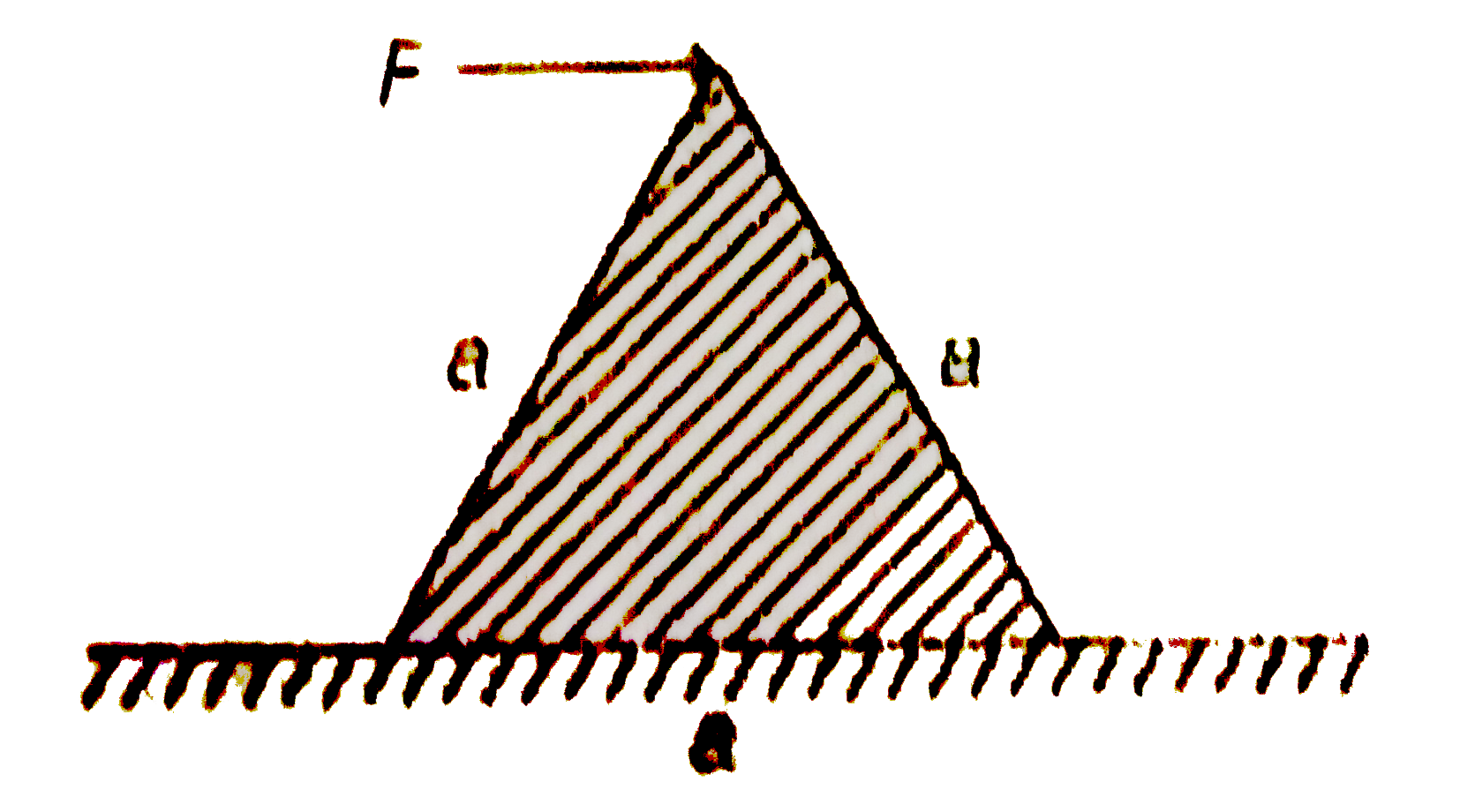
View SolutionAn equilateral prism of mass m rests on a rough horizontal surface with cofficent of friction μ. A horizontal force F is applied on the prism as shown in the figure. If the cofficent of the friction is sufficently high so that the prism does not slide before toppling, then the minimum force required to topple the prism is
View SolutionA homogeneous block rests against a vertical wall for which the coefficient of friction is 1/2. A force 'F' is applied to the block in the direction as shown in figure. Find the maximum magnitude of applied force 'F' such that block remains in rest.

View SolutionA box of mass 200 kg is kept on a rough horizontal floor having coefficient of static friction μs=0.25 and coefficient kinetic friction μk=0.2. Find the work done by a man to slide the body slowly through 20 m with a minimum force. Also find the magnitude of the minimum force.
View SolutionA block of mass 2 kg is kept on a rough horizontal floor an pulled with a force F. If the coefficient of friction is 0.5. then the minimum force required to move the block is :-

View Solution
