A 150 g tenins ball coming at a speed of 40m/s is hit straight back by a bat to speed of 60m/s. The magnitude of the average force F on the ball, when it is in contact for 5ms is,
2500N
3000N
3500N
4000N
The correct Answer is:B
To solve the problem, we need to calculate the average force exerted on the tennis ball during the time it is in contact with the bat. We will use the formula for average force, which is given by the change in momentum divided by the time duration of contact.
1. Convert Mass to Kilograms:
The mass of the tennis ball is given as 150 grams. We need to convert this to kilograms since the standard unit of mass in physics is kilograms.
Mass=150g=0.150kg
Hint: Remember to convert grams to kilograms by dividing by 1000.
2. Identify Initial and Final Velocities:
The initial velocity u of the ball is given as 40 m/s (towards the bat), and since it is hit back, we consider this direction as negative. Therefore:
u=−40m/s
The final velocity v after being hit back is 60 m/s (in the opposite direction):
v=60m/s
Hint: Pay attention to the direction of the velocities; assign negative or positive signs accordingly.
3. Calculate Change in Momentum:
The change in momentum Δp is given by:
Δp=m(v−u)
Substituting the values:
Δp=0.150kg×(60m/s−(−40m/s))
Δp=0.150kg×(60+40)m/s
Δp=0.150kg×100m/s=15kg m/s
Hint: Remember that the change in momentum considers both the final and initial velocities.
4. Convert Time to Seconds:
The time duration Δt during which the ball is in contact with the bat is given as 5 milliseconds. We need to convert this to seconds:
Δt=5ms=5×10−3s
Hint: Milliseconds can be converted to seconds by multiplying by 10−3.
5. Calculate Average Force:
The average force F can now be calculated using the formula:
F=ΔpΔt
Substituting the values we have:
F=15kg m/s5×10−3s=3000N
Hint: The average force is directly proportional to the change in momentum and inversely proportional to the time duration.
6. Final Answer:
The magnitude of the average force F on the ball is:
F=3000N
Therefore, the correct answer is 3000N.
The mass of the tennis ball is given as 150 grams. We need to convert this to kilograms since the standard unit of mass in physics is kilograms.
Mass=150g=0.150kg
The initial velocity u of the ball is given as 40 m/s (towards the bat), and since it is hit back, we consider this direction as negative. Therefore:
u=−40m/s
The final velocity v after being hit back is 60 m/s (in the opposite direction):
v=60m/s
The change in momentum Δp is given by:
Δp=m(v−u)
Substituting the values:
Δp=0.150kg×(60m/s−(−40m/s))
Δp=0.150kg×(60+40)m/s
Δp=0.150kg×100m/s=15kg m/s
The time duration Δt during which the ball is in contact with the bat is given as 5 milliseconds. We need to convert this to seconds:
Δt=5ms=5×10−3s
The average force F can now be calculated using the formula:
F=ΔpΔt
Substituting the values we have:
F=15kg m/s5×10−3s=3000N
The magnitude of the average force F on the ball is:
F=3000N
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-II
112 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - SWOE
44 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)
4 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
24 Videos
Similar Questions
The following problems illustrate the effect of a time-dependent force of a large average magnitude which acts on a moving object only for a short duration. Such forces are called 'impulsive' forces. According to the impulse-momentum theorem, impulse delivered to a body is equal to the change of linear momentum of the body.
A ball of mass 250g is thrown with a speed of 30m/s. The ball strikes a bat and it is hit straight back along the same line at a speed of 50m/s. Variation of the interaction force, as long as the ball remains in contact with the bat, is as shown in Fig.
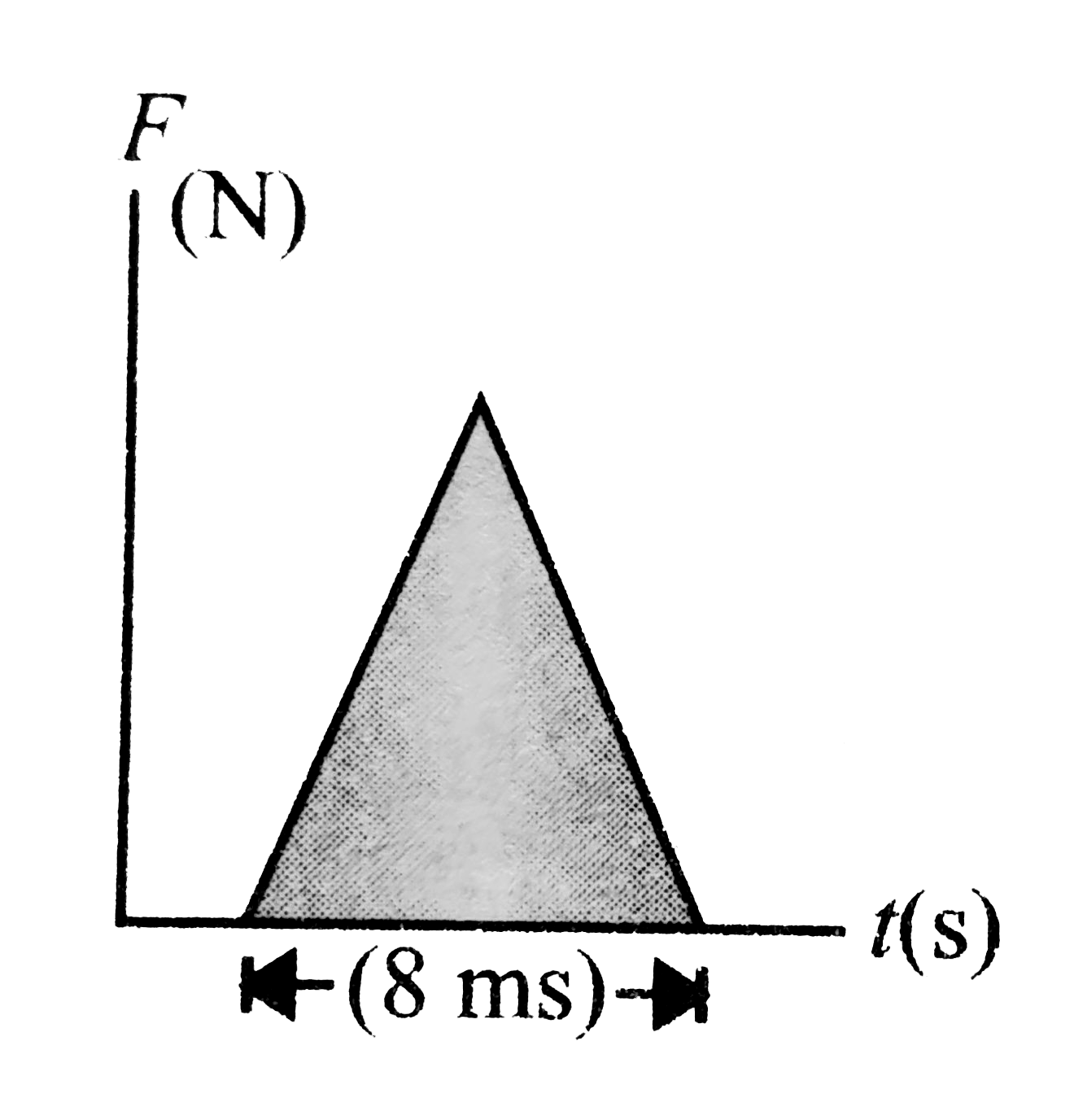
Maximum force exerted by the bat on the ball is
View SolutionFind average speed of 40m/s and 50m/s.
View SolutionA 150 g ball, moving horizontally at 20m/s was hit straight back to bowler at 12m/s. If contact with bat lasted for 0.04 sec, then average force exerted by the bat on the ball is:-
View SolutionA golf ball of mass m is hit by a golf club so that the ball leaves the tee with speed v. The club is in contact with the ball for time T. The magnitude of the average force on the club on the ball during the time T is :
View SolutionA cricket ball of mass 150 g moving at a speed of 25 ms−1 is brought to rest by a player in 0.03 s. Find the average force applied by the player.
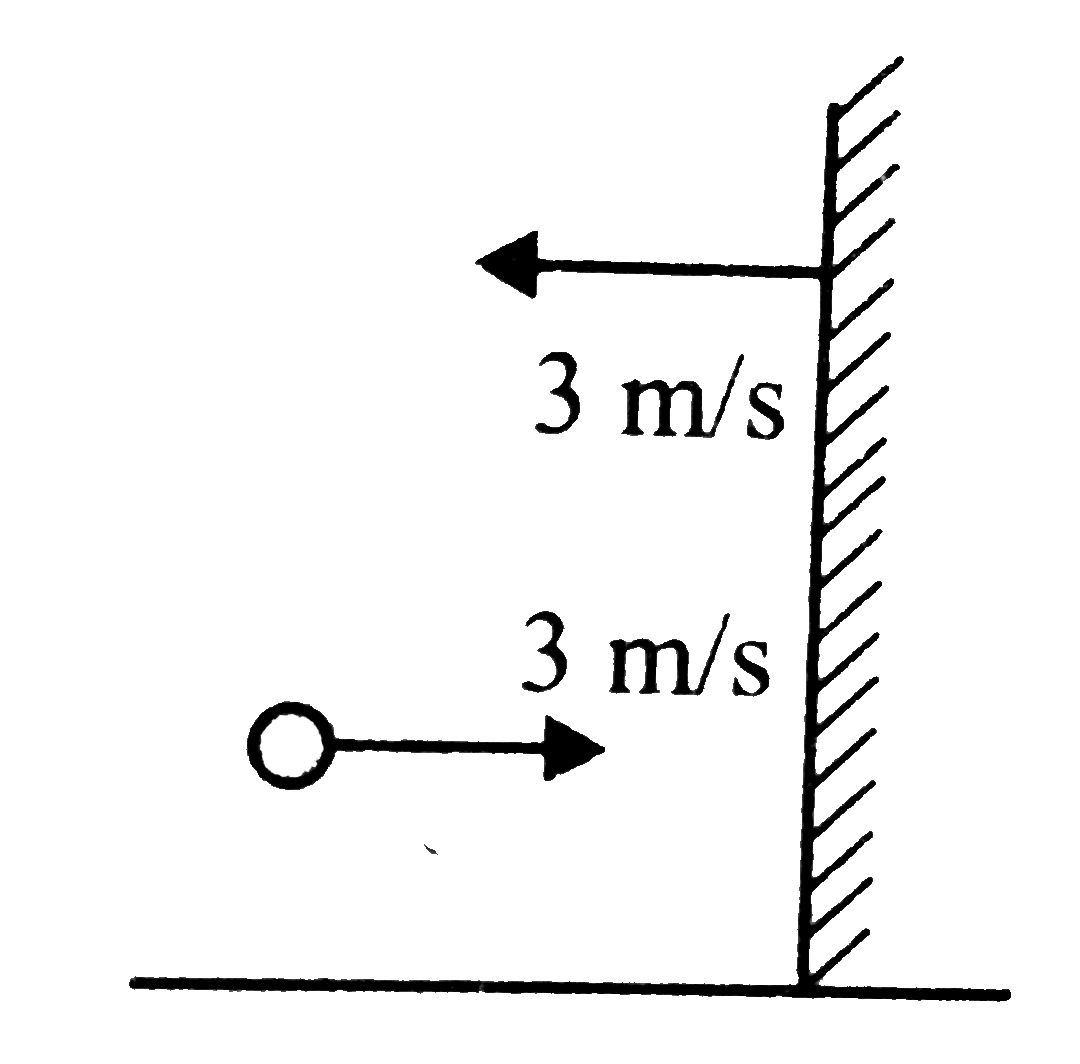
View SolutionA highly elastic ball moving at a speed of 3m/s approaches a wall moving towards it with a speed of 3m/s. After the collision. the speed of the ball will be
View SolutionA man travels 200 km distance with the speed of 40 m/s and next 50 km with the speed of 10 m/s . Find the average speed of man.
View SolutionA ball of mass 0⋅2 kg moving with a speed of 30 m/s strikes a bat returns in opposite direction with speed of 90m/s . Magnitude of change in momentu,m of ball will be
View SolutionA 150 g ball, moving horizontally at 20 m/s was hit straight back to bowler at 35 m/s. If contact with bat lasted for 0.04sec, than average force exerted by the bat on the ball is:

View SolutionA ball is thrown up at a speed of 4.0 m/s. Find the maximum height reached by the ball. Take g=10m/s2.
View Solution
