When unit mass of water boils to become steam at 100∘C, it absorbs Q amount of heat. The densities of water and steam at 100∘C are ρ1 and ρ2 respectively and the atmospheric pressure is P0. The increase in internal energy of the water is
a.Q
b.Q+P0(1ρ1−1ρ2)
c.Q+P0(1ρ2−1ρ1)
d.Q−P0(1ρ1+1ρ2)
The correct Answer is:B
To find the increase in internal energy of water when it boils to become steam at 100∘C, we can use the first law of thermodynamics, which states:
ΔU=ΔQ−W
Where:
- ΔU is the change in internal energy,
- ΔQ is the heat absorbed,
- W is the work done by the system.
Step 1: Identify the heat absorbed
Since it is given that the unit mass of water absorbs Q amount of heat to convert to steam, we can write:
ΔQ=Q
Step 2: Calculate the work done
The work done W during the phase change at constant pressure can be expressed as:
W=P⋅(V2−V1)
Where:
- V2 is the volume of steam,
- V1 is the volume of water.
Step 3: Express volumes in terms of densities
Using the relationship between mass, volume, and density, we can express the volumes as:
V1=mρ1andV2=mρ2
Since we are considering unit mass of water, m=1:
V1=1ρ1andV2=1ρ2
Step 4: Substitute volumes into the work done equation
Now substituting V1 and V2 into the work done equation:
W=P0(1ρ2−1ρ1)
Step 5: Substitute ΔQ and W into the first law equation
Now we can substitute ΔQ and W back into the first law of thermodynamics:
ΔU=Q−P0(1ρ2−1ρ1)
Step 6: Rearranging the equation
This can be rearranged to:
ΔU=Q−P0(1ρ2−1ρ1)
Final Expression
Thus, the increase in internal energy of the water when it boils to steam is:
ΔU=Q+P0(1ρ1−1ρ2)
- ΔU is the change in internal energy,
- ΔQ is the heat absorbed,
- W is the work done by the system.
Since it is given that the unit mass of water absorbs Q amount of heat to convert to steam, we can write:
The work done W during the phase change at constant pressure can be expressed as:
- V2 is the volume of steam,
- V1 is the volume of water.
Using the relationship between mass, volume, and density, we can express the volumes as:
Now substituting V1 and V2 into the work done equation:
Now we can substitute ΔQ and W back into the first law of thermodynamics:
This can be rearranged to:
Thus, the increase in internal energy of the water when it boils to steam is:
Topper's Solved these Questions
- GEOMETRICAL OPTICSBook:ALLENChapter:GEOMETRICAL OPTICSExercise:EXERCISE -03
Explore 10 Videos
- GEOMETRICAL OPTICSBook:ALLENChapter:GEOMETRICAL OPTICSExercise:ASSERTION-REASON
Explore 36 Videos
- GEOMETRICAL OPTICSBook:ALLENChapter:GEOMETRICAL OPTICSExercise:EXERCISE -01
Explore 65 Videos
- CURRENT ELECTRICITYBook:ALLENChapter:CURRENT ELECTRICITYExercise:EX.II
Explore 66 Videos
- GRAVITATIONBook:ALLENChapter:GRAVITATIONExercise:EXERCISE 4
Explore 9 Videos
Similar Questions
Calculate amount of heat required to convert 1kg steam form 100∘C→200∘C steam
View Solution540 calories of heat convert 1 cubic centimeter of water at 100oC into 1671 cubic centimeter of steam at 100oC at a pressure of one atmosphere. Then the work done against the atmospheric pressure is nearly
View Solution
Knowledge Check
The density of water at the surface of ocean is ρ . If the bulk modulus of water is B, then the density of ocean water at depth, when the pressure at a depth is αp0 and p0 is the atmospheric pressure is
AρKk−nP0BρKK+nP0CρKK−(n−1)P0DρK(K+(n−1)P0)
How much work is done on the steam when 1.00 mol of water at 100∘C boils and becomes 1.00 mole of steam at 100∘C at 1.00 atm pressure? Assume the steam to behave as an ideal gas , determine the change in the internal energy of the material as it vapourizes.
View SolutionA sample of 0.1g of water of 100∘C and normal pressure (1.013×105Nm−2) requires 54 cal of heat energy to convert to steam at 100∘C. If the volume of the steam produced is 167.1 cc, the change in internal energy of the sample is
View Solution1 kg of water having a volume of 10−3m3 becomes 1.67m3 of steam when boiled at a pressure of one atmosphere. The heat of vapourization at this pressure is 540 kcal/kg. Calculate the increase in internal energy and the work done in expansion.
View SolutionWrite true or false for the following statements:
Steam at100∘C causes more severe burns than water at 100∘C _________.
View SolutionWater is filled up to a height h in a beaker of radius R as shown in the figure. The density of water is ρ, the surface tension of water is T and the atmospheric presure is p0. Consider a vertical section ABCDof the water on one side of this section by water on the other side of this section has magnitude
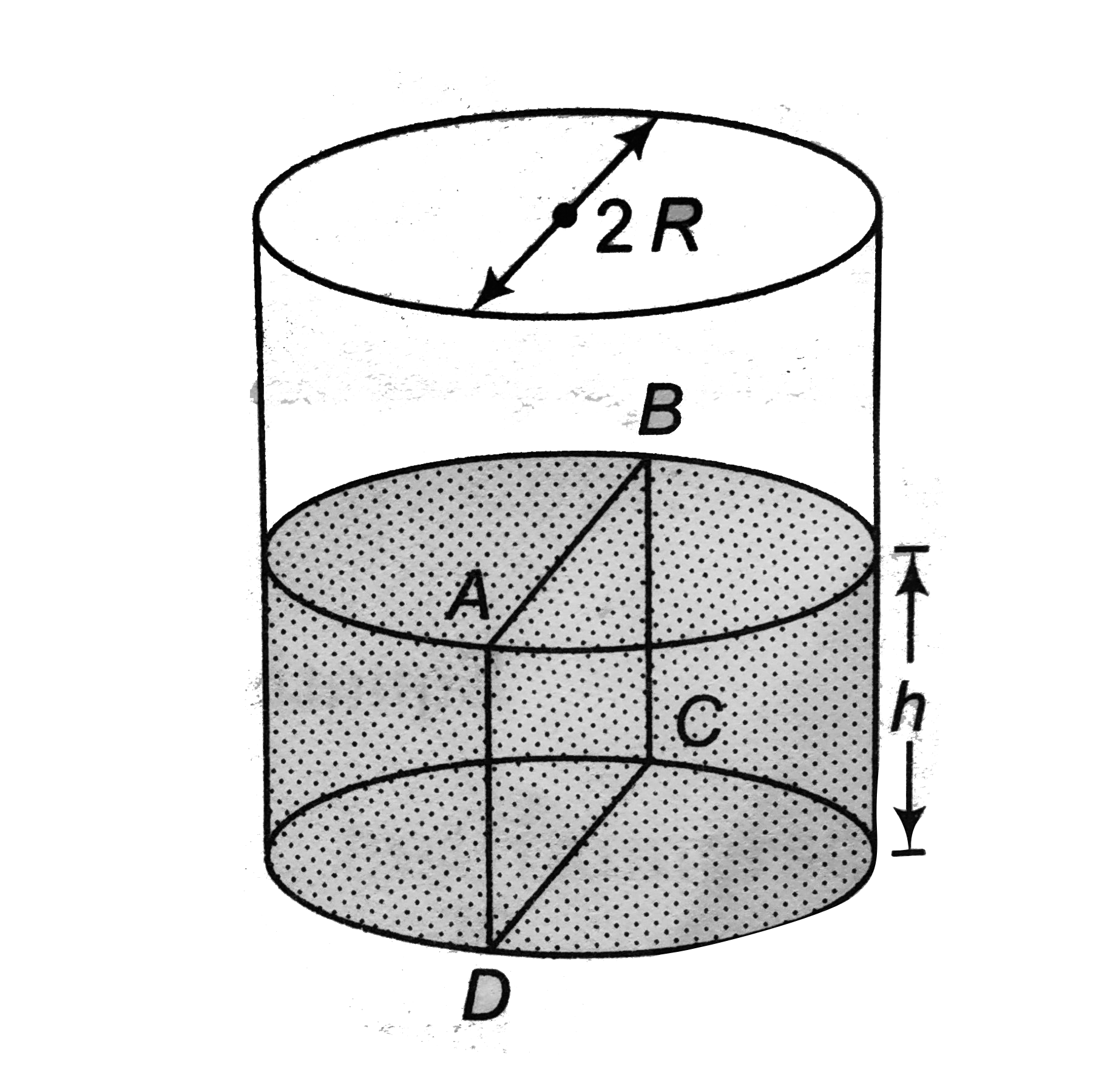
(a) ∣∣2p0Rh+πR2ρgh−2RT∣∣
(b)∣∣2p0Rh+Rρgh2=2RT∣∣
(c ) ∣∣p0(π)R2+Rρgh2−2RT∣∣
(d) ∣∣p0πR2+Rρgh2+2RT∣∣.
View Solution1 g of water at 100∘C is completely converted into steam at 100∘C. 1g of steam occupies a volume of 1650cc. (Neglect the volume of 1g of water at 100∘C). At the pressure of 105N/m2, latent heat of steam is 540 cal/g (1 Calorie=4.2 joules). The increase in the internal energy in joules is
View Solutioncalculate the increase in internal energy of 1 kg of water at 100oC when it is converted into steam at the same temperature and at 1atm (100 kPa). The density of water and steam are 1000 kg m−3 and 0.6 kg m−3 respectively. The latent heat of vaporization of water =2.25×106Jkg1.
View Solution
