The average value of alternating current I=I0sinωt in time interval [0,πω] is
To find the average value of the alternating current I=I0sin(ωt) over the time interval (0,πω), we will follow these steps:
Step 1: Define the average value of the current
The average value Iavg of a function over a time interval (a,b) is given by the formula:
Iavg=1b−a∫baI(t)dt
In our case, a=0 and b=πω.
Step 2: Set up the integral
Substituting the values into the formula, we have:
Iavg=1πω−0∫πω0I0sin(ωt)dt
This simplifies to:
Iavg=ωπ∫πω0I0sin(ωt)dt
Step 3: Factor out constants
We can factor I0 out of the integral:
Iavg=ωI0π∫πω0sin(ωt)dt
Step 4: Evaluate the integral
Now, we need to evaluate the integral ∫πω0sin(ωt)dt. The integral of sin(kx) is:
∫sin(kx)dx=−1kcos(kx)+C
Applying this to our integral:
∫sin(ωt)dt=−1ωcos(ωt)
Now, we evaluate it from 0 to πω:
∫πω0sin(ωt)dt=(−1ωcos(ωt))πω0=−1ω(cos(πω⋅ω)−cos(0))
This simplifies to:
−1ω(cos(π)−cos(0))=−1ω(−1−1)=−1ω⋅(−2)=2ω
Step 5: Substitute back into the average formula
Now substituting this result back into our average value formula:
Iavg=ωI0π⋅2ω=2I0π
Final Answer
Thus, the average value of the alternating current I=I0sin(ωt) in the time interval (0,πω) is:
Iavg=2I0π
The average value Iavg of a function over a time interval (a,b) is given by the formula:
Iavg=1b−a∫baI(t)dt
In our case, a=0 and b=πω.
Substituting the values into the formula, we have:
Iavg=1πω−0∫πω0I0sin(ωt)dt
This simplifies to:
Iavg=ωπ∫πω0I0sin(ωt)dt
We can factor I0 out of the integral:
Iavg=ωI0π∫πω0sin(ωt)dt
Now, we need to evaluate the integral ∫πω0sin(ωt)dt. The integral of sin(kx) is:
∫sin(kx)dx=−1kcos(kx)+C
Applying this to our integral:
∫sin(ωt)dt=−1ωcos(ωt)
Now, we evaluate it from 0 to πω:
∫πω0sin(ωt)dt=(−1ωcos(ωt))πω0=−1ω(cos(πω⋅ω)−cos(0))
This simplifies to:
−1ω(cos(π)−cos(0))=−1ω(−1−1)=−1ω⋅(−2)=2ω
Now substituting this result back into our average value formula:
Iavg=ωI0π⋅2ω=2I0π
Thus, the average value of the alternating current I=I0sin(ωt) in the time interval (0,πω) is:
Iavg=2I0π
Topper's Solved these Questions
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Exersice-03
7 Videos
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - ASSERTION-REASON
11 Videos
- AIIMS 2019BOOK - ALLENCHAPTER - AIIMS 2019EXERCISE - PHYSICS
40 Videos
- CIRCULAR MOTIONBOOK - ALLENCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-A)
6 Videos
Similar Questions
The average value of an alternating voltage V=V0sinωt over a full cycle is
View SolutionThe average value of an alternating voltage V=V0sinωt over a full cycle is
View SolutionThe average value of current i=Imsinωtomt=π2ω to t=3π2ω si how many times of (Im)?
View SolutionThe average value of current i=Imsinωtomt=π2ω to t=3π2ω si how many times of (Im)?
View SolutionFind the rms value of current i=Imsinωt from (i)t=0tot=πω (ii) t=π2ωtot=3π2ω
View SolutionA direct current of 5 amp is superimposed on an alternating current I=10sinωt flowing through a wire. The effective value of the resulting current will be:
View SolutionA direct current of 5 amp is superimposed on an alternating current I=10sinωt flowing through a wire. The effective value of the resulting current will be:
View SolutionFind the average value in the following cases
(i) i=4+3cosωt
(ii) I=5sinωt+2sin2ωt+3sin3ωt
(iv) V=cosωt+3cos2ωt+3cos3ωt+2
View SolutionIf a direct current of value a ampere is superimposed on an alternative current I=b sinωt flowing through a wire, what is the effective value of the resulting current in the circuit?
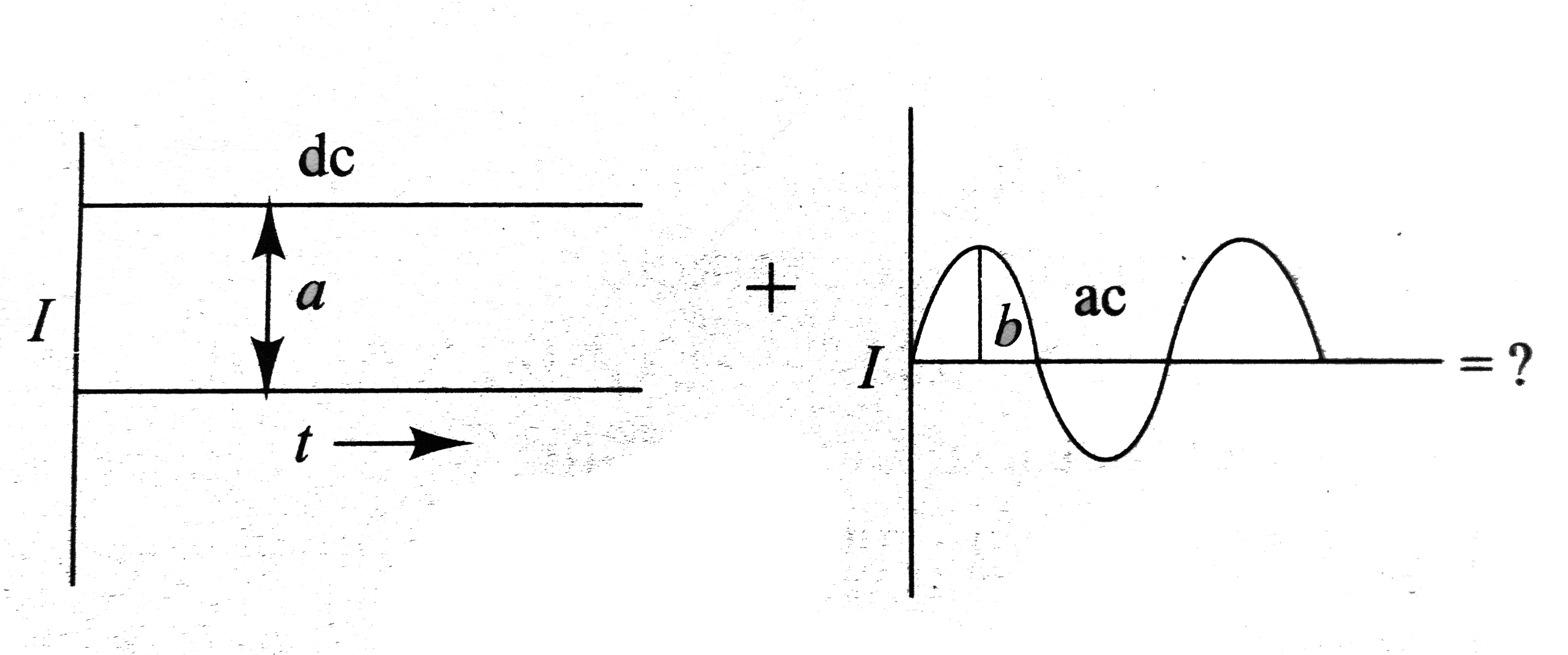
View SolutionThe average value of current for the current shown for time period 0 to T2 is

View Solution
