The average value of an alternating voltage V=V0sinωt over a full cycle is




The correct Answer is:A
To find the average value of the alternating voltage V=V0sin(ωt) over a full cycle, we can follow these steps:
Step 1: Understand the Function
The voltage function given is V(t)=V0sin(ωt), where:
- V0 is the peak voltage,
- ω is the angular frequency,
- t is time.
Step 2: Define the Average Value Over a Full Cycle
The average value Vavg of a function over one complete cycle is defined as:
Vavg=1T∫T0V(t)dt
where T is the period of the function. For a sine function, the period T is given by:
T=2πω
Step 3: Set Up the Integral
Substituting V(t) into the average value formula, we have:
Vavg=1T∫T0V0sin(ωt)dt
Substituting T:
Vavg=ω2π∫2π/ω0V0sin(ωt)dt
Step 4: Evaluate the Integral
Now, we need to evaluate the integral:
∫2π/ω0sin(ωt)dt
Using the substitution u=ωt, du=ωdt, we change the limits accordingly:
- When t=0, u=0
- When t=2πω, u=2π
Thus, the integral becomes:
∫2π0sin(u)duω
Step 5: Solve the Integral
The integral of sin(u) over one complete cycle (from 0 to 2π) is:
∫2π0sin(u)du=0
This is because the area under the curve for sin(u) from 0 to π is positive and from π to 2π is negative, canceling each other out.
Step 6: Calculate the Average Value
Substituting back, we find:
Vavg=V02π⋅0=0
Conclusion
The average value of the alternating voltage V=V0sin(ωt) over a full cycle is:
0
The voltage function given is V(t)=V0sin(ωt), where:
- V0 is the peak voltage,
- ω is the angular frequency,
- t is time.
The average value Vavg of a function over one complete cycle is defined as:
Vavg=1T∫T0V(t)dt
where T is the period of the function. For a sine function, the period T is given by:
T=2πω
Substituting V(t) into the average value formula, we have:
Vavg=1T∫T0V0sin(ωt)dt
Substituting T:
Vavg=ω2π∫2π/ω0V0sin(ωt)dt
Now, we need to evaluate the integral:
∫2π/ω0sin(ωt)dt
Using the substitution u=ωt, du=ωdt, we change the limits accordingly:
- When t=0, u=0
- When t=2πω, u=2π
∫2π0sin(u)duω
The integral of sin(u) over one complete cycle (from 0 to 2π) is:
∫2π0sin(u)du=0
This is because the area under the curve for sin(u) from 0 to π is positive and from π to 2π is negative, canceling each other out.
Substituting back, we find:
Vavg=V02π⋅0=0
The average value of the alternating voltage V=V0sin(ωt) over a full cycle is:
0
Topper's Solved these Questions
Similar Questions
The average value of alternating current I=I0sinωt in time interval [0,πω] is
View SolutionThe peak value of an alternating emf E given by
E=(E0)cosωt
is 10V and frequency is 50 Hz. At time t=(1/600)s the instantaneous value of emf isView SolutionFind the average speed of a particle whose velocity is given by V=V0sinωt t where T=2π/ω is the time of complete cycle
View SolutionThe equation of an alternating voltage is E=220sin(ωt+π/6) and the equation of the current in the circuit is I=10sin(ωt−π/6). Then the impedance of the circuit is
View SolutionThe average value of voltage (V) in one time period will be
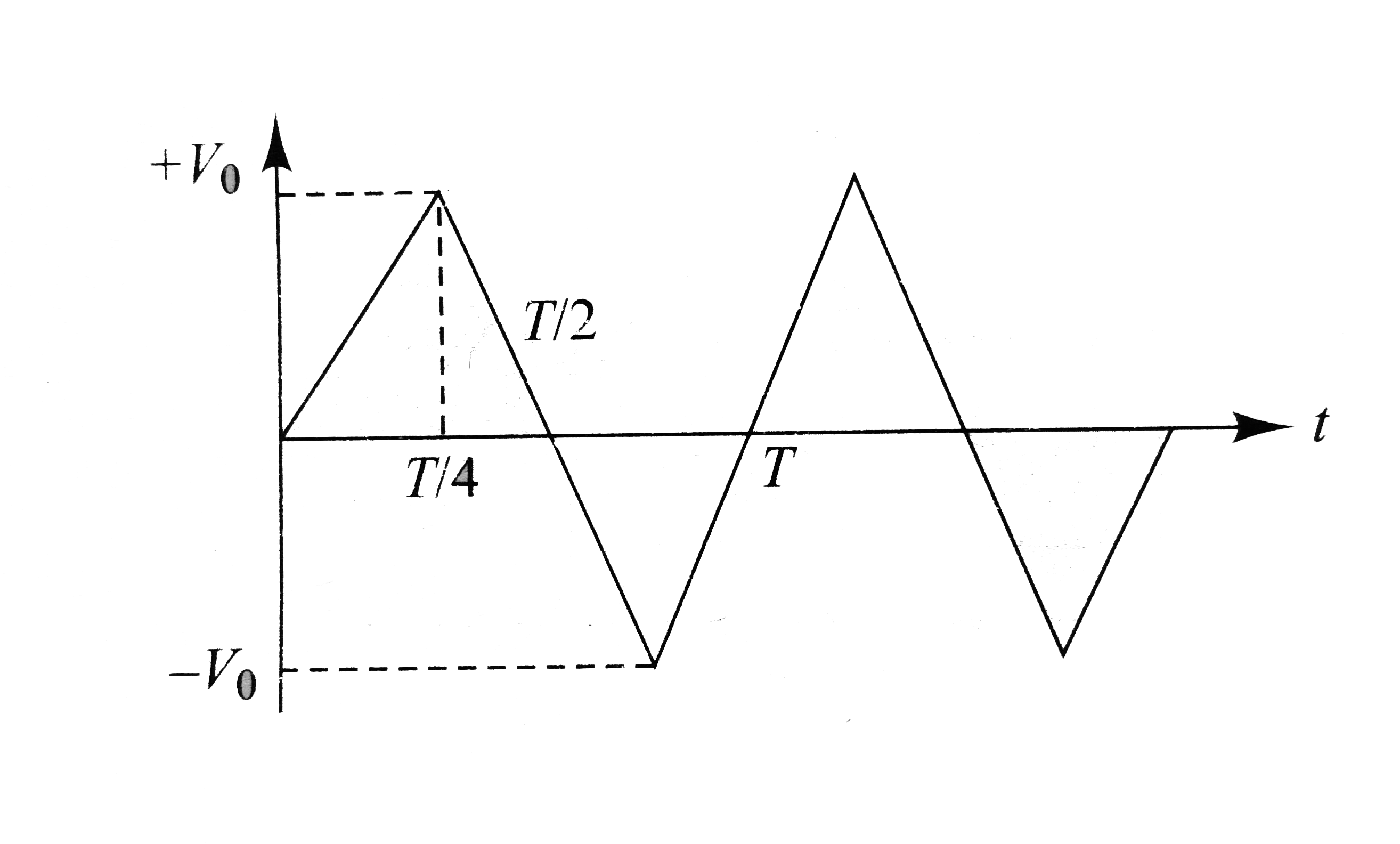 View Solution
View SolutionThe average value of a saw-tooth voltage V=V0(2tT−1) Over 0 to T2, (T- time period) is given by
View SolutionA series R-L circuit is subjected to an alternating voltage given as (v=v0sinωt). Then the variation of peak current (i) with frequency (v) is denoted by
View SolutionA small signal voltage V(t)=V0sinωt is applied across an ideal capacitor C:
View SolutionA coil of inductance 5.0 mH and negligible resistance is connected to an alternating voltage V=10sin(100t). The peak current in the circuit will be:
View SolutionThe voltage over a cycle varies as
V=V0sinωt for 0≤t≤πω
=−V0sinωt for πω≤t≤2πω
The average value of the voltage one cycle isView Solution

