As θ increases from 0∘ to 90∘, the value of cosθ is
Statement-I is true, Statement-II is true, Statement-II is correct explanation for statement-I
Statement-I is true, Statement-II is true, Statement-II is NOT a correct explanation for statement-I
Statement-I is true, Statement-II is false.
Statement-I is false and Statement-II is true.
The correct Answer is:D
To solve the question "As θ increases from 0∘ to 90∘, the value of cosθ is", we will analyze the behavior of the cosine function over the specified interval.
1. Understanding the Cosine Function:
The cosine function, denoted as cos(θ), is a trigonometric function that relates the angle θ to the ratio of the adjacent side to the hypotenuse in a right triangle.
2. Range of Theta:
We are interested in the range of θ from 0degrees to 90degrees.
3. Values of Cosine at Key Angles:
- At θ=0degrees, cos(0)=1.
- At θ=90degrees, cos(90)=0.
4. Graphical Representation:
If we plot the cosine function on a graph:
- The x-axis represents the angle θ (from 0 to 90degrees).
- The y-axis represents the value of cos(θ).
5. Behavior of Cosine Function:
As θ increases from 0degrees to 90degrees, the value of cos(θ) decreases:
- Starting from 1 at 0degrees, it gradually decreases.
- It reaches 0 at 90degrees.
6. Conclusion:
Therefore, as θ increases from 0degrees to 90degrees, the value of cos(θ) decreases from 1 to 0.
Final Answer:
As θ increases from 0∘ to 90∘, the value of cos(θ) decreases from 1 to 0.
---
The cosine function, denoted as cos(θ), is a trigonometric function that relates the angle θ to the ratio of the adjacent side to the hypotenuse in a right triangle.
We are interested in the range of θ from 0degrees to 90degrees.
- At θ=0degrees, cos(0)=1.
- At θ=90degrees, cos(90)=0.
If we plot the cosine function on a graph:
- The x-axis represents the angle θ (from 0 to 90degrees).
- The y-axis represents the value of cos(θ).
As θ increases from 0degrees to 90degrees, the value of cos(θ) decreases:
- Starting from 1 at 0degrees, it gradually decreases.
- It reaches 0 at 90degrees.
Therefore, as θ increases from 0degrees to 90degrees, the value of cos(θ) decreases from 1 to 0.
As θ increases from 0∘ to 90∘, the value of cos(θ) decreases from 1 to 0.
Topper's Solved these Questions
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Comprehension 1
5 Videos
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Comprehension 2
3 Videos
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Exersice-03
7 Videos
- AIIMS 2019BOOK - ALLENCHAPTER - AIIMS 2019EXERCISE - PHYSICS
40 Videos
- CIRCULAR MOTIONBOOK - ALLENCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-A)
6 Videos
Similar Questions
Knowledge Check
As θ increases from π4 to 5π4, the value of 4cos12θ
Aincreases, and then decreasesBdecreases, and then increasesCdecreases throughoutDincreases throughout
A body is placed on a rough inclined plane of inclination θ. As the angle θ is the increased from 0∘ to 90∘, the contact force between the block and the plane

View SolutionIn the odjoining figure, angle of plane θ is increased from 0∘to 90∘. Plot force of friction f veraus θ graph
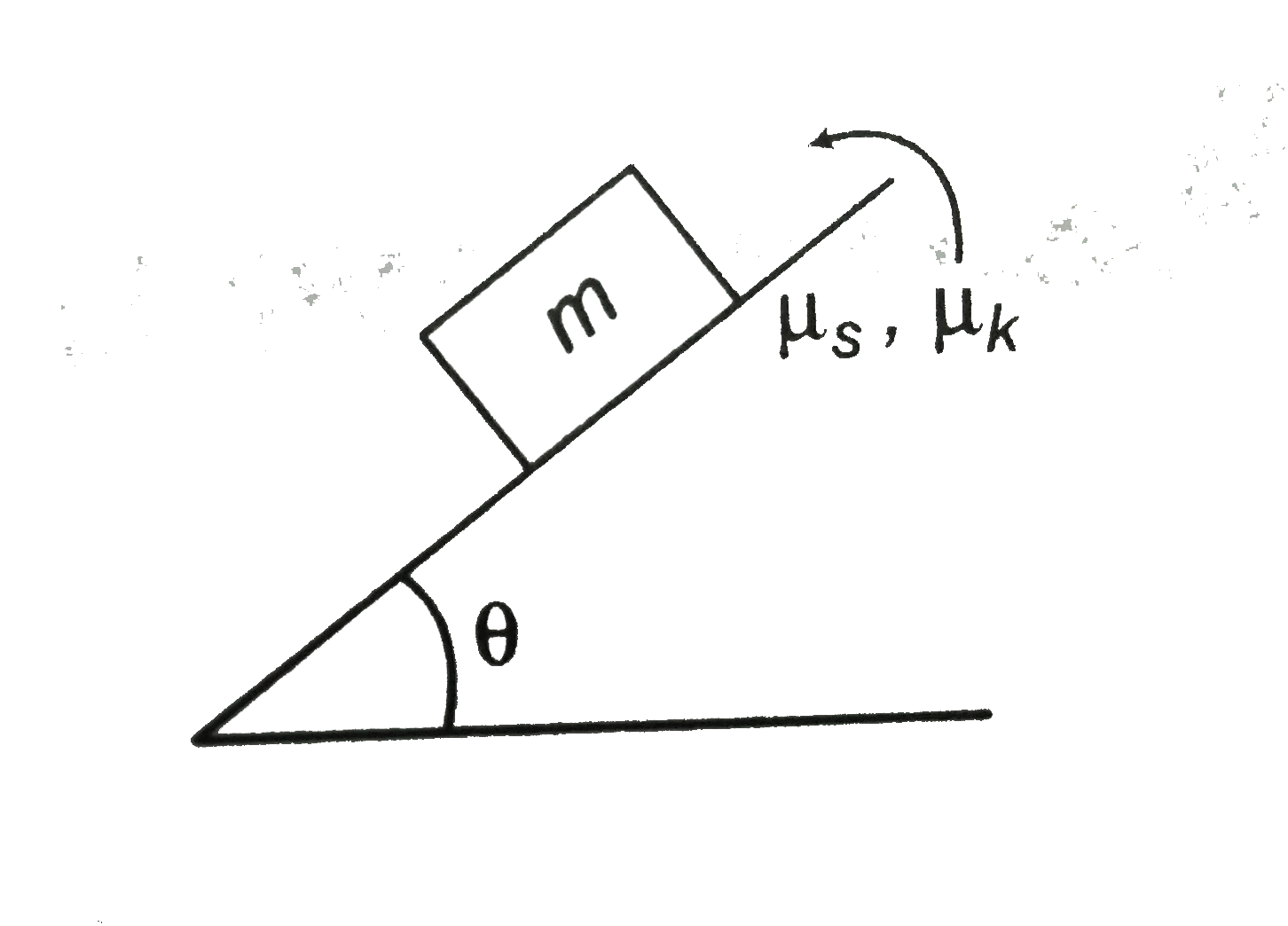
View SolutionIf tanθ=cotθ and 0∘≤θ≤90∘, state the value of θ.
View SolutionIf tanθ=cotθ and 0∘≤θ≤90∘, state the value of θ.
View Solution(i) If secA=cosec A and0∘≤A≤90∘, State the value of A.
(ii) If tanθ=cotθand0∘≤θ≤90∘, State the value of θ.
View SolutionIf 2 cos2θ+sinθ−2=0and0∘≤θ≤90∘ find the value of θ.
View SolutionIf sinθ+cosθ=√2cosθ,(θ≠90∘) then value of tanθ is
View Solution